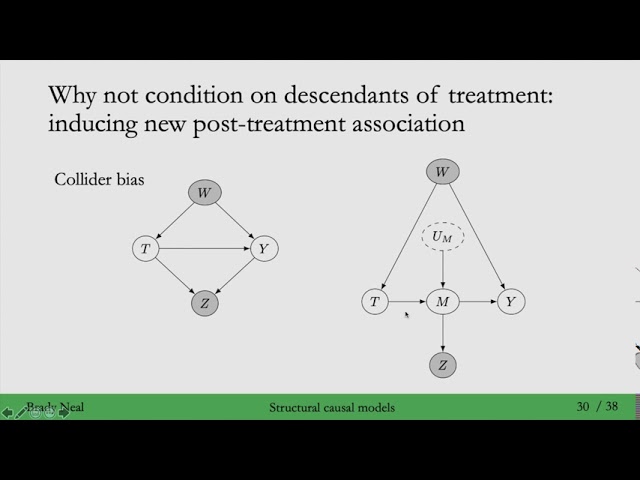
4.9 - M-Bias and Conditioning on Descendants of Treatment

In this part of the Introduction to Causal Inference course, we cover M-bias and what can go wrong if you condition on descendants of treatment. Please post questions in the YouTube comments section.

[sebastienlachapelle]

Thanks again for these clear lectures!
Around 2:33 you mention that M is a collider, but I believe it is false. I would go as far as to say the statement is ill-defined because a collider is defined only with respect to a path. So if you look specifically at the path T -> M -> Y, M is not a collider and it doesn't change anything that M is part of an immorality with T and U_M. See Elements of Causal Inference by Peters et al p.82-83.

A correct justification for why (most) descendants of T cannot be in the adjustment set is presented in page 113-114 of the same book. I have added "most" in parenthesis because some descendants of T are actually allowed (see Proposition 6.41 (iii)).

[lunapopo]

4:11 In case of M-bias, what if Z2 is also a confounding variable? Do we have to block both Z2 AND (Z1 and/or Z3)?

[JP-zzxx]

How do you apply the backdoor adjustment when you have multiple paths from T to Y to get the total causal flow? In your example, you have mediated path via m and a parallel direct path from T to Y. It got me thinking how to apply it when you have N parallel paths. While on the subject, are there methods to collapse parallel paths from T to Y to a single equivalent path?

[JP-zzxx]

define post-treatment and pre-treatment covariates. Pre-treatment covariates seems to be anything allowing association via backdoor paths and post-treatment covariates allows bias on the directed causal path.