filmov
tv
A nice geometry trick!

Показать описание
We use the notion of signed area to solve a geometry problem from a Hungarian contest.
If you are going to use an ad-blocker, considering using brave and tipping me BAT!
Books I like:
Abstract Algebra:
Differential Forms:
Number Theory:
Analysis:
Calculus:
My Filming Equipment:
If you are going to use an ad-blocker, considering using brave and tipping me BAT!
Books I like:
Abstract Algebra:
Differential Forms:
Number Theory:
Analysis:
Calculus:
My Filming Equipment:
A nice geometry trick!
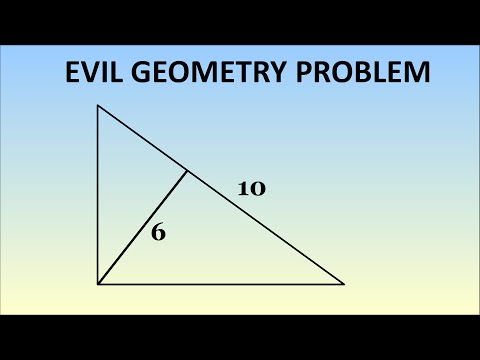
Evil Geometry Problem
Geometry Tips : Math Geometry Tips
Geometry everyone should learn
Fastest Geometry Summary
How to INSTANTLY Improve in Geometry Dash!
Missing Angles Geometry Problem | Tricky Math Question | JusticeTheTutor #maths #math #shorts
10 Geometry Dash Editor Tricks to make you a FASTER Creator!
Square short trick | math magic trick | maths short trick | #maths #learnmath#shortvideo #shortfeed
geometry dash logic
Understand Geometry in 10 min
Geometry Dash 2.2 Keyframe System
GRE Math | Geometry Secret Shortcut
How to make a GOOD level in GEOMETRY DASH ( GD 2.113 )
How To Make A Good Layout! (Geometry Dash 2.11) [FULL GUIDE FOR BEGINNERS] | DeVeReL
How to prepare your Geometry for the IMO and other math competitions
TOP 5 EDITOR TIPS & TRICKS | GEOMETRY DASH
Geometry Trick | Lines and Angles | Maths Trick | Best For All Competitive Exam | imran sir maths
A Beginners Guide To GEOMETRY DASH | How to get started!
Maths Trick By Imran Sir | Geometry Trick | Star Figure Trick | Rectangle Trick
How to Make Less Annoying Gameplay in Geometry Dash
Geometry Dash Animation difficulties #geometrydash #animation #viral
Geometry Dash: Level Editor Tips and Tricks
The ULTIMATE BLOCK DESIGN TUTORIAL! (GD Geometry dash 2.113)
Комментарии
 0:12:30
0:12:30
 0:03:23
0:03:23
 0:02:00
0:02:00
 0:00:15
0:00:15
 0:02:52
0:02:52
 0:00:55
0:00:55
 0:00:37
0:00:37
 0:10:13
0:10:13
 0:00:45
0:00:45
 0:00:57
0:00:57
 0:21:34
0:21:34
 0:00:33
0:00:33
 0:02:34
0:02:34
 0:09:00
0:09:00
 0:16:12
0:16:12
 0:05:42
0:05:42
 0:09:48
0:09:48
 0:21:16
0:21:16
 0:07:32
0:07:32
 0:09:23
0:09:23
 0:08:13
0:08:13
 0:00:31
0:00:31
 0:09:06
0:09:06
 0:09:13
0:09:13