filmov
tv
Integral of sqrt(x^2-1)

Показать описание
In this video, the last one of the integral-trilogy, I find the antiderivative of the square root of x^2 - 1. The method is surprisingly similar to the one of the square root of x^2 + 1, and I recommend you see that one first, because it uses the formula for the integral of sec^3, which I derive in that video. Enjoy!
Integral of sqrt(x^2+1), integral of sqrt(x^2-1), integral of sqrt(1-x^2)
integral of sqrt(x^2+1), with Euler Substitution, math for fun
Integral of sqrt(x^2-1)
Integral sqrt(x^2+1)
a hard integral of sqrt(x^2-1), trig substitution, calculus 2 tutorial
Integral of sqrt(x^2-1)/x (substitution)
integral of sqrt(1-x^2), trig substitution, calculus 2 tutorial
integral of sqrt(1+x^2), trig substitution, calculus 2 tutorial
Calculus 2 Exam Review Part6
Trigonometric substitution for a definite integral sqrt(x^2-1)/x^2 on [1,2].
Integral of x*sqrt(1-x^2) vs. integral of sqrt(1-x^2), u-substitution vs. integration by parts
Integral sqrt(1-x^2)
Integral of sqrt(1-x^2)
Weird u-substitution for the integral of sqrt(1+x^2)
hyperbolic substitution, integral of sqrt(1+x^2)
integrate sqrt(x+1) over sqrt(x+2)
Find Antiderivative of integral x sqrt(x^2 +1) dx using variable substitution
How to integrate 1/(2 sqrt x)
integral of sqrt(1+x^2)/x vs integral of x/sqrt(1+x^2)
How to integrate 1/sqrt(x^2+1)
Integral of sqrt(x^2-1)/x^4
integral of 1/(x^2*sqrt(x^2-1), trig substitution, calculus 2 tutorial.
Integral of sqrt(1-2x)
Let me quickly integrate x/sqrt(1-x^2)
Комментарии
 0:17:27
0:17:27
 0:20:42
0:20:42
 0:06:27
0:06:27
 0:10:22
0:10:22
 0:02:56
0:02:56
 0:03:02
0:03:02
 0:03:41
0:03:41
 0:02:36
0:02:36
 5:47:36
5:47:36
 0:07:12
0:07:12
 0:09:15
0:09:15
 0:11:01
0:11:01
 0:03:45
0:03:45
 0:01:21
0:01:21
 0:08:11
0:08:11
 0:15:00
0:15:00
 0:03:49
0:03:49
 0:01:38
0:01:38
 0:11:51
0:11:51
 0:02:50
0:02:50
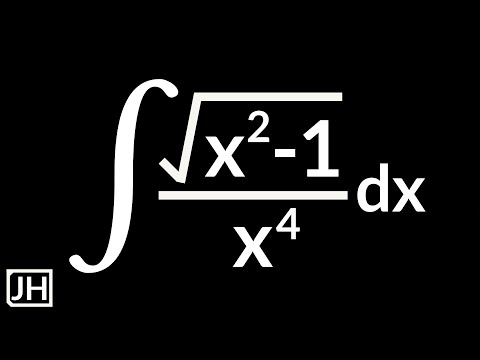 0:06:50
0:06:50
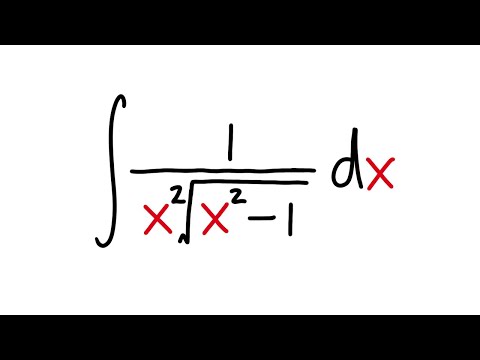 0:04:23
0:04:23
 0:01:40
0:01:40
 0:01:01
0:01:01