filmov
tv
Real Analysis | Subsequences

Показать описание
We introduce the notion of a subsequence and prove a few simple results including the Bolzano-Weirstrass Theorem.
If you are going to use an ad-blocker, considering using brave and tipping me BAT!
Books I like:
Abstract Algebra:
Differential Forms:
Number Theory:
Analysis:
Calculus:
My Filming Equipment:
If you are going to use an ad-blocker, considering using brave and tipping me BAT!
Books I like:
Abstract Algebra:
Differential Forms:
Number Theory:
Analysis:
Calculus:
My Filming Equipment:
Intro to Subsequences | Real Analysis
Real Analysis | Subsequences
Real Analysis 9 | Subsequences and Accumulation Values
Sequence Converges iff Every Subsequences Converge to the Same Limit | Real Analysis
An Important Fact about Subsequences | Real Analysis
Monotone Subsequence Theorem (Every Sequence has Monotone Subsequence) | Real Analysis
Subsequence of a Sequence | Complimentary Pair of Subsequences | Sequence of real numbers : 03
If Sequence Diverges to Infinity then so do Subsequences | Real Analysis
Subsequences of a Sequence | Real Analysis | MA CLASSES
Subsequences Introduction [Real Analysis]
Intro Real Analysis, Lec 8: Subsequences, Bolzano-Weierstrass, Cauchy Criterion, Limsup & Liminf
Sequence (1^n) Diverges using Subsequences | Real Analysis
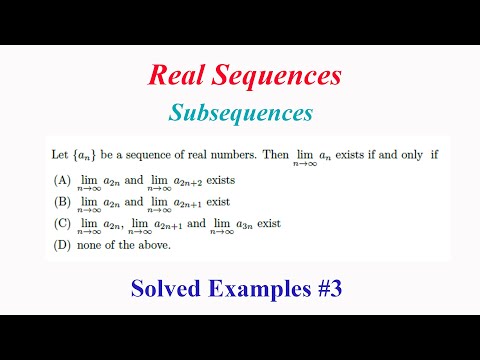
Sequences (Real Analysis) | Subsequences | SE #3 | Sequence {an} exists iff a2n, a2n+1 & a3n exi...
#9 Real Analysis | Intro to Subsequences | Hunter College
Subsequences & The Bolzano-Weierstrass Theorem - Real Analysis I (full course) - lecture 7b (of ...
Real Analysis | Cauchy Sequences
What is a Subsequence?
Subsequence Theorem Introduction [Real Analysis]
Short Proof of Bolzano-Weierstrass Theorem for Sequences | Real Analysis
Find two sequences that are subsequences of each other | Real Analysis
Monotone subsequence
Real Analysis Ep 12: Subsequences & Bolzano Weierstrauss
Real Analysis 9 | Subsequences and Accumulation Values [dark version]
Subsequences & The Bolzano-Weierstrass Theorem - Real Analysis I (full course) - lecture 7a (of ...
Комментарии
 0:15:25
0:15:25
 0:22:16
0:22:16
 0:08:18
0:08:18
 0:08:54
0:08:54
 0:09:00
0:09:00
 0:09:14
0:09:14
 0:17:11
0:17:11
 0:06:56
0:06:56
 0:20:49
0:20:49
 0:12:00
0:12:00
 0:57:23
0:57:23
 0:07:10
0:07:10
 0:03:27
0:03:27
 0:22:13
0:22:13
 0:32:57
0:32:57
 0:19:15
0:19:15
 0:14:59
0:14:59
 0:15:29
0:15:29
 0:05:21
0:05:21
 0:08:29
0:08:29
 0:08:14
0:08:14
 0:33:45
0:33:45
 0:08:05
0:08:05
 0:54:16
0:54:16