filmov
tv
Sequence (1^n) Diverges using Subsequences | Real Analysis

Показать описание
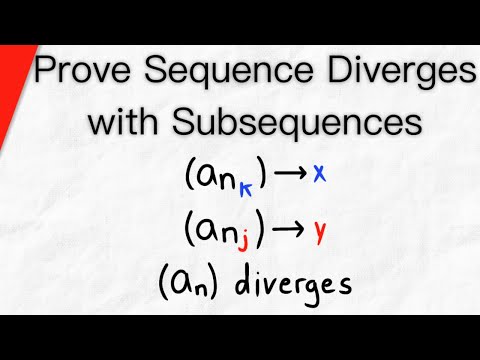
We prove the sequence (-1)^n diverges by finding two subsequences of (-1)^n that converge to different limits. We previously proved (-1)^n diverges by using a contradiction argument, assuming it does converge to some real number L and showing an absurdity. However, now that we have proven a sequence converges to L if and only if all of its subsequences converge to L, we have a much quicker way of proving divergence for oscillating sequences! #RealAnalysis
Note that this technique does not work for divergent sequences that aren't oscillating. If a sequence diverges to +/-infinity, all of its subsequences do as well, and so it will not be possible to find two subsequences with different limits.
★DONATE★
Thanks to Robert Rennie, Barbara Sharrock, and Rolf Waefler for their generous support on Patreon!
Follow Wrath of Math on...
Note that this technique does not work for divergent sequences that aren't oscillating. If a sequence diverges to +/-infinity, all of its subsequences do as well, and so it will not be possible to find two subsequences with different limits.
★DONATE★
Thanks to Robert Rennie, Barbara Sharrock, and Rolf Waefler for their generous support on Patreon!
Follow Wrath of Math on...
Sequence (1^n) Diverges using Subsequences | Real Analysis
Proof: Sequence (-1)^n Diverges | Real Analysis
Prove Sequence Diverges with Subsequences | Real Analysis
If Sequence Diverges to Infinity then so do Subsequences | Real Analysis
Sequence Converges iff Every Subsequences Converge to the Same Limit | Real Analysis
Determining whether a sequence converges or diverges
Sequence (-1)^n Converges or Diverges? Proof |Mad Teacher
Intro to Subsequences | Real Analysis
Sequences (Real Analysis) | Lecture 6 | Subsequences | Introduction & examples an=(-1)^n(1+1/n^...
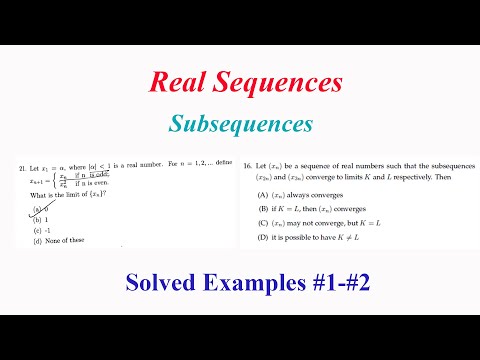
Sequences (Real Analysis) | Subsequences | SE 1-2 | Subsequences x2n and x3n converges to K and L
Proof: Sequence (1/n) is a Cauchy Sequence | Real Analysis Exercises
Subsequences and Divergence
Lecture 6.1 - Subsequences
(RA08) Subsequences of Sequences
Subsequences || Part 8 || Sequences and their Convergence
⛓Sequence Convergence Divergence solved ! ! ! ! !
Tail and Subsequence Convergence
Convergence of Sequences
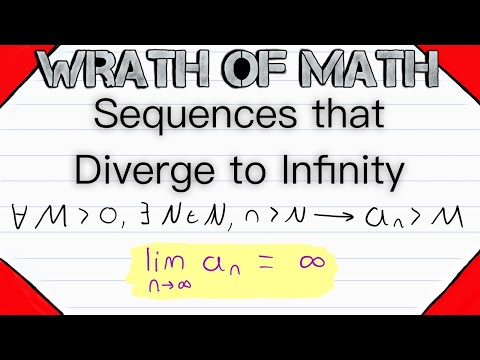
Sequences that Diverge to Infinity (Definition) | Calculus, Real Analysis
Subsequences
An Important Fact about Subsequences | Real Analysis
Lecture 6.2 - More examples; Subsequences of Convergent Sequences
Sequence Convergence Divergence
Monotone Sequence with Convergent Subsequence Converges | Real Analysis
Комментарии
 0:07:10
0:07:10
 0:13:07
0:13:07
 0:07:41
0:07:41
 0:06:56
0:06:56
 0:08:54
0:08:54
 0:05:39
0:05:39
 0:04:20
0:04:20
 0:15:25
0:15:25
 0:04:44
0:04:44
 0:04:00
0:04:00
 0:08:16
0:08:16
 0:10:53
0:10:53
 0:16:00
0:16:00
 0:39:14
0:39:14
 0:27:02
0:27:02
 0:09:08
0:09:08
 0:13:35
0:13:35
 0:23:51
0:23:51
 0:09:59
0:09:59
 0:15:51
0:15:51
 0:09:00
0:09:00
 0:16:37
0:16:37
 0:10:12
0:10:12
 0:09:49
0:09:49