Finding Increasing/Decreasing Intervals – Function Has Rational (Fractional) Exponents

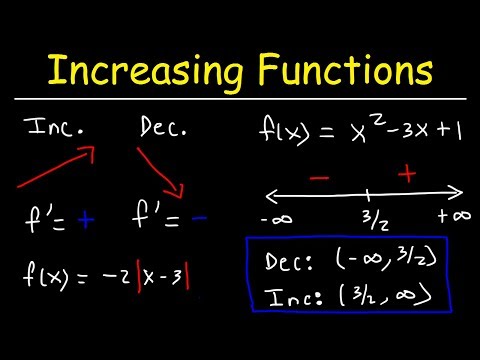
This video shows how to find where a function involving rational exponents increases and decreases. The function in this case is f(x)= x^(2/3)(x^2-4). Once we take the derivative, we use some fancy algebra to simplify the resulting expression, including factoring out the term with the most negative exponent. The steps to find where the function is increasing and decreasing are:
1. Compute f'
2. Use the critical points to define regions
3. Check each region for f' greater than 0 and f' less than 0