filmov
tv
Trig sub integral of x^3*sqrt(R^2-x^2) on zero to R. Let x=Rsin(theta) and transform the limits.

Показать описание
In this trigonometric substitution integral, we integrate x^3*sqrt(R^2-x^2) on zero to R, where R is a constant. We can tell this is a trig sub integral because of the form R^2-x^2 in the square root.
We let x=Rsin(theta) with the intent of using the pythagorean identity 1-sin^2(theta)=cos^2(theta) inside the square root. We also transform the differential dx=Rcos(theta)d(theta) and replace x^3 with (Rsin(theta))^3 in the integrand. Finally, we transform the limits of integration to theta-space.
After simplifying the trig substitution integral, we are left with a classic trigonometric integral of sin^3(theta)*cos^2(theta). When we integrate and odd power of sine next to a power of cosine, we can split off a factor of sin(theta) and transform the rest of the integrand to powers of cosine. The sin(theta) provides the correct differential to take care of using the chain rule backwards, and our antiderivative contains only powers of the cosine function.
Evaluating across the limits of integration, the upper limit gives us zero because the cosine of pi/2 is zero, and the lower limit gives us 2/15, as the cosine evaluates to 1 at theta =0. So the result of the trig sub integral of x^3*sqrt(R^2-x^2) on zero to R is 2/15*R^5!
We let x=Rsin(theta) with the intent of using the pythagorean identity 1-sin^2(theta)=cos^2(theta) inside the square root. We also transform the differential dx=Rcos(theta)d(theta) and replace x^3 with (Rsin(theta))^3 in the integrand. Finally, we transform the limits of integration to theta-space.
After simplifying the trig substitution integral, we are left with a classic trigonometric integral of sin^3(theta)*cos^2(theta). When we integrate and odd power of sine next to a power of cosine, we can split off a factor of sin(theta) and transform the rest of the integrand to powers of cosine. The sin(theta) provides the correct differential to take care of using the chain rule backwards, and our antiderivative contains only powers of the cosine function.
Evaluating across the limits of integration, the upper limit gives us zero because the cosine of pi/2 is zero, and the lower limit gives us 2/15, as the cosine evaluates to 1 at theta =0. So the result of the trig sub integral of x^3*sqrt(R^2-x^2) on zero to R is 2/15*R^5!
Комментарии
 0:06:29
0:06:29
 0:08:52
0:08:52
 0:03:34
0:03:34
 0:15:55
0:15:55
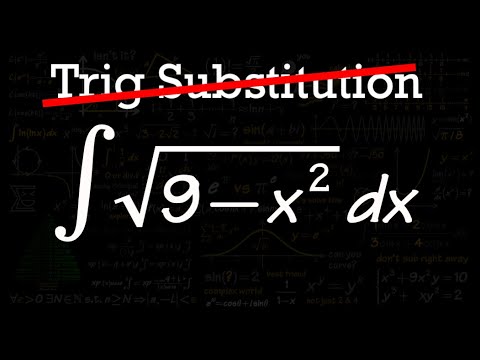 0:06:55
0:06:55
 0:00:43
0:00:43
 0:04:24
0:04:24
 0:00:35
0:00:35
 0:00:37
0:00:37
 0:03:41
0:03:41
 0:00:50
0:00:50
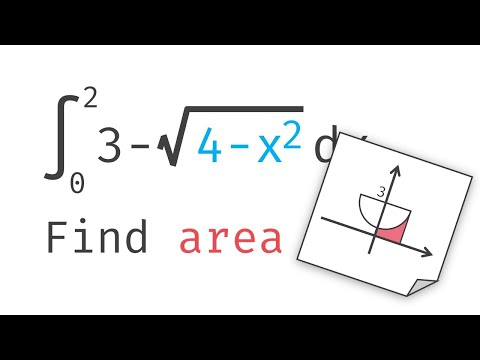 0:08:55
0:08:55
 0:05:40
0:05:40
 0:04:45
0:04:45
 0:10:32
0:10:32
 0:22:40
0:22:40
 0:48:59
0:48:59
 0:38:08
0:38:08
 0:06:07
0:06:07
 0:11:16
0:11:16
 0:02:45
0:02:45
 0:03:02
0:03:02
 0:01:01
0:01:01
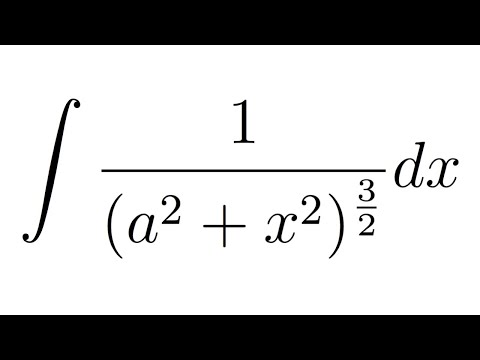 0:05:24
0:05:24