Ch 10: What's the commutator and the uncertainty principle? | Maths of Quantum Mechanics

Hello!

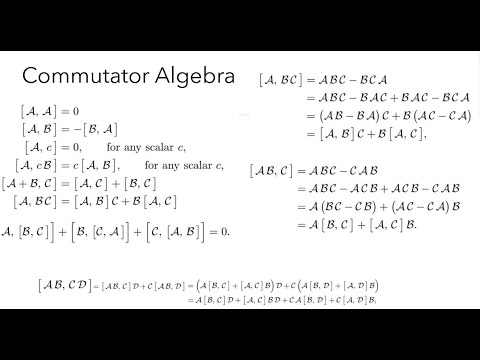
This is the tenth chapter in my series "Maths of Quantum Mechanics." In this episode, we'll define the commutator, and we'll derive how commuting observables share a simultaneous eigenbasis. We'll then dive into how non-commutation necessarily leads to uncertainty relations in quantum mechanics.

If you have any questions or comments, shoot me an email at:
Thanks!

Animations:
All animations created by me within Python, using Manim. To learn more about Manim and to support the community, visit here:

Music:
--------------------------------------------------------------
♪ Astral 1 by Patricia Taxxon
--------------------------------------------------------------

[quantumsensechannel]

Hi everyone, a point of clarification:

At 13:53 when I say “constant times the identity” I mean that we have “i * hbar * Identity”, where the identity operator isn’t shown (since no one ever shows it, but it’s there!). Since we have operators on the left, we need an operator on the right, so that’s why the identity is there. Sorry if this caused some confusion!

-QuantumSense

[benedettosecco]

Concreteness in speeches, expository clarity, very clear voice for non-native english speakers, excellent video editing and superb quality images. This channel deserves a big round of applause. Thanks, thanks, thanks

[ibrahimzaghw]

I just have to say how big of a fan I am of all the hard work you are putting into this. Beautifully explained, well-illustrated, and logically-sequenced. Thanks a million.

[vimiphysics]

Hey creator it's been a long time you didn't uploaded any such videos like this...your content is appreciable ...pls keep posting..

[stevenschilizzi]

This video was absolutely ILLUMINATING, just as the one on observables as linear operators was (where I finally understood how central the property of an operator’s linearity is). Textbooks and other introductory material could well take your exposition as a model to emulate - perhaps even rewriting them accordingly. If only all physics teachers had as clear and meaningful a way to explain things perhaps Feynman’s famous (apocryphal?) quip might be proven wrong, or at least exaggerated, that “Anyone who thinks they understand QM clearly doesn’t understand it”. - Though this of course begs the question: What does it mean “to understand” something? (There are different types of “understanding”! There’s an excellent Mindscape podcast by Sean Carroll on this).

[kgblankinship]

A sign of understanding of a subject and intelligence is the clarity and simplicity of how one presents ideas. Quantum Sense's video presentation here is brilliant!

[khiemgom]

That recap on the proof made u better than 80% of physic/maths explainer out there. Its like they dont see how lengthy their explaination, even though intuitive, are.

[HilbertXVI]

That was a really nice explanation, keep these coming!
Also at 7:10, this is the key fact used to prove the spectral theorem for self adjoint operators in finite dimensions!

[suhanisoni]

dude, you are legit lifesaver. I couldn't attend my university lectures on quantum mechanics because I fell ill and this has literally saved my life. Thank you so much, I am literally going to cry. Best channel ever!

[jaylenchiang]

Why must the eigenvectors of operator B span the eigenspace of A at 9:05 ? Am I missing something from a past video?

[strawberry_cake]

8:46 after thinking about this a while I have one question... Since the parallel and orthogonal eigenvectors share the same eigen value that would mean that the parallel and orthogonal eigenvectors should lie in the same degenerate eigenspace i.e. that would imply that the orthogonal eigenvector should lie in the degenerate eigenspace to which it should be orthogonal to which is contradiction i.e. the orthogonal vector is a 0 vector...

Is this why you state the eigenvectors of B must lie inside or orthogonal to the degenerate eigenspace? if so it wasn't clear

[lucamattioni]

Hey, as I said before, your videos are really mind opening. It would be awsome, for me, if I may suggest, if at the end of this series, will be included one or more videos showing typical applications in problems of some of the explained topics!

[quantumeveryone]

Thanks for another great video. I just wanted to point out a common misconception that you repeated in your summary. Namely that one cannot measure position and momentum at the same time. The simple follow-up question is “so, how do we measure momentum?”, because the detector is always located somewhere and seems like it must de facto measure both. Indeed, the most common way to measure momentum is via a time-of-flight experiment. Here, the quantum particle is trapped in a region of small size and at t=0, the trap is released. Then the particle is allowed to expand in free space until detected. We measure this time of flight along with the particle being detected at a specific position. Then we can determine the momentum (from distance travelled and time travelled and the mass). Uncertainty only enters when the experiment is repeated, because you get a different answer and ultimately determine the momentum probability distribution of the original quantum state. Cindy Regal’s group has recently used this method to image the harmonic oscillator Fock states in momentum space with time-of-flight of atoms. Other applications include the momentum microscope used in XFELs. So, it actually is very common to measure position and momentum at the same time. Proper interpretation of the uncertainty principle requires care.

[sarveshpadav]

if A and B are two observables that commute then we know for sure that all eigenvectors of A are also eigenvectors of B. But if they do not commute then by using the commutation relation, is there a way to know the no of eigenvectors common to both A and B?

[artifinch]

I took a semester of introductory QM and none of it made sense until watching this series. I don't comment much, but this video on particular is really phenomenal :) thank you so much for making this, it's the first engaging, coherent explanation of QM that I've seen

[heack]

There is a more proper title for this series: Master Quantum Machanics in One Day

[HansPeterSloot]

Could you do the proof with non commuting observables?
Would be very enlightening for me.
Regards Hans

[OnlyOnePlaylist]

00:00 : introduction commutators and uncertainty relationsa
00:45 : Motivation for commutation of operators
01:35: Definition of the commutator
02:35 : Motivation and importance of the commutator operator of operators in terms of eigenvectors
04:20 : Motivation and importance of the commutator operator of operators in terms of eigenvectors: nondegenerate case
04:57 : Motivation and importance of the commutator operator of operators in terms of eigenvectors: degenerate case
09:40: Motivation and importance of the commutator operator of observables in terms of eigenvectors: degenerate case recap
10:40: Consequences for observable operators: simultaneous diagonalization
11:40: Importance in physics: commutating vs noncommutating observables
13:40: Importance in physics: noncommutating observables
14:40 : Importance in physics: Heisenberg uncertainty principle
15:36 : Importance in physics: Generalized uncertainty principle and other uncertainty principles
16:30 Recap about commutation of observables
17:00 : Next video

[zlatanbrekke]

Around 8:50 You say that: «we can take the eigenvectors of B to lie either inside the eigenspace of A, or orrhogonal to it - there is no in between». I think that for this to be considered a proof, this statement needs further justificatio. Hopefully you could clarify

[aafeer]

This really connects the formal math and the intuition in a beautiful way. Well structured, excellent execution.