filmov
tv
Solving x^sqrt(x)=1/2, an Exponential Equation

Показать описание
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #ExponentialEquations
PLAYLISTS 🎵 :
#ChallengingMathProblems #ExponentialEquations
PLAYLISTS 🎵 :
Solving x^sqrt(x)=1/2, an Exponential Equation
Solving x^(1/x)=sqrt(2), a Homemade Exponential Equation
Exponential Equations Problem - sqrt(16^x) = (1/2)^(x^2 - 1)
Logarithmic Equation Solving - 2log(sqrt(x-1) - 2) = 0
2 different exponential equations
5 simple unsolvable equations
4^x = 1/sqrt(2) solve the exponential equation
Memorization Trick for Graphing Functions Part 1 | Algebra Math Hack #shorts #math #school
√8^x =16 | Nice Exponent Math Simplification
Can you solve this? | Exponential Equation | Algebra Problem.
Exponential Equations - Algebra and Precalculus
Why is sqrt(x) = x^(1/2)? What Ms. Michael didn't show you #SHORTS
Olympiad Exam | x sqrt 2/x-1 = (x-1)^x-2 | Solve for x | Nice Exponential Equation
Solving sqrt(1+sqrt(x)) = x-1in Two Ways
Solving Exponential Equation
When mathematicians get bored (ep1)
Solving A Beautiful Exponential Equation | sqrt(2x+9)–sqrt(x+1)=sqrt(x+4)
An Exponential Equation | (x^2-x)^x=1
How do you solve an exponential equation with e as the base
Solve (x-1)^⅔ = 4
Learn How to Solve Exponential Equations Using Two Different Methods | Step-by-Step Tutorial
Exponential equation | Find X in 2^(x-1) + 2^(x+1)=1280 algebraic problem| Algebra | Mathematics
A Nice Olympiad Exponential Multiplication Problem #short #olympiad #mathematics #maths #exponents
Why does 'x to the half power' mean square root?
Комментарии
 0:09:57
0:09:57
 0:09:05
0:09:05
 0:03:08
0:03:08
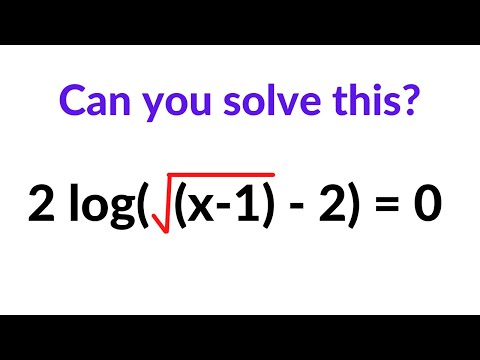 0:01:17
0:01:17
 0:09:19
0:09:19
 0:00:50
0:00:50
 0:00:47
0:00:47
 0:00:15
0:00:15
 0:01:22
0:01:22
 0:03:33
0:03:33
 0:05:58
0:05:58
 0:00:47
0:00:47
 0:09:17
0:09:17
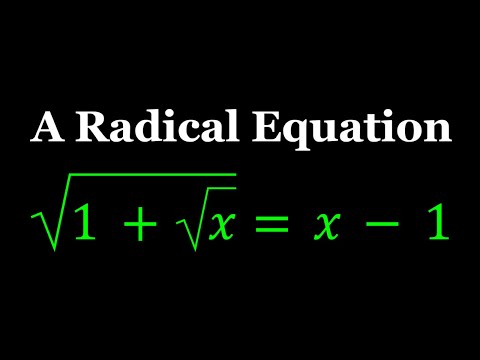 0:11:29
0:11:29
 0:00:56
0:00:56
 0:00:37
0:00:37
 0:09:00
0:09:00
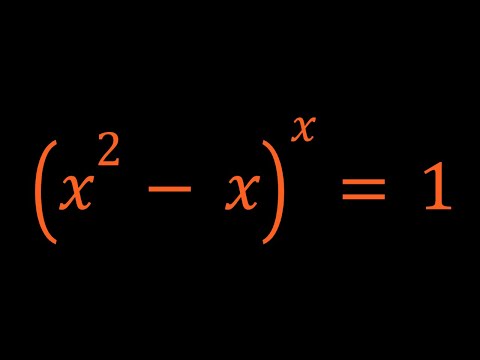 0:06:34
0:06:34
 0:02:47
0:02:47
 0:05:26
0:05:26
 0:12:15
0:12:15
 0:04:28
0:04:28
 0:00:52
0:00:52
 0:02:55
0:02:55