filmov
tv
Physics 36 Electric Field (10 of 18) Horizontal Line Charge
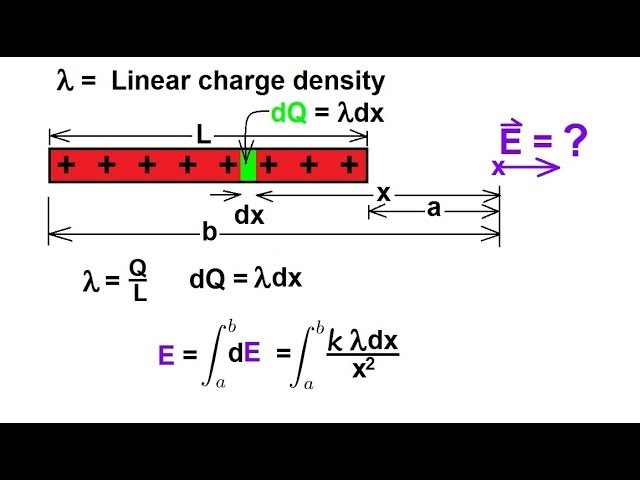
Показать описание
In this video I will find the electric field of a horizontal line charge.
Physics 36 Electric Field (10 of 18) Horizontal Line Charge
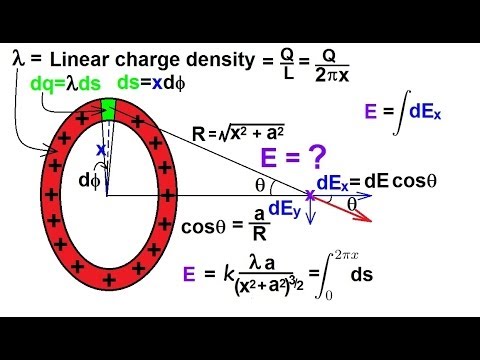
Physics 36 The Electric Field (8 of 18) Ring of Charge
Electric Field Due To Point Charges - Physics Problems
Physics 36 Electric Field (11 of 18) An Arc of Charge
Physics 36 The Electric Field (5 of 18) The Electric Dipole
Physics 36 The Electric Field (9 of 18) Disc of Charge
Physics 36 Electric Field (15 of 18) The Electric Dipole
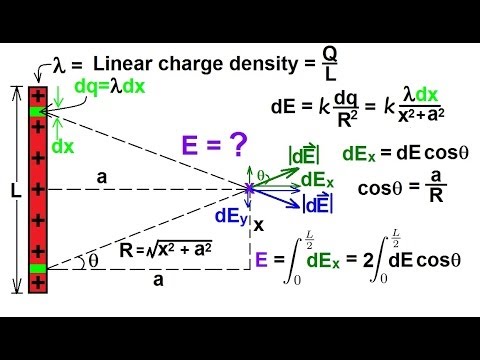
Physics 36 The Electric Field (7 of 18) Finite Length Line Charge
Class X | Science | Electricity MCQs | Part 1
Physics 36 The Electric Field (1 of 18)
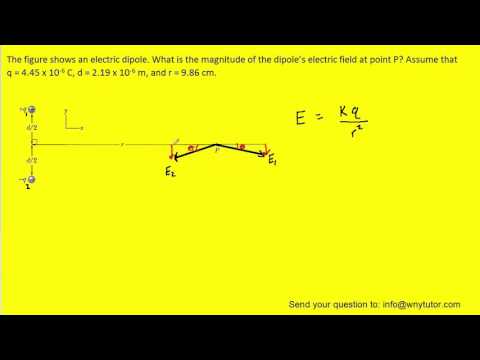
What is the magnitude of the dipole’s electric field at point P
Magnitude of electric field created by a charge | Physics | Khan Academy
Physics 36 Electric Field (6 of 18) Infinite Line Charge
JEE Main Physics E & M #5 The Electric Field
Electric Charge and Electric Fields
Physics 36 Electric Field (18 of 18) Line Charge with Positive and Negative Charges
Physics 36 Electric Field (16 of 18) Finite Line Charge with Varying Linear Charge
Electric Field Due to a Continuous Charge Distribution
Electric Field Midway
A Level Physics Revision: All of Electric Fields (in under 30 minutes)
Semi-Circle Electric Field: Uniform and non-uniform charge density
Physics 36 Electric Field (12 of 18) Infinite Sheet of Charge
Electric field definition | Electric charge, field, and potential | Physics | Khan Academy
Physics - E&M: Ch 36.1 The Electric Field Understood (10 of 17) Find the Electric Field Ex. 2
Комментарии
 0:04:11
0:04:11
 0:11:41
0:11:41
 0:59:59
0:59:59
 0:08:20
0:08:20
 0:09:01
0:09:01
 0:08:14
0:08:14
 0:13:20
0:13:20
 0:10:37
0:10:37
 0:29:41
0:29:41
 0:08:06
0:08:06
 0:07:05
0:07:05
 0:10:08
0:10:08
 0:11:32
0:11:32
 0:09:10
0:09:10
 0:06:41
0:06:41
 0:12:39
0:12:39
 0:10:53
0:10:53
 0:15:15
0:15:15
 0:07:16
0:07:16
 0:28:43
0:28:43
 0:21:41
0:21:41
 0:10:22
0:10:22
 0:13:46
0:13:46
 0:07:14
0:07:14