Calculus 3: Partial Derivative (14 of 30) Find More Partial Derivatives: Example (2 of 2)

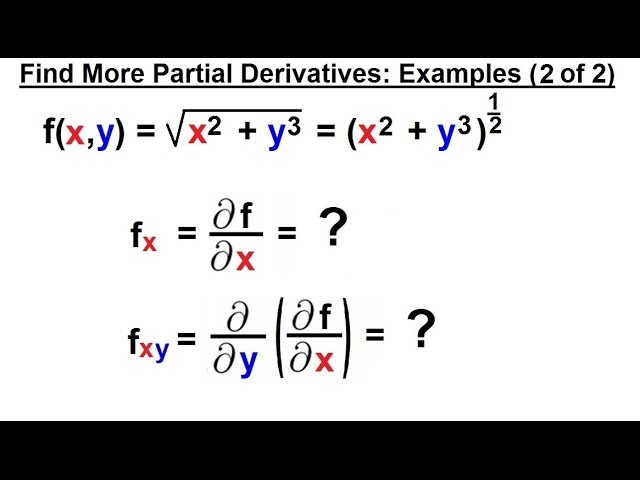
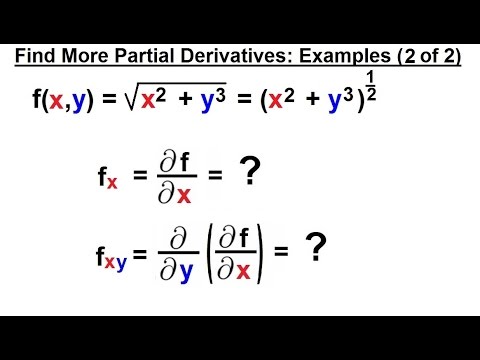
Показать описание

In this video I will find the 1st and 2nd partial derivative with-respect-to x and y of f(x,y)=(x^2+y^3)^(1/2).

Next video in the series can be seen at:

Рекомендации по теме

Комментарии

Can't thank you enough for this. This is a similar problem to the one in my Larson book section 13.6 #9

Capitan_Chaos

Thankyou very much professor Van Biezan! You are the best.

shaneaipa

thanks Professor Van Biezan! you are the best!

faizandarsot

@1:30 Is the quotient rule necessary? Since we treat x as a constant when taking the partial derivative with respect to y, I merely multiplied the numerator x, by the derivative of the denominator. So...

Partial derivative of (x)*[(x^2+y^3)^-1/2] with respect to y becomes:

Rewrite:

...which becomes:

-3x(y^2) / ((x^2+y^3)^(3/2))

...which is the same answer as you got in the video.

ssrrapper

Sir, the equality of Fxy(0, 0)and Fyx (0, 0) of the function f(x, y ) =[root over (x2 +y2)] sin2@

surjapurilarka

hey..! i was good at maths but now i am not getting it ln calculus any advise unlike

but if we should practice then tell me how to practice
and how to manage other subjects as well
please

hooriakhan

If it was sqrt(x^2 + y^2), could I do it using polar coordinates?😯

nilsoncampos

Walker Cynthia Lee Elizabeth Young Kimberly

FannyMag

You are like my teacher, this videos is not for you explain it better.

forcommentpurposes

Professor, isn't the derivative of x equal to 1, not 0?

dhanushkakonara

Calculus 3: Partial Derivative (14 of 30) Find More Partial Derivatives: Example (2 of 2)

14.3 Partial derivative | Anas Abu Zahra

Calculus 3 Lecture 13.3: Partial Derivatives (Derivatives of Multivariable Functions)

Partial Derivatives - Multivariable Calculus

Calculus 3: Partial Derivative (14 of 30) Find More Partial Derivatives: Example (2 of 2)

14.1 functions of several variables | Anas Abu Zahra

Calculus 14.3 Partial Derivatives

Calculus 3: Partial Derivatives (Video #13) | Math with Professor V

14.3: Partial Derivatives

Lec-14 | Applications of Derivatives-II | Calculus | Engineering Mathematics |#FMIH | Shailendra Sir

Chain Rule With Partial Derivatives - Multivariable Calculus

Implicit Differentiation With Partial Derivatives Using The Implicit Function Theorem | Calculus 3

Calculus 3 -- Partial derivatives -- Overview

Partial Derivatives ; Lec | 57 ; Ex: 14.3 | Thomas Calculus

Absolute Maximum and Minimum Values of Multivariable Functions - Calculus 3

Calculus 3 -- Partial derivatives -- Practice

14.1 Domain and range for multi-variable functions

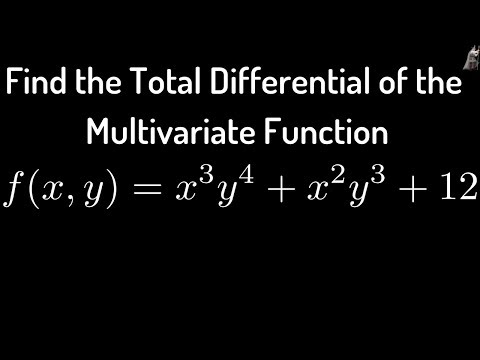
Total Differential of Multivariate Function f(x, y) = x^3y^4 + x^2y^3 + 12

14.6 Directional derivative and gradient | Anas Abu Zahra

Calculus 3 Lecture 13.1: Intro to Multivariable Functions (Domain, Sketching, Level Curves)

Calculus 3 Chapter 14 Review & Test

Partial Derivatives (Quick Example)

Calculus 3: Partial Derivative (24 of 50) The Chain Rule

Continuity vs Partial Derivatives vs Differentiability | My Favorite Multivariable Function

Thomas Calculus Exercise 14.3 Q. 1 to 15 first partial derivatives.