Symmetric matrices

preview_player
Показать описание
Symmetric matrices have nice properties. We show that all the eigenvalues of a real symmetric matrix are real, and the eigenvectors corresponding to different eigenvalues are orthogonal. We further show the diagonalization and the spectral decomposition of a symmetric matrix.

Subscribe:

Twitter:
  1. Brunei Math Club
  2. Mathematics
  3. linear algebra
  4. symmetric matrix
  5. spectral decomposition
  6. diagonalization
Рекомендации по теме
Symmetric Matrix | 0:06:47

Symmetric Matrix | Don't Memorise

Symmetric Matrices, Real 0:15:55

Symmetric Matrices, Real Eigenvalues, Orthogonal Eigenvectors

Symmetric Matrices and 0:10:14

Symmetric Matrices and their Properties | Linear Algebra

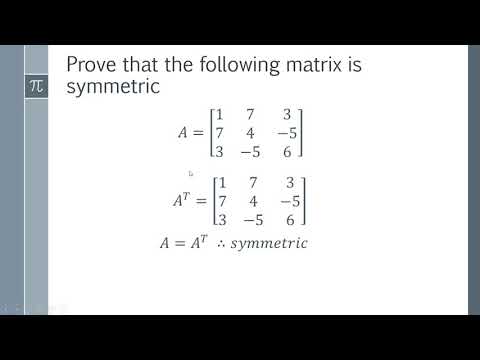
How to Prove 0:01:22

How to Prove a Matrix is Symmetric

Symmetric Matrix 0:10:37

Symmetric Matrix

🔷06 - Symmetric 0:11:31

🔷06 - Symmetric and Skew Symmetric Matrices | Properties

Visualize Spectral Decomposition 0:15:55

Visualize Spectral Decomposition | SEE Matrix, Chapter 2

Skew-symmetric Matrix | 0:05:16

Skew-symmetric Matrix | Don't Memorise

Symmetric and Skew 0:53:15

Symmetric and Skew Symmetric Matrices Class 12 maths for Board | JEE Maths by Ashwani Sir

Symmetric Matrix 0:25:51

Symmetric Matrix

Symmetric and Skew 0:03:46

Symmetric and Skew symmetric matrix - Problem Solving Techniques

Diagonalization of Real 0:08:31

Diagonalization of Real Symmetric Matrices - Linear Algebra - F8

Eigenvectors of Symmetric 0:11:28

Eigenvectors of Symmetric Matrices Are Orthogonal

Symmetric Matrix Solved 0:02:00

Symmetric Matrix Solved Problem || Find a&b Values

What is a 0:04:25

What is a Skew-Symmetric Matrix?

Class 12th – 0:07:00

Class 12th – Overview of Symmetric Matrix | Matrices | Tutorials Point

Properties of Symmetric 0:11:52

Properties of Symmetric Matrices

7.1 - Diagonalization 0:23:00

7.1 - Diagonalization of Symmetric Matrices

How to Find 0:03:14

How to Find Symmetric Matrix Eigenvalues

Symmetric and Skew 0:07:20

Symmetric and Skew Symmetric Matrix | Matrices | Class 12 Maths | #Epselon

Linear Algebra - 0:10:12

Linear Algebra - Diagonalization of Symmetric Matrices

Linear Algebra 90 0:06:33

Linear Algebra 90 Symmetric Matrices, proofs

Best way to 0:00:57

Best way to understand Symmetric and Skew Symmetric Matrix 🙋🏻‍♂️📖

Symmetric Matrices and 0:12:40

Symmetric Matrices and Positive Definiteness

Комментарии
Автор

The eigen basis is dual to the standard basis -- conjugacy is dual, spectral decomposition.
The integers are self dual as they are their own conjugates.
"Always two there are" -- Yoda.
Real is dual to imaginary -- complex numbers are dual.
Antipodal points identify for the rotation group SO(3) -- stereographic projection.

hyperduality