filmov
tv
Trigonometric Identity: sin(π-x) = sin(x)
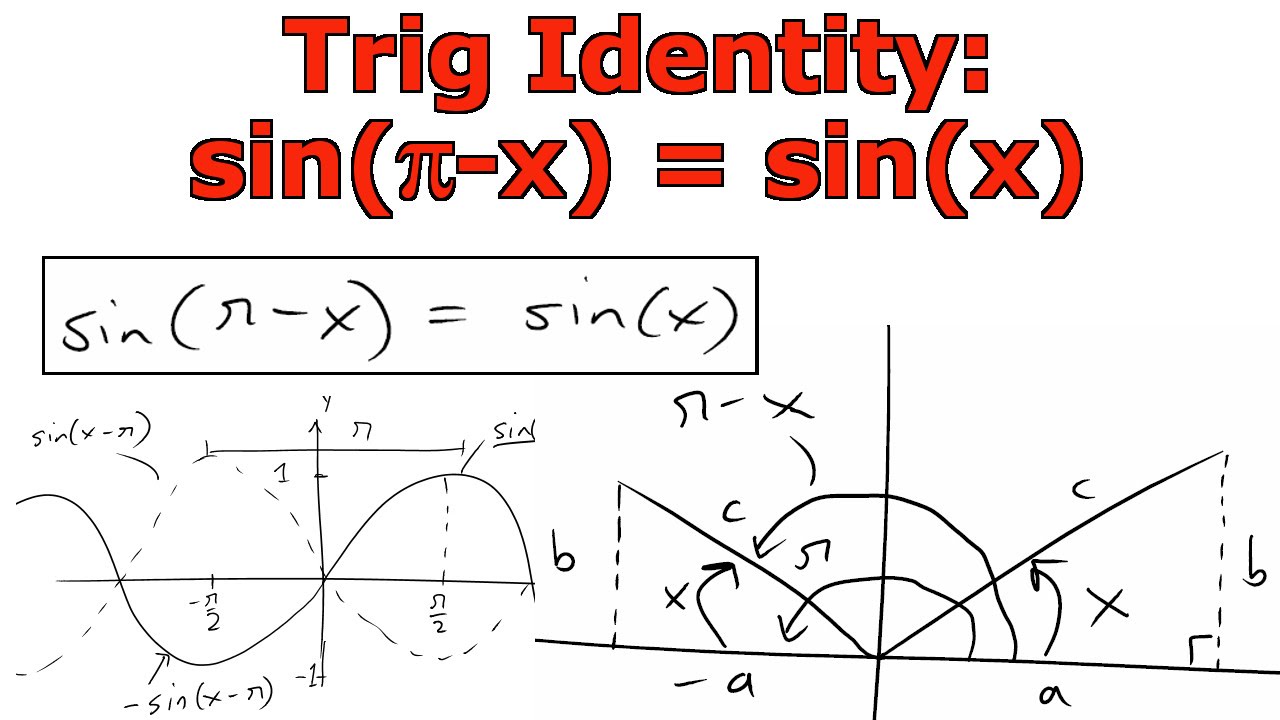
Показать описание
In this video I go over an overtly detailed analysis of the trig identity sin(π-x) = sin(x). I go over the simple proof of it but then illustrate how the graph of sin(x) gets shifted to the left by π and then flipped vertically and ending up coinciding with sin(x). This is an interesting trig identity because it's unlike the usual trig identities which involve constants being subtracted from x, such as sin(x-π) = -sin(x), as opposed to the other way around.
Related Videos:
------------------------------------------------------
Related Videos:
------------------------------------------------------
0:08:27
Trigonometric Identity: sin(π-x) = sin(x)
0:01:26
Verify The Trigonometric Identity sin(x + pi) = -sin(x)
0:01:57
Establish sum and difference identity sin(pi - x) = sin x
0:01:26
Prove the identity: sin(π-x)=sin x | Plainmath
0:02:04
Trigonometric Identity sin(π-x) = sin(x)
0:02:06
Establish sum and difference identity sin(pi + x) = - sin x
0:06:16
Proof sin(x) = sin(pi - x)
0:00:43
when calculus students use trig identities too early
0:03:04
Verify the Trigonometric Identity cos(pi/2 + x) = -sin(x)
0:01:44
sin(π+x)=-sinx | sin(180+x)=-sinx trigonometry solve
0:05:25
Prove that (i) sin(pi+x)=-sin x (ii) sin(pi-x)=sin x (iii) cos(pi+x)=-cos x (ii) cos(pi-x)=-cos x
0:03:24
Why sin(pi-x) = sinx?
0:07:07
Trigonometry Identities: cos(2π + x), sin(2π + x), cos(π/2 -x), sin(π/2 -x)
0:00:16
#Trigonometry all formulas
0:03:29
Verify the Trigonometric Identity cos(x - pi/2) = sin(x)
0:01:00
And You Thought Trigonometry Was Pointless…
0:00:56
prove sin(x - pi) = -sinx
0:00:46
Show the trig identity sin( pi/2 + theta) = cos theta
0:04:14
cos (pi/2 - x) = sin x 🔥 sin (pi/2 - x) = cos x 🔥 Solved trigonometric identities by #mathemafia...
0:00:57
Pre-Calculus - How to simplify a trig expression using co-function, sin((pi/2) -x)/cos((pi/2) -x)
0:03:56
Cofunction Trigonometric Identity Explanation sin(3pi/2 - x)
0:01:27
Pre-Calculus - Simplify expressions using fundamental identities, sin(pi/2 - x)/cos(pi/2 - x)
0:01:30
Verify The Trigonometric Identity sin(x + (1/4)pi) = (sqrt(2))/2)(sin(x) + cos(x))
0:03:05
Verify the Trigonometric Identity cos(3pi/2 + x) = sin(x)
Комментарии