Taylor polynomial remainder (part 1) | Series | AP Calculus BC | Khan Academy

Показать описание

The more terms we have in a Taylor polynomial approximation of a function, the closer we get to the function. But HOW close? Let's embark on a journey to find a bound for the error of a Taylor polynomial approximation. Created by Sal Khan.

AP Calculus BC on Khan Academy: Learn AP Calculus BC - everything from AP Calculus AB plus a few extra goodies, such as Taylor series, to prepare you for the AP Test

For free. For everyone. Forever. #YouCanLearnAnything

Khan Academy

Рекомендации по теме

Комментарии

This guy is always here to rescue when I don't understand something. He makes it so easy.

uranium-ho

It would be nice if you would include an example with

edrickramos

The first time I saw Taylor Series in Calc II I had no idea what I was doing.
Now that I'm taking D.Es, this is finally starting to make sense and I can say
that having a good understanding of Taylor Series will prove to be extremely useful.

icee

I loved the colors in this vid :) makes studying a tiny bit better.

ginayang

I Really Like The Video Understanding the properties of the remainder or error function for an Nth degree Taylor approximation of a function From Your

imegatrone

i cant be more thankful bro u saved my day

Riddimental

Thanks sal for this wonderful video..i really appreciate it..

Amirsyafiq

We should eat fresh
We should drink fresh
But most importantly we should keep our hand fresh

keshavchaturvedi

died laughing when he said screen real-estate

jonathanjaycheng

This is in the realms of numerical analysis.

CLfreak

Very helpful, get some idea behind those equations, Thanks Khan!!

yifeipeng

Nobody taught us like this 😭....thanku 👍

anushkaagrahari

didn't get it at all so confusing this course, change variable as his wish really confusing

nara

00:04 An "arbitrary" f(x) needs to have n+1 derivatives in the neighborhood of a according to your lesson.

tobiaszb

Only if there was a example in the video

harshitsinghai

man i watched the first few minutes and i already understand my whole class hahahah

goddess_ofchaos

Pi is also wrong. It gives shorter circumference length if compared to the actual circunference in reality. Tiny arcs are confused with straight lines. It’s an ignoring of some length/area that is actually exist.

What if the slice is so tiny till millions parts? They’re supposed to be counted as well. In fact, not.

gwebangetforever

Pet Norvig and Sebastian Thrun reference Sal's videos on their ai-class website!

geekoist

LOL 5:20 not going to write subscript "to keep my hand fresh"

maple

In my understanding n equal not N. to me n+1=N. So what is N+1? to me N+1 does not exist. If it is exist to you please show me how!! If you care.

Iberedmas

Taylor polynomial remainder (part 1) | Series | AP Calculus BC | Khan Academy

Taylor polynomial remainder (part 1) | Series | AP Calculus BC | Khan Academy

📚 Finding the Remainder of a Taylor Polynomial – Example Using Taylor’s Remainder Theorem 📚...

Taylor's Remainder Theorem

Taylor polynomial remainder (part 2) | Series | AP Calculus BC | Khan Academy

Taylor's Theorem with Remainder

Taylor's Theorem for Remainders

Why Taylor Series actually work: The Taylor Inequality

Example 1: Taylor Series Remainder Theorem

Taylor's Theorem : Remainder for 1/(1-x)

Math Integral Calculus - Taylor polynomial remainder part 1

Taylor's Remainder Theorem - Finding the Remainder, Ex 1

Taylor Polynomial Dance

Taylor Polynomials & Maclaurin Polynomials With Approximations

Calculus 1: Taylor's Theorem with Remainder term for Lab 4, 3-12-21

Taylor series | Chapter 11, Essence of calculus

Taylor & Maclaurin polynomials intro (part 1) | Series | AP Calculus BC | Khan Academy

Proof of Taylor's theorem with integral remainder

Taylor Series Applications Video 1 - Taylor's Remainder Theorem

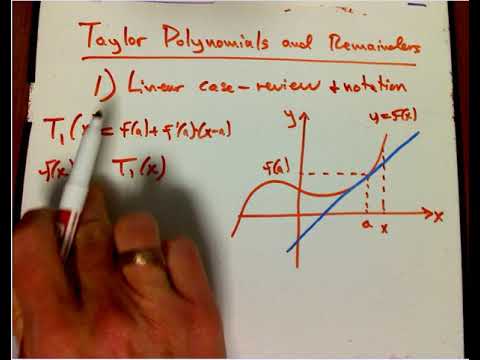
Taylor Polynomials and Remainders (Part 1)

Taylor's Theorem with Remainder- Examples 1 and 2

Taylor's Remainder Theorem - Finding the Remainder, Ex 2

Taylor Polynomials #5 Taylor's Remainder Theorem

Taylor's Remainder Theorem - Finding the Remainder, Ex 3

Find degree of Taylor polynomial so error is less than a given error value