filmov
tv
Introductory Conservation of Momentum Explosion Problem Demonstration

Показать описание
Now that we have learned about conservation of momentum, let’s apply what we have learned to an “explosion”. Okay, it’s really just the nerd-a-pult launching a ball while on momentum carts.
Want lecture notes?
This is an AP Physics 1 Topic.
0:00 Intro
0:38 The demonstration
1:16 The known values
2:07 Solving the problem using conservation of momentum
4:00 Measuring the final velocity of the nerd-a-pult
4:39 Determining relative error
5:09 What happens with a less massive projectile?
Next Video: Introduction to Elastic and Inelastic Collisions
Multilingual? Please help translate Flipping Physics videos!
Previous Video: Introduction to Conservation of Momentum with Demonstrations
Please support me on Patreon!
Want lecture notes?
This is an AP Physics 1 Topic.
0:00 Intro
0:38 The demonstration
1:16 The known values
2:07 Solving the problem using conservation of momentum
4:00 Measuring the final velocity of the nerd-a-pult
4:39 Determining relative error
5:09 What happens with a less massive projectile?
Next Video: Introduction to Elastic and Inelastic Collisions
Multilingual? Please help translate Flipping Physics videos!
Previous Video: Introduction to Conservation of Momentum with Demonstrations
Please support me on Patreon!
Introductory Conservation of Momentum Explosion Problem Demonstration
Momentum Explosions
Introduction to Conservation of Momentum with Demonstrations
Conservation of Momentum - Explosions
Conservation of Momentum & Explosions | A Level Physics
An Introductory Conservation of Momentum Problem - Exploding Firecracker
Is Momentum Conserved in an Explosion?
Conservation of Momentum Physics Problems - Basic Introduction
Slow Motion Rocket Demonstration (Conservation of Momentum)
Conservation of Momentum In Two Dimensions - 2D Elastic & Inelastic Collisions - Physics Problem...
Explosions | Momentum | Physics
Explosions - Conservation of Linear Momentum
AP Physics Lesson 23: Momentum Explosions
Conservation of Momentum: Explosion problem
Conservation of momentum - Explosion
Investigating conservation of momentum in explosions using PASCO Smart Carts
Honors Physics Video 5.10 Conservation of Momentum in Explosions
Momentum - Skateboarder problem - An example of an explosion problem
A level physics momentum - explosions
Elastic and Inelastic Collisions
Momentum Explosion Conservation Physics Help
Conservation of Momentum Derivation and Rocket Demonstration
AP Physics Video 4.3 Conservation of Momentum Explosions & Collisions
PHYS-101-Conservation of Linear Momentum Example explosion
Комментарии
 0:05:39
0:05:39
 0:08:00
0:08:00
 0:03:58
0:03:58
 0:04:30
0:04:30
 0:02:54
0:02:54
 0:05:08
0:05:08
 0:07:07
0:07:07
 0:12:19
0:12:19
 0:04:00
0:04:00
 0:10:25
0:10:25
 0:15:23
0:15:23
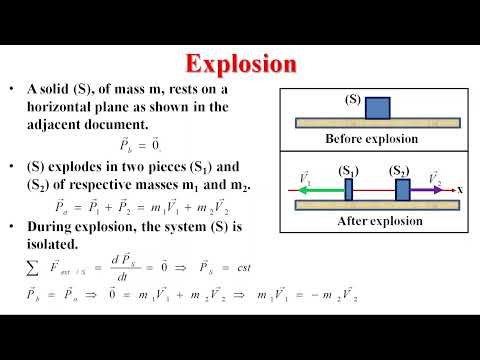 0:06:04
0:06:04
 0:13:04
0:13:04
 0:04:08
0:04:08
 0:00:12
0:00:12
 0:10:22
0:10:22
 0:04:43
0:04:43
 0:06:48
0:06:48
 0:08:14
0:08:14
 0:05:14
0:05:14
 0:06:37
0:06:37
 0:08:35
0:08:35
 0:13:43
0:13:43
 0:12:28
0:12:28