Linear Equations in Two Variable PYQ

Linear equations are algebraic equations that express a linear relationship between two variables. In Grade 10, you'll explore the properties, graphing, and applications of linear equations.

Key Concepts
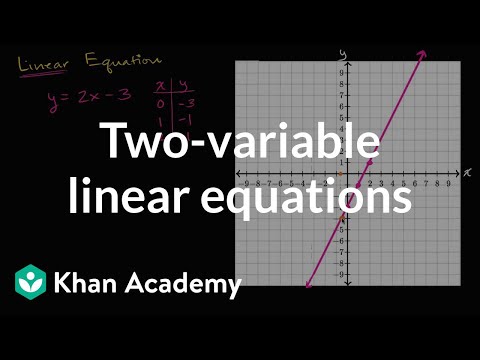
Slope-Intercept Form: y = mx + b, where m is the slope and b is the y-intercept.
Point-Slope Form: y - y1 = m(x - x1), where (x1, y1) is a point on the line and m is the slope.  
Standard Form: Ax + By = C, where A, B, and C are constants.  
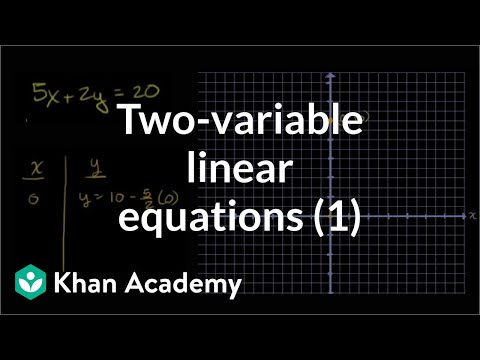
Graphing Linear Equations: Plotting points that satisfy the equation and connecting them to form a straight line.
Systems of Linear Equations: Two or more linear equations with the same variables.
Solving Systems of Linear Equations: Finding the values of the variables that satisfy all equations in the system.
Applications of Linear Equations
Geometry: Finding equations of lines, determining intersections, and calculating distances.
Science: Modeling relationships between variables.
Economics: Analyzing supply and demand, pricing, and profit.
Engineering: Designing structures and systems.
Practice Problems
Slope-Intercept Form: Find the equation of the line with slope 2 and y-intercept 3.

Point-Slope Form: Find the equation of the line passing through the points (2, 3) and (4, 7).

Standard Form: Convert the equation y = 2x - 5 to standard form.

Graphing: Graph the equation y = -3x + 4.

Systems of Linear Equations: Solve the following system of equations:

2x + 3y = 7
3x - 2y = 1