Proof: Monotone Sequence has Monotone Subsequences | Real Analysis

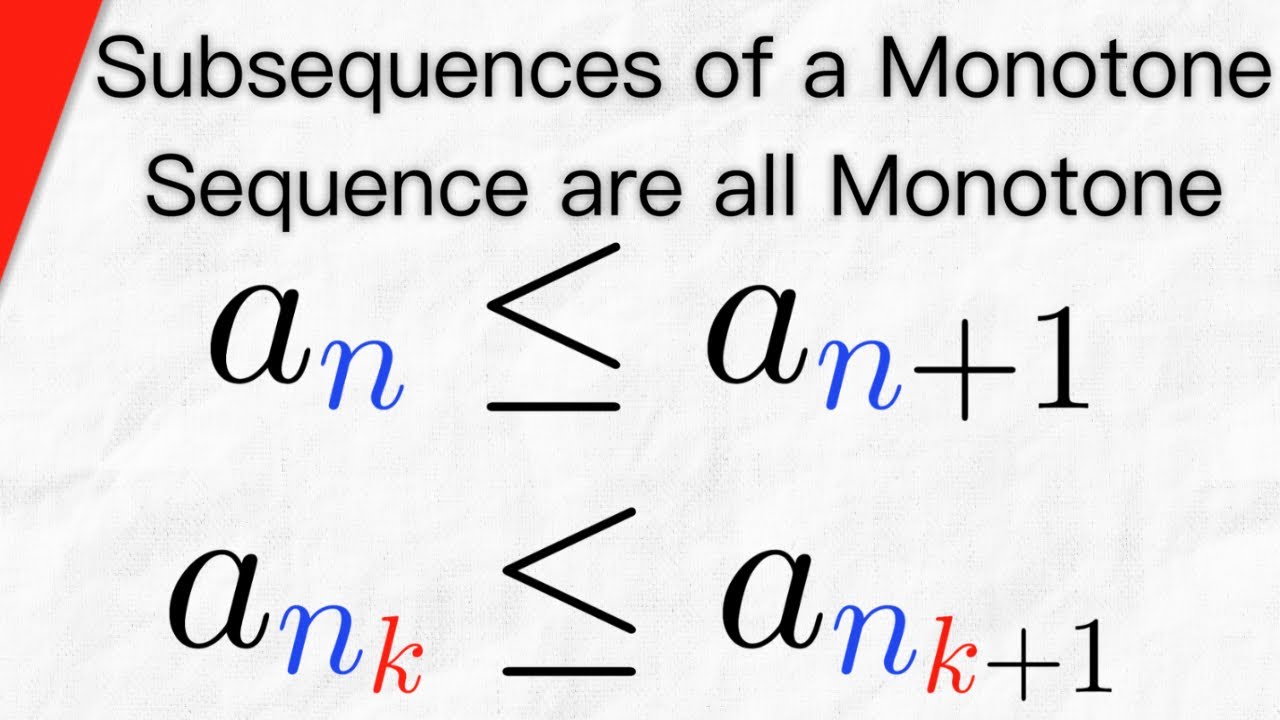
preview_player
Показать описание
We prove if a sequence is monotone then all of its subsequences are monotone. In particular, we prove that all subsequences of an increasing sequence are increasing and all subsequences of a decreasing sequence are decreasing. We prove this using the definition of a monotone sequence. #RealAnalysis

★DONATE★

Thanks to Robert Rennie, Barbara Sharrock, and Rolf Waefler for their generous support on Patreon!

Follow Wrath of Math on...

  1. Wrath of Math
  2. wrath of math
  3. math lessons
  4. math
  5. education
  6. math video
Рекомендации по теме
Proof: Monotone Sequence 0:06:10

Proof: Monotone Sequence has Monotone Subsequences | Real Analysis

Monotone Subsequence Theorem 0:09:14

Monotone Subsequence Theorem (Every Sequence has Monotone Subsequence) | Real Analysis

Detailed Proof of 0:13:44

Detailed Proof of the Monotone Convergence Theorem | Real Analysis

What are Monotone 0:12:41

What are Monotone Sequences? | Real Analysis

Real Analysis | 0:09:17

Real Analysis | The monotone sequence theorem.

Monotonic Sequences and 0:31:23

Monotonic Sequences and Bounded Sequences - Calculus 2

Monotone subsequence 0:08:14

Monotone subsequence

How to prove 0:15:29

How to prove monotone sequences

Monotone subsequence theorem 0:20:32

Monotone subsequence theorem || Every sequence has monotone subsequence ||

Monotone Sequence Theorem 0:19:05

Monotone Sequence Theorem

Monotone Convergence Theorem 0:06:38

Monotone Convergence Theorem for Sequences

Monotone Subsequence Theorem 0:13:38

Monotone Subsequence Theorem | Every Sequence of Real Numbers has Monotonic Subsequence

EVERY REAL SEQUENCE 0:24:43

EVERY REAL SEQUENCE HAS A MONOTONE SUBSEQUENCE | RIGOROUS PROOF

Prove that every 0:11:44

Prove that every sequence has a monotone subsequence (ILIEKMATHPHYSICS)

Bounded and Monotone 0:26:15

Bounded and Monotone Sequences

Monotone Convergence Theorem 0:17:25

Monotone Convergence Theorem Easiest Proof

Monotone Sequence with 0:09:49

Monotone Sequence with Convergent Subsequence Converges | Real Analysis

11.6 The monotone 0:07:47

11.6 The monotone convergence theorem for sequences

Proof : Every 0:33:03

Proof : Every Real Sequence has a Monotone Subsequence.

Using the Monotone 0:05:50

Using the Monotone Convergence Theorem! | Real Analysis

Real Analysis | 0:08:46

Real Analysis | Monotone sequence theorem example.

401.6 Using Monotone 0:11:54

401.6 Using Monotone Convergence

Using the Monotone 0:04:54

Using the Monotone Convergence Theorem to Prove a Recursive Sequence Converges: Example 1

Monotonic Sequences and 0:23:45

Monotonic Sequences and Subsequences.

Комментарии
Автор

It' really well explained, thank you but I'm not clear about at 4:37 how can we say that a_n_k > a_n_k + 1 ? Don't we need to write it as a_n_k > a_n_k -1 > a_n_k - 2 > ... > a_n_k+1 ?

ruffionline