Using the Monotone Convergence Theorem to Prove a Recursive Sequence Converges: Example 1

preview_player
Показать описание
We consider a recursive sequence. We show that the sequence is bounded and monotone. We apply the Monotone Convergence Theorem to show that the sequence converges. This is our first example.

#mikedabkowski, #mikethemathematician, #profdabkowski, #realanalysis
  1. Mike, the Mathematician
  2. recursive sequences
  3. monotone convergence theorem
  4. mike the mathematician
  5. mike dabkowski math
Рекомендации по теме
Using the Monotone 0:05:50

Using the Monotone Convergence Theorem! | Real Analysis

Detailed Proof of 0:13:44

Detailed Proof of the Monotone Convergence Theorem | Real Analysis

How can the 0:05:45

How can the Monotone Convergence Theorem help? - Week 1 - Lecture 15 - Sequences and Series

Using the Monotone 0:04:54

Using the Monotone Convergence Theorem to Prove a Recursive Sequence Converges: Example 1

Monotonic Sequences and 0:31:23

Monotonic Sequences and Bounded Sequences - Calculus 2

Monotone Convergence Theorem 0:08:55

Monotone Convergence Theorem

Monotone Convergence Theorem 0:06:38

Monotone Convergence Theorem for Sequences

401.6 Using Monotone 0:11:54

401.6 Using Monotone Convergence

The Monotone Convergence 0:14:39

The Monotone Convergence Theorem - Example

Monotone Convergence Theorem 0:25:11

Monotone Convergence Theorem - Example

Real Analysis | 0:09:17

Real Analysis | The monotone sequence theorem.

11.6 The monotone 0:07:47

11.6 The monotone convergence theorem for sequences

Monotone Convergence Theorem: 0:11:05

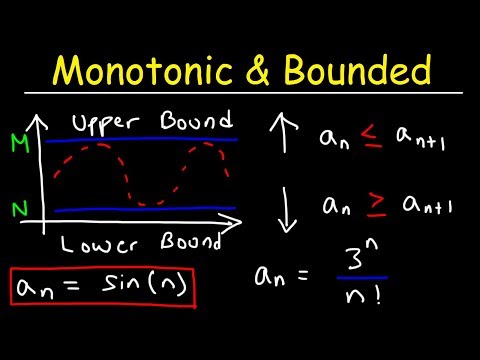
Monotone Convergence Theorem: Explained with graph and examples.

Monotone Convergence Theorem 0:03:14

Monotone Convergence Theorem --- Part 1

Using the Monotone 0:05:00

Using the Monotone Convergence Theorem to Prove a Recursive Sequence Converges: Example 2

Measure Theory 7 0:19:53

Measure Theory 7 | Monotone Convergence Theorem (and more)

The Monotone Convergence 0:20:53

The Monotone Convergence Theorem

Using the Monotone 0:18:16

Using the Monotone Convergence Theorem

Sequences 4 - 0:05:15

Sequences 4 - Example of Monotone Convergence Theorem

How to prove 0:15:29

How to prove monotone sequences

What are Monotone 0:12:41

What are Monotone Sequences? | Real Analysis

Monotone Convergence Theorem 0:05:09

Monotone Convergence Theorem [Real Analysis]

Monotone Convergence Theorem 0:08:31

Monotone Convergence Theorem - Intuition

monotonic Sequences full 0:00:05

monotonic Sequences full definition and example most IIT JAM

Комментарии
Автор

You are the best, thank you. I have been having trouble proving convergence with recursive sequences. Appreciate it!

scienceappreciator
Автор

What would the be process for lets say x_1 = a (a is from the set of the Reals), x_{n+1} = x^2_n + x_n? This I already calculated and the sequence converges to 0 for any a from (-1, 1) but how do I prove it? Or is it neccessary to always prove this stuff via induction?

danilojonic
Автор

why we consider that an is bigger than one half?

KefaleLiche