filmov
tv
Find two solutions for two equations
Показать описание
Find two solutions for two equations
In this lesson you will find two solutions for one linear and one quadratic equation by looking at their intersection on a graph.
ADDITIONAL MATERIALS
STANDARDS
CCSS.HSA-REI.D.11 Explain why the 𝘹-coordinates of the points where the graphs of the equations 𝘺 = 𝘧(𝘹) and 𝘺 = 𝑔(𝘹) intersect are the solutions of the equation 𝘧(𝘹) = 𝑔(𝘹); find the solutions approximately, e.g., using technology to graph the functions, make tables of values, or find successive approximations. Include cases where 𝘧(𝘹) and/or 𝑔(𝘹) are linear, polynomial, rational, absolute value, exponential, and logarithmic functions.
VA.EI.AII.4 The student will solve systems of linear-quadratic and quadratic-quadratic equations, algebraically and graphically.
FL.MAFS.912.A-REI.4.11 Explain why the 𝘹-coordinates of the points where the graphs of the equations 𝘺 = 𝘧(𝘹) and 𝘺 = 𝑔(𝘹) intersect are the solutions of the equation 𝘧(𝘹) = 𝑔(𝘹); find the solutions approximately, e.g., using technology to graph the functions, make tables of values, or find successive approximations. Include cases where 𝘧(𝘹) and/or 𝑔(𝘹) are linear, polynomial, rational, absolute value, exponential, and logarithmic functions.
In this lesson you will find two solutions for one linear and one quadratic equation by looking at their intersection on a graph.
ADDITIONAL MATERIALS
STANDARDS
CCSS.HSA-REI.D.11 Explain why the 𝘹-coordinates of the points where the graphs of the equations 𝘺 = 𝘧(𝘹) and 𝘺 = 𝑔(𝘹) intersect are the solutions of the equation 𝘧(𝘹) = 𝑔(𝘹); find the solutions approximately, e.g., using technology to graph the functions, make tables of values, or find successive approximations. Include cases where 𝘧(𝘹) and/or 𝑔(𝘹) are linear, polynomial, rational, absolute value, exponential, and logarithmic functions.
VA.EI.AII.4 The student will solve systems of linear-quadratic and quadratic-quadratic equations, algebraically and graphically.
FL.MAFS.912.A-REI.4.11 Explain why the 𝘹-coordinates of the points where the graphs of the equations 𝘺 = 𝘧(𝘹) and 𝘺 = 𝑔(𝘹) intersect are the solutions of the equation 𝘧(𝘹) = 𝑔(𝘹); find the solutions approximately, e.g., using technology to graph the functions, make tables of values, or find successive approximations. Include cases where 𝘧(𝘹) and/or 𝑔(𝘹) are linear, polynomial, rational, absolute value, exponential, and logarithmic functions.
0:05:25
0:01:23
0:06:05
0:02:36
0:01:40
0:06:16
0:02:48
0:03:12
1:26:38
0:08:50
0:21:12
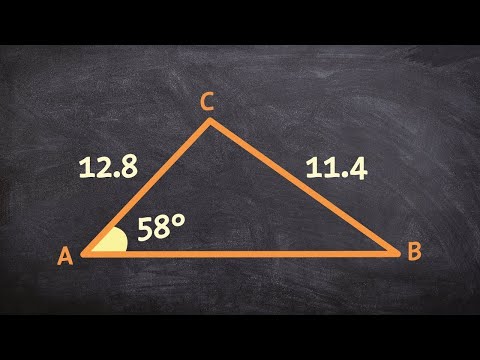0:15:58
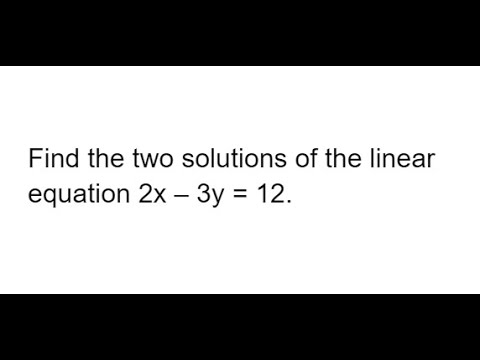0:01:16
0:00:33
0:03:48
0:04:35
0:01:17
0:08:16
0:04:59
0:12:10
0:03:44
0:07:30
0:02:15
0:05:24