Linear Algebra 13c: The Inverse of an Elementary Matrix

preview_player
Показать описание
  1. MathTheBeautiful
  2. Linear Algebra (Field Of Study)
  3. Matrix Multiplication (Literature Subject)
  4. The Matrix (Award-Winning Work)
  5. Vector
  6. Linear Equation (Literature Subject)
Рекомендации по теме
Linear Algebra 13c: 0:08:00

Linear Algebra 13c: The Inverse of an Elementary Matrix

3.1.13-Linear Algebra: Matrix 0:02:09

3.1.13-Linear Algebra: Matrix Inverse

Inverse matrices, column 0:12:09

Inverse matrices, column space and null space | Chapter 7, Essence of linear algebra

Linear Algebra - 0:15:58

Linear Algebra - Lecture 24 - Elementary Matrices and Inverses

Elementary matrices | 0:11:24

Elementary matrices | Lecture 13 | Matrix Algebra for Engineers

Linear Algebra 29 0:11:01

Linear Algebra 29 | Identity and Inverses

Computing inverse matrices 0:13:13

Computing inverse matrices using Gaussian elimination | Lecture 12 | Matrix Algebra for Engineers

Left inverse and 0:13:51

Left inverse and right matrix inverses

Maths For Class 1:29:46

Maths For Class 7(B-1) Day 24 Shounak Sir (24/08/2024)( Ratio & Proportion-6)

Change of basis 0:12:51

Change of basis | Chapter 13, Essence of linear algebra

[Linear Algebra] Elementary 0:17:11

[Linear Algebra] Elementary Matrices and Inverse Algorithm

Gauss Jordan Elimination 0:10:51

Gauss Jordan Elimination & Reduced Row Echelon Form

Linear Algebra - 0:05:25

Linear Algebra - Maple (Matrix Addition Subtraction Multiplication Inverse Determinant Transpose)

Algebra - Ch. 0:06:37

Algebra - Ch. 34: Solving System of Linear Equations (13 of 31) Inverse Matrix: Method 7: Part 2

Linear Algebra: The 0:38:20

Linear Algebra: The Inverse of a Matrix Part 2 (Section 2.3)

Linear Algebra 11t: 0:08:23

Linear Algebra 11t: The Inverse of a Product of Two Matrices

Linear algebra: Prove 0:12:55

Linear algebra: Prove the Sherman-Morrison formula for computing a matrix inverse

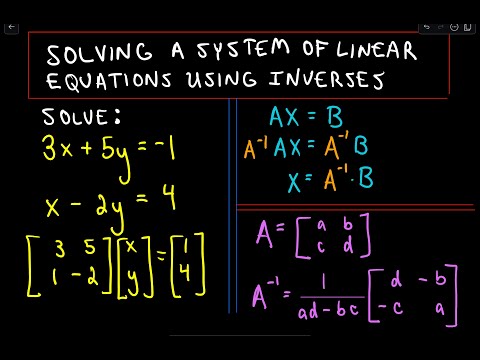
Solving a System 0:06:28

Solving a System of Linear Equations Using Inverses

Linear Algebra 11p: 0:05:15

Linear Algebra 11p: Some Matrices Are Not Invertible - I.e. They Don't Have an Inverse

Linear algebra for 0:06:12

Linear algebra for data science, chapter 13 exercise 1 (eigenvalues of the matrix inverse)

When mathematicians get 0:00:37

When mathematicians get bored (ep1)

Inverse of a 0:15:21

Inverse of a 3x3 Matrix

NEWYES Calculator VS 0:00:14

NEWYES Calculator VS Casio calculator

Linear Algebra: Ch 0:02:10

Linear Algebra: Ch 2 - Determinants (19 of 48) Example of Rule 13: Invertible Matrix

Комментарии
Автор

Absolutely beautiful! Thank you Prof Grinfeld, you are such a great teacher! :D

lochestnut
Автор

Intuitively it feels right , that looking for matrix B which is an inverse of elementary matrix A, indeed one can look for such B that will "undo" what A "do" (to I -dentity matrix) .
But to explain it to myself more formally, it helped me to introduce temporarily one more I- matrix in the game, and to group two last matrices together.
To look at the product of 3 matrices [A] [ [B][ I ] ] = I makes it (to me) more clear that matrix A "undo" on matrix [B][I ] all the actions that B "did" to I, so I is restored hence the result equals I .Finely since [B] [ I] =I , the I in the left wing I can be dropped and from what remains, per definition A, B are inverses of each other .

sergelifshitz
Автор

5:15 This reminds me of Dr. Seuss - are you having trouble saying this stuff? I just look in a mirror and I see what I say, then I say what I see.

solfeinberg