filmov
tv
Differential Calculus | Applications of Maxima and Minima (Part 2)

Показать описание
The following problems are solved in the video:
1. A printed page must contain 60 square cm of printed material. There are to be margins of 5cm on either side and margins of 3cm on the top and bottom. What are the dimensions of the printed area to minimize the amount of paper to be used?
2) A closed box with a square base is to contain 252 cubic feet. The bottom costs $5 per square foot, the top costs $2 per square foot, and the sides cost $3 per square foot. Find the dimensions that will minimize the cost.
Feel free to comment if you have questions or any suggested topics.
"Be completely humble and gentle; be patient, bearing with one another in love." - Ephesians 4:2, NIV
1. A printed page must contain 60 square cm of printed material. There are to be margins of 5cm on either side and margins of 3cm on the top and bottom. What are the dimensions of the printed area to minimize the amount of paper to be used?
2) A closed box with a square base is to contain 252 cubic feet. The bottom costs $5 per square foot, the top costs $2 per square foot, and the sides cost $3 per square foot. Find the dimensions that will minimize the cost.
Feel free to comment if you have questions or any suggested topics.
"Be completely humble and gentle; be patient, bearing with one another in love." - Ephesians 4:2, NIV
Differential Calculus- Explained in Just 4 Minutes
Marginal cost & differential calculus | Applications of derivatives | AP Calculus AB | Khan Acad...
This is why you're learning differential equations
What is Calculus used for? | How to use calculus in real life
Related Rates - Distance Problems - Application of Derivatives
APPLICATIONS OF DERIVATIVES ( DIFFERENTIAL CALCULUS WORD PROBLEM ) #differentialcalculus
Finding Local Maxima and Minima by Differentiation
Optimization Problems - Calculus
Advancing Calculus Research and Teaching with Wolfram Language
Calculus in a nutshell
19. Application of Differentiation | Rates of Change 1 | Calculus
Calculus - Application of Derivatives - Course Structure | Don't Memorise
REAL LIFE APPLICATIONS OF CALCULUS WITH EXAMPLES | MATHS IN REAL LIFE | MATHS REAL WORLD PROBLEMS
Differentiation Formulas - Notes
Differential Equation Mixing Problem, calculus 2 tutorial
Differential equations, a tourist's guide | DE1
Calculus, what is it good for?
Optimization: profit | Applications of derivatives | AP Calculus AB | Khan Academy
Related Rates in Calculus
Calculus - Application of Differentiation (1 of 60) Max/Min Values: Introduction
Understand Calculus in 35 Minutes
Optimisation Gr 12
Local linearization | Derivative applications | Differential Calculus | Khan Academy
Differentiation | Derivatives (General Method)
Комментарии
 0:03:57
0:03:57
 0:04:40
0:04:40
 0:18:36
0:18:36
 0:11:39
0:11:39
 0:18:38
0:18:38
 0:03:39
0:03:39
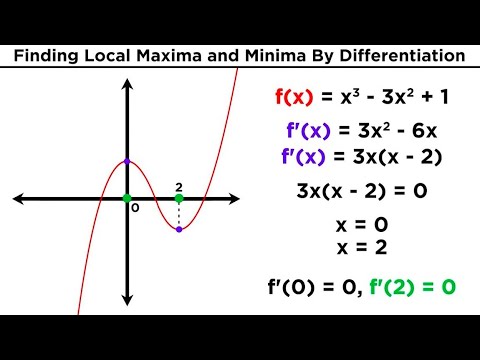 0:06:17
0:06:17
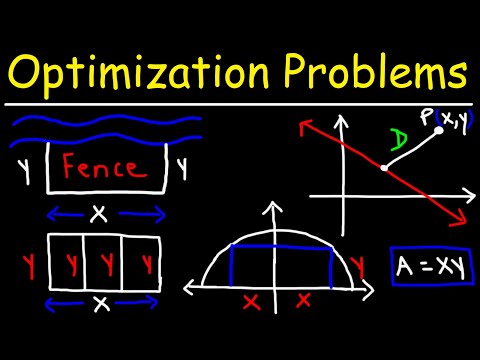 1:04:56
1:04:56
 1:24:32
1:24:32
 0:03:01
0:03:01
 0:16:38
0:16:38
 0:04:06
0:04:06
 0:07:48
0:07:48
 0:13:51
0:13:51
 0:11:47
0:11:47
 0:27:16
0:27:16
 0:07:43
0:07:43
 0:11:27
0:11:27
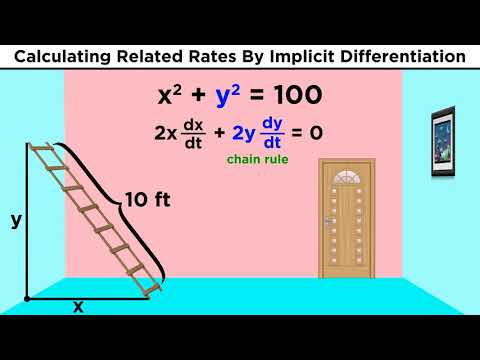 0:08:53
0:08:53
 0:01:47
0:01:47
 0:36:22
0:36:22
 0:03:30
0:03:30
 0:09:38
0:09:38
 0:13:33
0:13:33