filmov
tv
Pythagorean Identities Visualized #shorts

Показать описание
Pythagorean Identities Visualized #shorts
The Pythagorean Identities in Less Than 60 Seconds #shorts
Pythagoras’ theorem, an animated explanation!
Trig Visualized: One Diagram to Rule them All (six trig functions in one diagram)
Pythagorean Identities
And You Thought Trigonometry Was Pointless…
Pythagorean Theorem II (visual proof)
What are the 3 Pythagorean identities?
Deriving Every Trig Identity in a Minute
Pythagorean Identities
Pythagorean Identity in 8 seconds
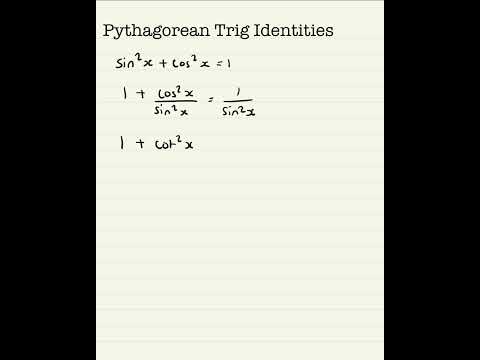
Pythagorean Trig Identities
a trig identities song???
When mathematicians get bored (ep1)
'So close 🤏🏼,yet so far ♾️' Explanation and code in description #maths #satisfying #adhd #...
Isaac Newton's INSANE Sleep Habits 😬
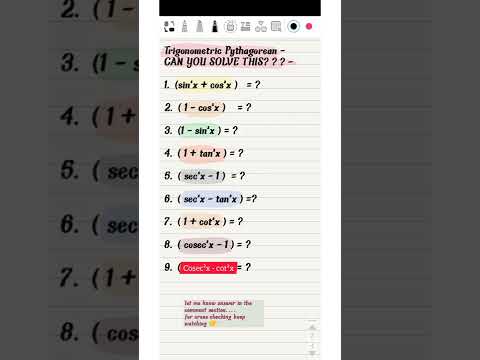
#Trigonometry - Complete the Pythagorean Identities • #shorts,#trigonomtry,#formulas
Why greatest Mathematicians are not trying to prove Riemann Hypothesis? || #short #terencetao #maths
Deriving the Law of Cosines With Geometric Algebra
Maths vs Physics
Memorization Trick for Graphing Functions Part 1 | Algebra Math Hack #shorts #math #school
Can you calculate like shakuntala Devi ?|Human calculator|Shakuntala Devi|India's World record ...
India vs japan || mathematics challenge || 😅🤣🤣🤭
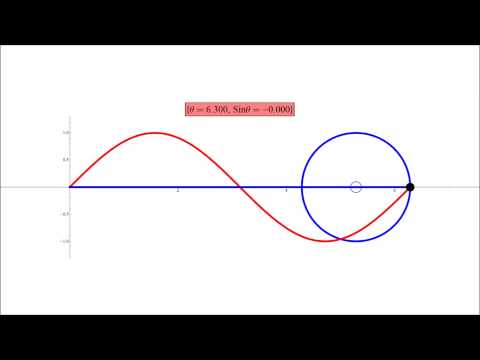
Sine Curve and the Unit Circle
Комментарии
 0:01:00
0:01:00
 0:00:54
0:00:54
 0:00:40
0:00:40
 0:04:15
0:04:15
 0:00:06
0:00:06
 0:01:00
0:01:00
 0:01:26
0:01:26
 0:00:39
0:00:39
 0:00:51
0:00:51
 0:00:54
0:00:54
 0:00:18
0:00:18
 0:00:41
0:00:41
 0:00:26
0:00:26
 0:00:37
0:00:37
 0:01:00
0:01:00
 0:00:24
0:00:24
 0:00:16
0:00:16
 0:00:38
0:00:38
 0:00:31
0:00:31
 0:00:25
0:00:25
 0:00:15
0:00:15
 0:00:34
0:00:34
 0:00:12
0:00:12
 0:00:27
0:00:27