filmov
tv
3. Probability Theory

Показать описание
MIT 18.S096 Topics in Mathematics with Applications in Finance, Fall 2013
Instructor: Choongbum Lee
This lecture is a review of the probability theory needed for the course, including random variables, probability distributions, and the Central Limit Theorem.
*NOTE: Lecture 4 was not recorded.
License: Creative Commons BY-NC-SA
Instructor: Choongbum Lee
This lecture is a review of the probability theory needed for the course, including random variables, probability distributions, and the Central Limit Theorem.
*NOTE: Lecture 4 was not recorded.
License: Creative Commons BY-NC-SA
3. Probability Theory
Probability Theory 3 | Discrete vs. Continuous Case
Introduction to Probability, Basic Overview - Sample Space, & Tree Diagrams
Math Antics - Basic Probability
Why “probability of 0” does not mean “impossible” | Probabilities of probabilities, part 2
Multiplication & Addition Rule - Probability - Mutually Exclusive & Independent Events
Probability of Complementary Events & Sample Space
Bayes theorem, the geometry of changing beliefs
Master Linear Algebra & Probability for Machine Learning
Binomial distributions | Probabilities of probabilities, part 1
Bayes' Theorem EXPLAINED with Examples
But what is the Central Limit Theorem?
Probability Theory 3 | Discrete vs. Continuous Case [dark version]
3 game theory tactics, explained
Probability
Probability explained | Independent and dependent events | Probability and Statistics | Khan Academy
Types of Probability - Classical, Empirical, Subjective - Prob / Stat
Probability - Drawing Venn Diagrams
Experimental vs Theoretical Probability
Fundamentals of Probability Theory (3/12): Conditional Probability Example
The Monty Hall Problem - Explained
Probability of Dice
3.1.3 Three Types of Probability (Classical-Theoretical, Empirical-Statistical & Subjective)
Basic Probability Part 1 Important Question Solution MBS, MBA, MPA, BBS, BBA Statistics Solution
Комментарии
 1:18:25
1:18:25
 0:11:27
0:11:27
 0:16:59
0:16:59
 0:11:28
0:11:28
 0:10:01
0:10:01
 0:10:02
0:10:02
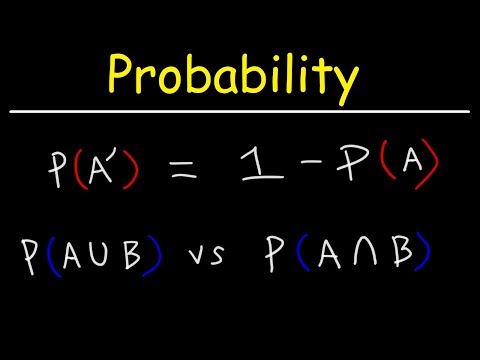 0:05:48
0:05:48
 0:15:11
0:15:11
 0:13:37
0:13:37
 0:12:34
0:12:34
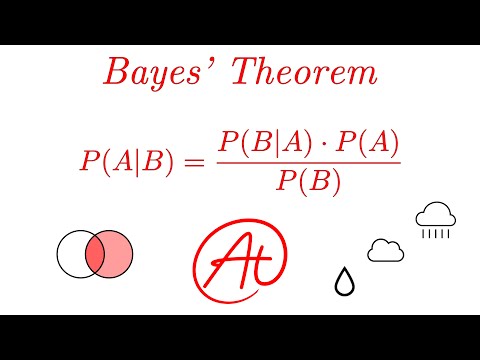 0:08:03
0:08:03
 0:31:15
0:31:15
 0:11:12
0:11:12
 0:07:11
0:07:11
 0:19:01
0:19:01
 0:08:18
0:08:18
 0:08:10
0:08:10
 0:06:13
0:06:13
 0:04:26
0:04:26
 0:03:13
0:03:13
 0:02:48
0:02:48
 0:02:44
0:02:44
 0:18:26
0:18:26
 0:11:21
0:11:21