filmov
tv
3 Children Ages Puzzle || Brilliant puzzle

Показать описание
Alex and David have the following conversation:
Alex tells David: I have 3 children and the Product of their ages is 72
The Sum of their ages is equal to my House number
David replies : I know your House number
But I still can’t tell what their ages are
Then Alex says : The eldest child loves solving puzzles
David then replies : Now I know their ages
Puzzle :
Can you tell the Ages of 3 children
Also try these mind blowing puzzles:
Difficult Puzzle || 25 Horses Race || Asked in Google and Microsoft Interviews
5 Pirates PUZZLE || 100 Gold Coins 5 Pirates || Game Theory based Problem
You can follow my facebook page ( Mohammed Ammar ):
Alex tells David: I have 3 children and the Product of their ages is 72
The Sum of their ages is equal to my House number
David replies : I know your House number
But I still can’t tell what their ages are
Then Alex says : The eldest child loves solving puzzles
David then replies : Now I know their ages
Puzzle :
Can you tell the Ages of 3 children
Also try these mind blowing puzzles:
Difficult Puzzle || 25 Horses Race || Asked in Google and Microsoft Interviews
5 Pirates PUZZLE || 100 Gold Coins 5 Pirates || Game Theory based Problem
You can follow my facebook page ( Mohammed Ammar ):
3 Children Ages Puzzle || Brilliant puzzle
The Ages Puzzle - 3 Children Puzzle
'UNSOLVABLE' Logic Puzzle: What Are Their Ages?
10 Fun Kiddy Riddles That Stump Most Adults
A genius can solve in 10 seconds! 🤯 #shorts #puzzle #riddle
Interview puzzles with answers|AGE OF THREE DAUGHTERS
Find ages of daughters Puzzle || Microsoft Interview Puzzle
What Do Puzzles do to Your Brain? A Neurology Expert Explains
Magnetic Color & Number Maze - Montessori Wooden Color Matching Learning Counting Puzzle Board #...
A Genius can solve in 15 seconds! 🤯 #shorts #riddle #puzzle #dad #marriage #funny
Race to get 3 in a row in Rubiks Cage. #puzzles
Genius test! 🤯 (10 seconds to solve!) #shorts #riddle #puzzle #games #braingames
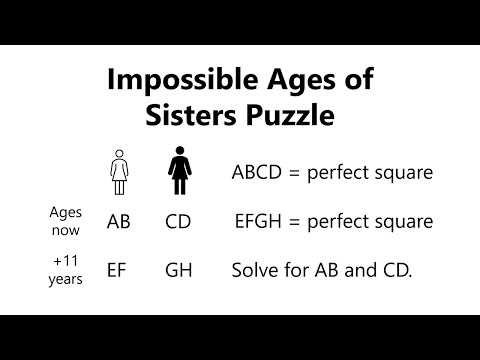
The 'Impossible' Ages of Sisters Puzzle
Match stick puzzle | Puzzles with Answer | Feed Brain with Prema
Find Ages of Daughters || Interview Puzzles
Viral Puzzle!! #shorts #puzzle #math #puzzles #aptitude #iq #cupidtwinversion @puzzleguy
Can you solve the birthday cake riddle? - Marie Brodsky
5 Seemingly Impossible Age Riddles
4 in 1 puzzle (part 3) 😀😀😍
When the puzzle says ages 5+ but you still want to be there for emotional support.
Numberblocks - Summer Puzzles | Learn to Count | Learning Blocks
children's puzzle #children #biggboss #big
Learn Wild Animal Names and Animal Videos for Kids - Safari Animal Puzzle
Genius IQ Test math puzzle🔥
Комментарии
 0:04:35
0:04:35
 0:05:38
0:05:38
 0:04:12
0:04:12
 0:11:41
0:11:41
 0:00:46
0:00:46
 0:03:56
0:03:56
 0:03:48
0:03:48
 0:03:39
0:03:39
 0:00:37
0:00:37
 0:01:00
0:01:00
 0:00:59
0:00:59
 0:01:00
0:01:00
 0:05:40
0:05:40
 0:03:39
0:03:39
 0:02:42
0:02:42
 0:00:12
0:00:12
 0:04:48
0:04:48
 0:15:21
0:15:21
 0:00:16
0:00:16
 0:00:25
0:00:25
 0:13:10
0:13:10
 0:00:16
0:00:16
 0:06:15
0:06:15
 0:00:05
0:00:05