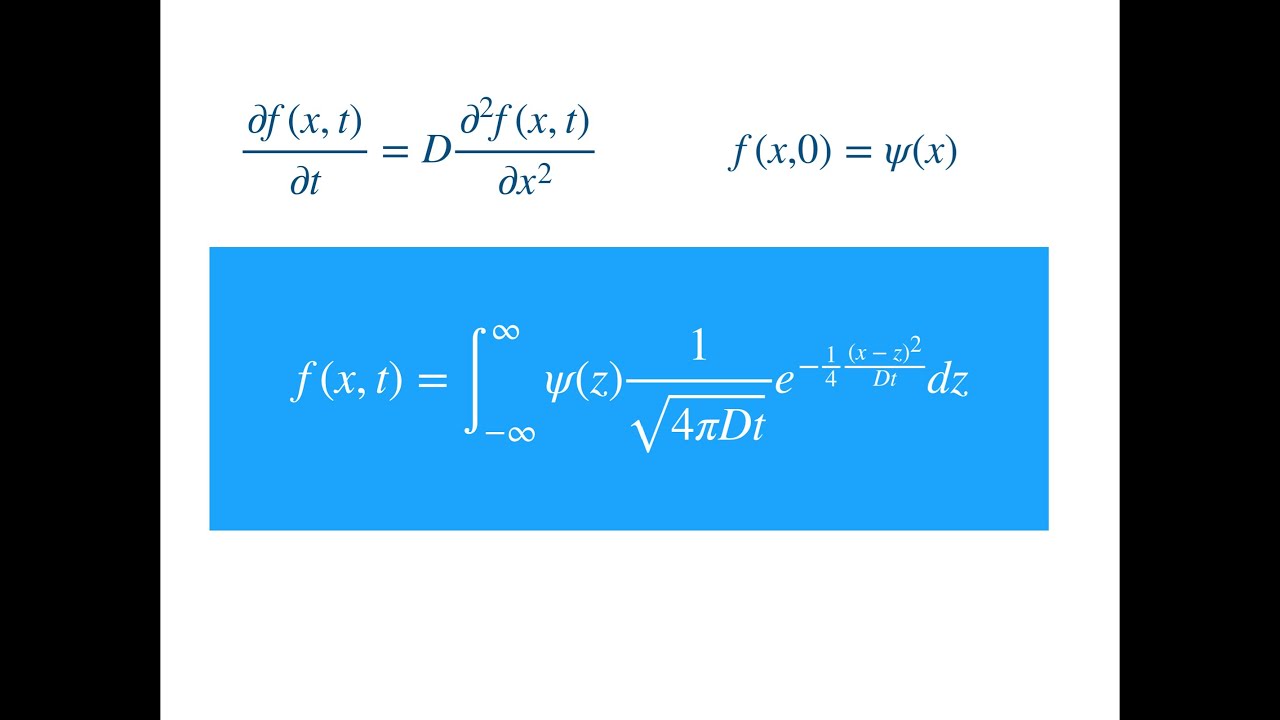
Fundamental Solution of the Diffusion Equation using the Similarity Method

Explains the derivation of the Fundamental solution of the diffusion equation, also known as heat equation, using the similarity solutions method, which you might know as the group theoretical methods of Lie.

[quantpie]

Our first live interactive session! The solution of the diffusion equation has wider uses, and for that reason, the subject will be of interest to people from very different backgrounds. It is hard to cover every possible use/approach to the problem in a video of this length, so we will be at hand to dispel any lingering doubts, and hopefully help connect the dots and our great community!

[lipaka]

Thank you very much for distilling the complex concept in a way accessible to every one. Truly exceptional!

[whosaguhboi]

Thank you very much, I was able to grasp the concept more easily with your visual explanations, after looking for hours for an explanation, this is by far the best for me.

[rohitdhawan]

At 12:21. Is it really ok to put lambda = 1/sqrt(t) because the derivation of alternative function i.e. lambda * f( lambda * x, lambda^2 * t ) which satisfies the diffusion equation assumed that lambda is independent of x and t. I am saying this because we calculated d(u)/dx and d(v)/t assuming this

[jimklm]

I didn't really get the step in 15:28 where you equated the two differentials of the tilda function. I suppose this is because the tilda function uses the parabolic transformation, but previously we supposed the λ to be constant. Now thow λ is dependent in time.

[nobo]

In my humble opinion as a practitioner, this playlist (full 7 videos) includes some the most important tools for IR and FX derivatives pricing. Btw, in terms of modelling playlists (SVol and IR), SABR and Cheyette could also be useful options to be added. Brilliant piece of QF work for one more time!

[apoorvmishra]

can you show the same for stokes problem in fluid dynamics

[nicofish]

At 16:58 when you integrate dln(\bar{f}(z)) why do you integrate from 0 to z? Could you have integrated from any constant not equal to z to z? Your videos on diffusion are excellent. Thank you

[ahmedgharieb]

I want to ask is the (t) means summation of all time of the particle or just delta (t=t0_t1) for example.

[stephan]

Really great video again! Amazing work! I was following everything up until the last part (the integral representation). How would we go about solving the final integral to plot, for example, the distribution of particles for all x at some t given some initial condition psi(x)? Do we need to worry about z being dependent on x and t while we integrate (like if z was any variable we could take t and x out of the integral, but now z depends on t and x)? I know integral e^(-x^2) is the erf(x), but my calculus skills are quite rusty after many years of not using it a lot so not sure how to obtain the final answer. Thanks a lot for the great videos and sorry if my question is rather ignorant :)!

[ritobt]

A bit different take on solving the diffusion equation, but quite nice and clear explanation. Thank you!

[yuthapongpakam]

Thank you, I can get how to derive the diffusion equation with this method

[ricardoraimundo]

I must say great video, but the last minute was so rushed, I didnt really get how you went from ~f to f, where the integral came from and some other questions.