filmov
tv
Measure Theory, Functional Analysis, and The Lebesgue Integral for Undergraduates - Johnston

Показать описание
To support our channel, please like, comment, subscribe, share with friends, and use our affiliate links!
Don't forget to check out our patreon:
(Affiliate Link)
0:00 Intro (LOWER VOL) BEFORE NEXT CH)
0:16 Preface/Contents
0:54 Section 1.1 Uncountable Sets
3:12 Section 1.2 Measure Theory
5:21 Section 1.3 Step Functions
6:17 Section 1.4 Limits: Can This Book Substitute a Course on Real Analysis
7:53 1.5 L¹ Space
8:38 Chapter 2: Lebesgue's vs Riemann's Integral
9:22 Application: Fourier Series
9:58 Chapter 3: Function Spaces
10:48 Application: Quantum Mechanics
11:39 Measure Theory
11:52 Application: Probability
12:06 Chapter 12: Hilbert Space Operators
13:00 Index
13:28 Closing Comments
Thank you for supporting my channel!
Don't forget to check out our patreon:
(Affiliate Link)
0:00 Intro (LOWER VOL) BEFORE NEXT CH)
0:16 Preface/Contents
0:54 Section 1.1 Uncountable Sets
3:12 Section 1.2 Measure Theory
5:21 Section 1.3 Step Functions
6:17 Section 1.4 Limits: Can This Book Substitute a Course on Real Analysis
7:53 1.5 L¹ Space
8:38 Chapter 2: Lebesgue's vs Riemann's Integral
9:22 Application: Fourier Series
9:58 Chapter 3: Function Spaces
10:48 Application: Quantum Mechanics
11:39 Measure Theory
11:52 Application: Probability
12:06 Chapter 12: Hilbert Space Operators
13:00 Index
13:28 Closing Comments
Thank you for supporting my channel!
Measure Theory, Functional Analysis, and The Lebesgue Integral for Undergraduates - Johnston
The Best Book on Measure Theory
Introduction to Measure Theory and Functional Analysis
Real Analysis for Measure, Probability, and Functional Analysis - Ash
Measure Theory & Functional Analysis: Q&A Session
Measure Theory series to come…
Measure Theory & Functional Analysis 3: Introduction to Measures
Measure Theory & Functional Analysis 1: Real Analysis/Riemann Integration (Review)
Measure Theory
measure and integration theory #questions paper for M.sc
Measure Theory & Functional Analysis 7: Integration of Positive Functions
Functional Analysis #4: Measures
Measure Theory & Functional Analysis 10: Fubini-Tonelli Theorem
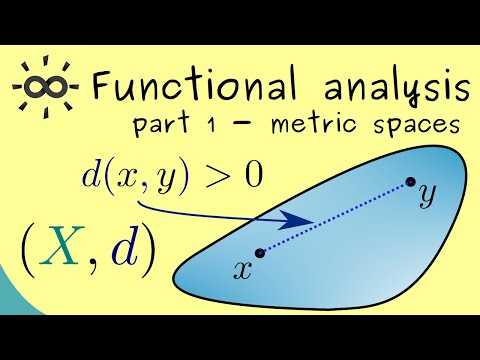
Functional Analysis 1 | Metric Space - How to Measure Distances?
Measure Theory & Functional Analysis 5: Borel Measures
Measure Theory & Functional Analysis 8: Riemann Integral vs Lebesgue Integral
Lecture 1: Basic Banach Space Theory
Measure Theory & Functional Analysis 9: Modes of Convergence
Real and Functional Analysis Part B Functional Analysis by Mukherjea and Pothoven #shorts
Lecture 8: Lebesgue Measurable Subsets and Measure
Measure Theory & Functional Analysis 6: Measurable Functions
pu affiliated colleges 2022 measure theory and lebesgue integration (416) past paper | #pastpapers
Functional Analysis Book
pu affiliated colleges 2021 measure theory and lebesgue integration (416) past paper | #pastpapers
Комментарии
 0:15:46
0:15:46
 0:01:46
0:01:46
 0:01:21
0:01:21
 0:12:51
0:12:51
 1:02:23
1:02:23
 0:21:51
0:21:51
 0:44:47
0:44:47
 1:07:28
1:07:28
 0:23:10
0:23:10
 0:00:16
0:00:16
 1:19:01
1:19:01
 0:30:55
0:30:55
 0:57:47
0:57:47
 0:05:59
0:05:59
 0:46:51
0:46:51
 0:40:14
0:40:14
 1:15:19
1:15:19
 0:50:41
0:50:41
 0:00:28
0:00:28
 1:14:02
1:14:02
 1:23:04
1:23:04
 0:00:12
0:00:12
 0:00:15
0:00:15
 0:00:11
0:00:11