filmov
tv
Lecture 1: Basic Banach Space Theory

Показать описание
MIT 18.102 Introduction to Functional Analysis, Spring 2021
Instructor: Dr. Casey Rodriguez
An introduction to Banach space theory, including vector spaces, norms and important examples of normed spaces.
License: Creative Commons BY-NC-SA
Instructor: Dr. Casey Rodriguez
An introduction to Banach space theory, including vector spaces, norms and important examples of normed spaces.
License: Creative Commons BY-NC-SA
Lecture 1: Basic Banach Space Theory
MAST30026 Lecture 18: Banach spaces (Part 1)
Banach Spaces- Lecture 01
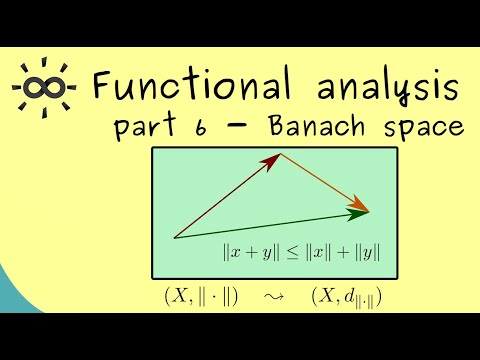
Functional Analysis 6 | Norms and Banach Spaces
Banach Spaces part 1
This chapter closes now, for the next one to begin. 🥂✨.#iitbombay #convocation
Why greatest Mathematicians are not trying to prove Riemann Hypothesis? || #short #terencetao #maths
Math-S401: Lecture II - Banach spaces
Lecture 21 (Part 1): Basic applications of Hahn-Banach Theorem
Joel FISH - 1/3 sc-Banach spaces and the sc-calculus
Normed, Banach and Hilbert Spaces: Everything You Need to Know! L^p Spaces and More 😉
BANACH SPACES PART-1 _PG TRB/ POLYTECHNIC
Lecture 8 (Part 1): A non example of Hilbert Space, Banach Space and example
Banach space lec2 sec 1
Normed and Banach Spaces Lec.-1
Cosplay by b.tech final year at IIT Kharagpur
Banach Spaces - Lec02 - Frederic Schuller
Hilbert Space in Quantum Mechanics | #shorts #viral #treanding #ytshorts #short #quantumphysics
Banach space definition with examples
Complete Metric Space | Banach Space in Mathematics | Banach Space Functional Analysis
Lecture 2, Addendum 1: Banach spaces
MAST30026 Lecture 1: What is space? (Part 1)
Functional Analysis: Weak convergence lecture 1 - Oxford Mathematics 3rd Year Student Lecture
Functional Analysis: Hahn-Banach Theorem - 1
Комментарии
 1:15:19
1:15:19
 0:49:55
0:49:55
 0:36:40
0:36:40
 0:07:57
0:07:57
 0:48:52
0:48:52
 0:00:16
0:00:16
 0:00:38
0:00:38
 1:07:16
1:07:16
 0:30:27
0:30:27
 1:11:50
1:11:50
 0:08:17
0:08:17
 0:09:09
0:09:09
 0:30:25
0:30:25
 0:21:00
0:21:00
 0:28:24
0:28:24
 0:00:15
0:00:15
 1:49:17
1:49:17
 0:00:06
0:00:06
 0:07:08
0:07:08
 0:10:06
0:10:06
 0:13:58
0:13:58
 0:46:29
0:46:29
 0:51:07
0:51:07
 0:39:39
0:39:39