Finding Distance Between Two Parallel Lines in 3D Space

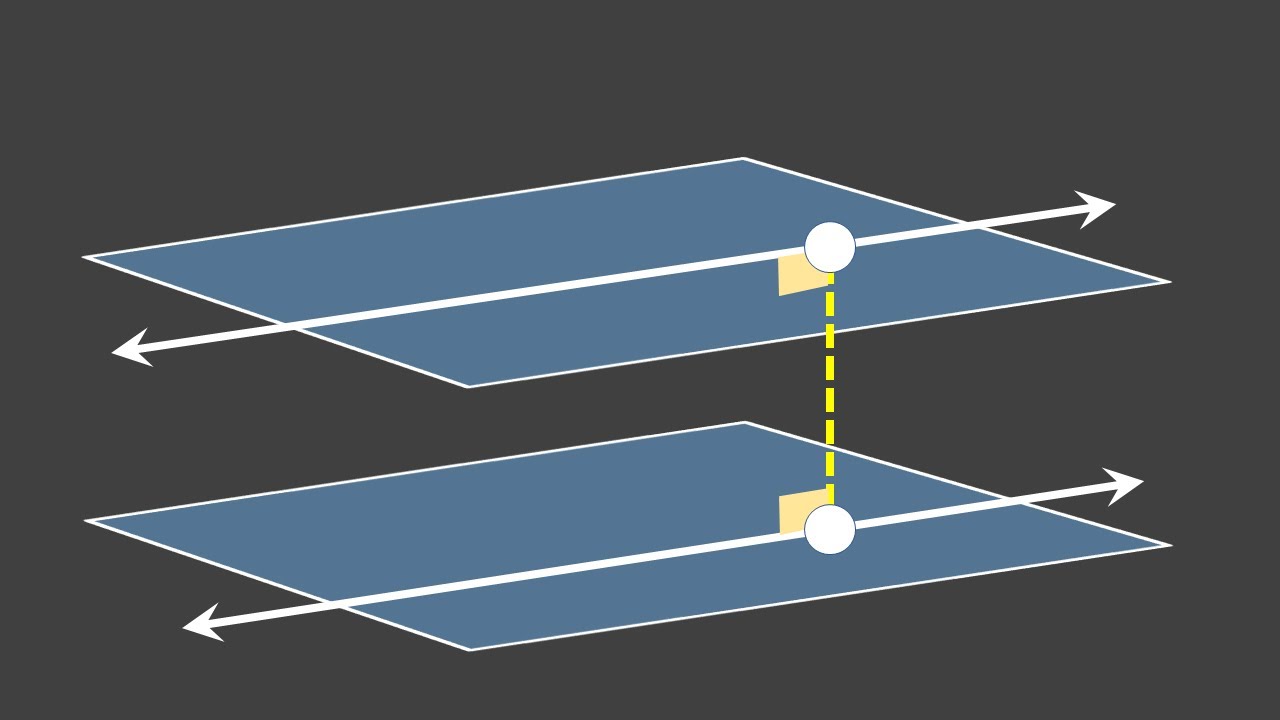
In this video, I am going to talk about “Finding the Distance Between Two Parallel lines in 3D Space”. Alternatively, you can say that “how to find distance between two lines in 3D space”. As you know distance between two intersecting or two coincident lines is always zero. But when we talk about non-intersecting lines in space; they might lie in parallel or non-parallel planes. Two given lines which lie in parallel planes may be skew or purely parallel to each other. We can calculate distance between two lines if they lie in parallel planes or coplanar. We will use properties of cross product of two vectors to find the distance between two parallel lines in 3d space. I have explained the concept through an example where two parallel lines are in symmetric form. But you can easily find distance between two parallel lines in 3d space if they are in parametric or vector form. Only thing is that you have to identify one point on each line and vectors to whom these lines are parallel. Two vectors are parallel if one is scalar product of other. Since, distance is non-negative quantity, you have to take magnitude of cross product of vector on both side of equation. This is the topic of class 12 mathematics three dimensional geometry.

[BrodieMitch]

I cannot tell if you are talking English or not