filmov
tv
Find the Limit of (x + x^3 + x^5)/(1 - x^2 + x^4) as x approaches Infinity
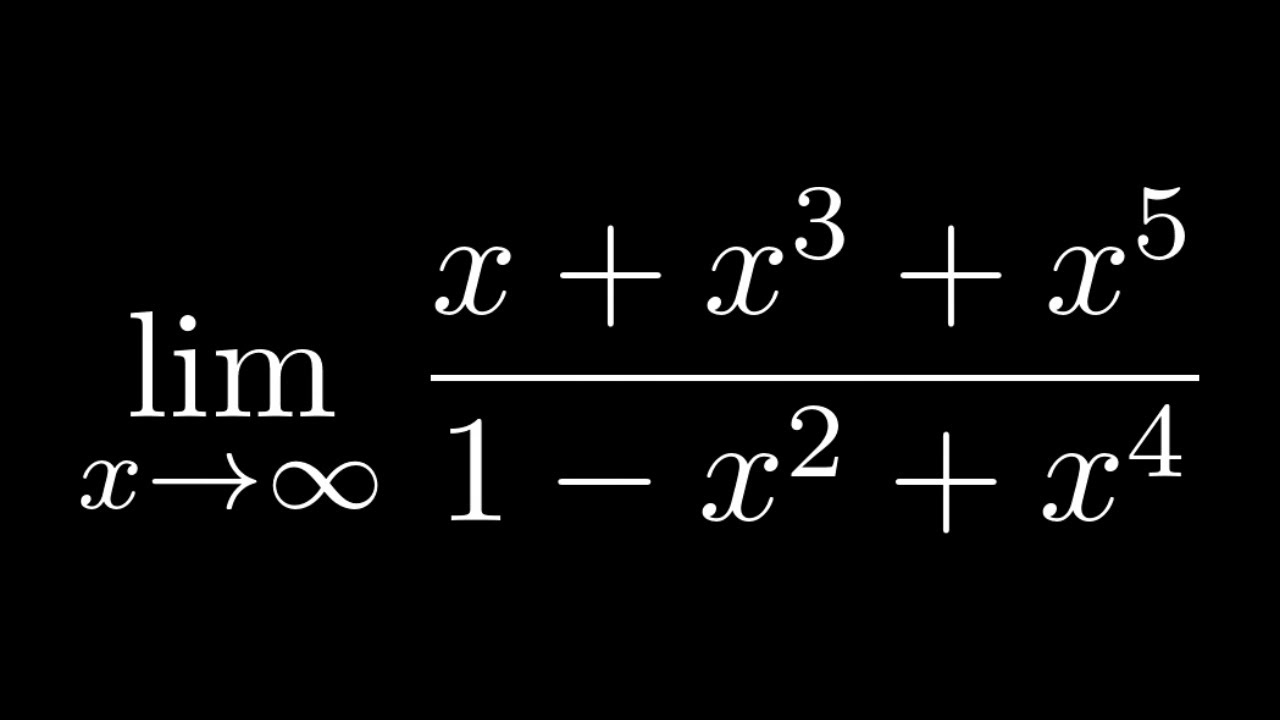
Показать описание
Find the Limit of (x + x^3 + x^5)/(1 - x^2 + x^4) as x approaches Infinity
If you enjoyed this video please consider liking, sharing, and subscribing.
There are several ways that you can help support my channel:)
************Udemy Courses(Please Use These Links If You Sign Up!)*************
Abstract Algebra Course
Advanced Calculus Course
Calculus 1 Course
Calculus 2 Course
Calculus 3 Course
Calculus 1 Lectures with Assignments and a Final Exam
Calculus Integration Insanity
Differential Equations Course
Differential Equations Lectures Course (Includes Assignments + Final Exam)
College Algebra Course
How to Write Proofs with Sets Course
How to Write Proofs with Functions Course
Trigonometry 1 Course
Trigonometry 2 Course
Statistics with StatCrunch Course
Math Graduate Programs, Applying, Advice, Motivation
Daily Devotionals for Motivation with The Math Sorcerer
Thank you:)
If you enjoyed this video please consider liking, sharing, and subscribing.
There are several ways that you can help support my channel:)
************Udemy Courses(Please Use These Links If You Sign Up!)*************
Abstract Algebra Course
Advanced Calculus Course
Calculus 1 Course
Calculus 2 Course
Calculus 3 Course
Calculus 1 Lectures with Assignments and a Final Exam
Calculus Integration Insanity
Differential Equations Course
Differential Equations Lectures Course (Includes Assignments + Final Exam)
College Algebra Course
How to Write Proofs with Sets Course
How to Write Proofs with Functions Course
Trigonometry 1 Course
Trigonometry 2 Course
Statistics with StatCrunch Course
Math Graduate Programs, Applying, Advice, Motivation
Daily Devotionals for Motivation with The Math Sorcerer
Thank you:)
How To Find The Limit At Infinity
How to Find Any Limit (NancyPi)
Evaluating Limits By Factoring
Limit of x/(x^2 + 7x) as x approaches 0
How to Find the Limit at Infinity (NancyPi)
Find the Limit of (2 - x)/(x - 1)^2 as x approaches 1
Find the Limit of (x^4 + x^5) as x Approaches Negative Infinity
Learn how to evaluate a limit at infinity
LIMITS AND DERIVATIVES in 1 Shot | Maths | 1st PUC
Find the Limit of (x + x^3 + x^5)/(1 - x^2 + x^4) as x approaches Infinity
Find the Limit of (x - sqrt(x)) as x approaches infinity
Find the limit of (x - 1)/(x^2(x + 2)) as x approaches negative 2 from the right
Find the Limit of (1 - x)/(x^2 - 1) as x approaches 1
Limit as x goes to negative infinity AGAIN!
Limit of the xth root of x as x approaches infinity
Find the limit as x approaches pi/2. Right sided limit for e^(tan x) Natural exponential function
How to Find the Limit of the Cube Root of x as x Approaches Infinity #shorts
How to Find the Limit of the Square Root of x as x approaches Infinity #shorts
Find the Limit of sqrt(x) as x Approaches Infinity
How To Tell If The Limit Exists
limit as x approaches infinity of x/sqrt(x^2+1)
Find the Limit of (1/x - 1/|x|) as x approaches 0 from the left
This is a very famous limit
Calculus Limits at Infinity The Limit of x/sqrt(x^2 - x) as x approaches negative infinity
Комментарии
 0:13:14
0:13:14
 0:16:42
0:16:42
 0:11:35
0:11:35
 0:01:49
0:01:49
 0:30:49
0:30:49
 0:02:18
0:02:18
 0:01:08
0:01:08
 0:01:40
0:01:40
 2:58:11
2:58:11
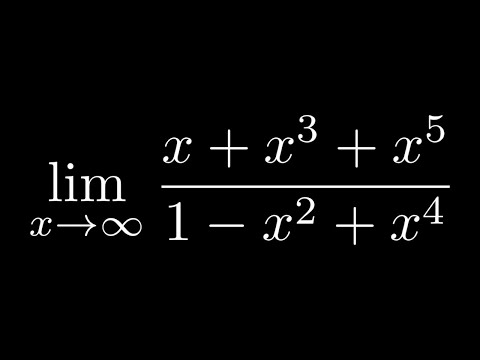 0:01:21
0:01:21
 0:03:08
0:03:08
 0:02:02
0:02:02
 0:01:43
0:01:43
 0:08:09
0:08:09
 0:08:58
0:08:58
 0:02:21
0:02:21
 0:00:25
0:00:25
 0:00:23
0:00:23
 0:01:13
0:01:13
 0:09:22
0:09:22
 0:00:48
0:00:48
 0:03:01
0:03:01
 0:00:48
0:00:48
 0:03:52
0:03:52