filmov
tv
Geometric Derivation of Centripetal Acceleration | Doc Physics
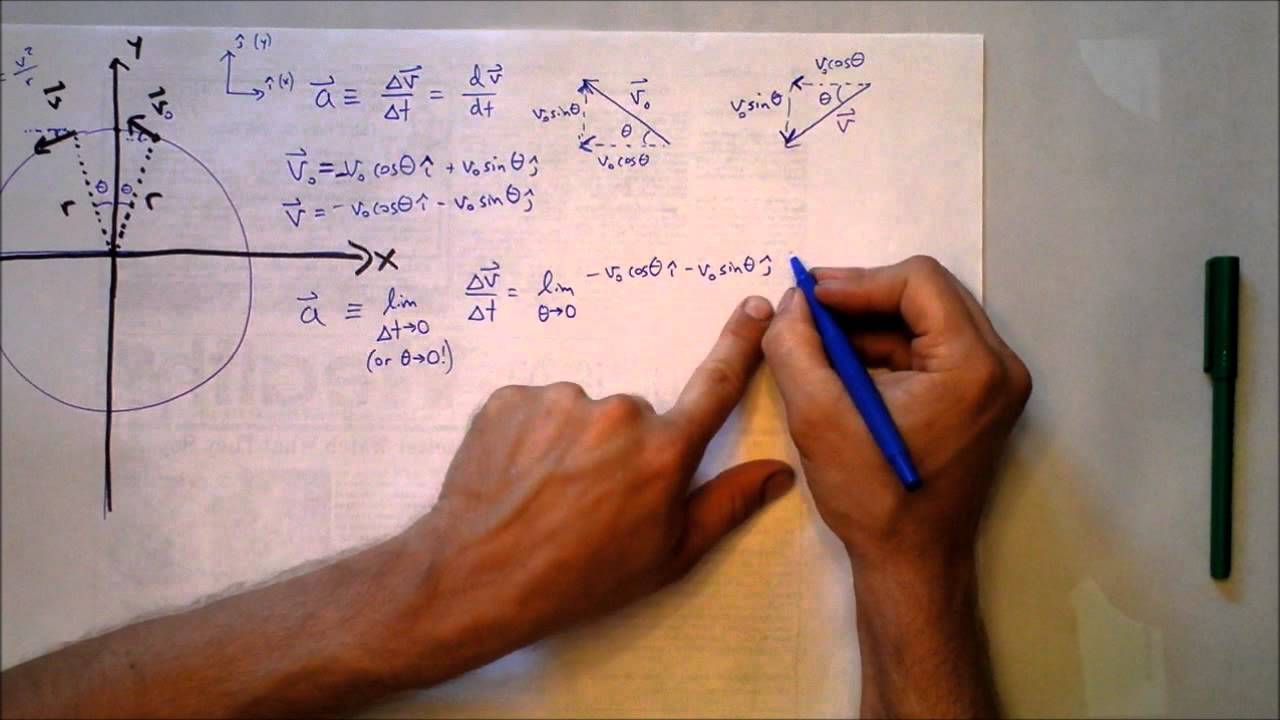
Показать описание
Maybe your teacher told you that a = v^2/r. Should you believe him?
Derivation of Formula for Centripetal Acceleration v^2/r
Geometric Derivation of Centripetal Acceleration | Doc Physics
Centripetal acceleration geometric derivation
Uniform Circular Motion - Geometrical Derivation of Centripetal Acceleration
Geometric Derivation of Centripetal Acceleration | Doc Physics
Two Simple Derivations of Centripetal Acceleration
Centripetal Acceleration: Formula Derivation - Geometrically(kind of)
Derivation of Centripetal Acceleration
Centripetal forces, question no-2. Edexcel past paper (WME03/01) 2022 June.
Centripetal Acceleration Using Geometric Method - Circular Motion - Physics Class 12
8.2 Circular Motion: Position and Velocity Vectors
9.2 Uniform Circular Motion: Direction of the Acceleration
Circular Motion: Derivation of Centripetal Acceleration
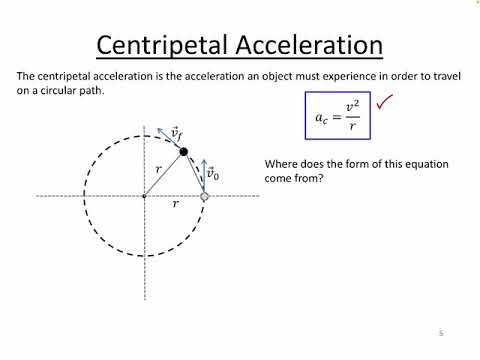
Visual understanding of centripetal acceleration formula | Physics | Khan Academy
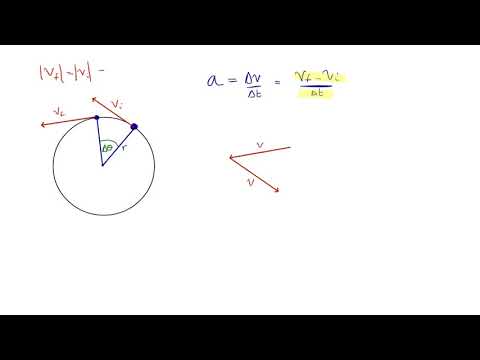
A Quick Derivation of Centripetal Acceleration
Uniform Circular Motion - Centripetal Acceleration Derivation
quick derivation of centripetal acceleration formula
Derivation of Formula for Centripetal Acceleration =v^2/r
Circular Motion for A level physics EXTRA - deriving the equation for centripetal acceleration.
1.5 Derivation of Centripetal Acceleration Formula
RM Video 2 Derivation of Centripetal Acceleration
Derivation of Centripetal Force
Grade 11 Physics - Complete Derivation of Centripetal Acceleration
Derivation of Centripetal Acceleration
Комментарии
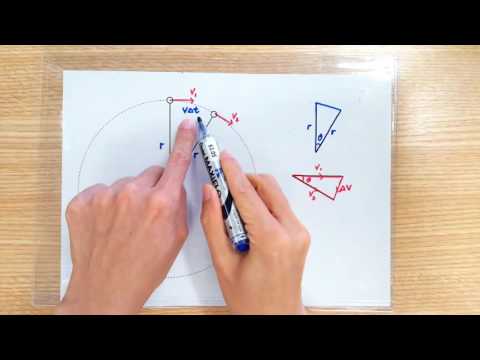 0:03:59
0:03:59
 0:12:30
0:12:30
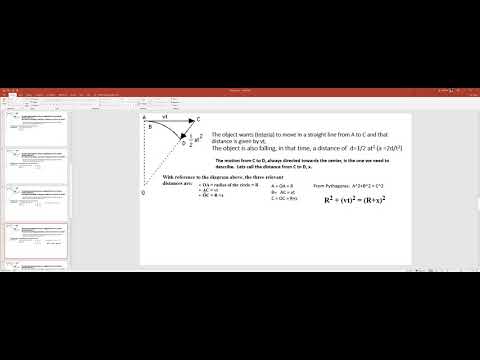 0:03:54
0:03:54
 0:17:57
0:17:57
 0:14:35
0:14:35
 0:22:52
0:22:52
 0:06:29
0:06:29
 0:03:16
0:03:16
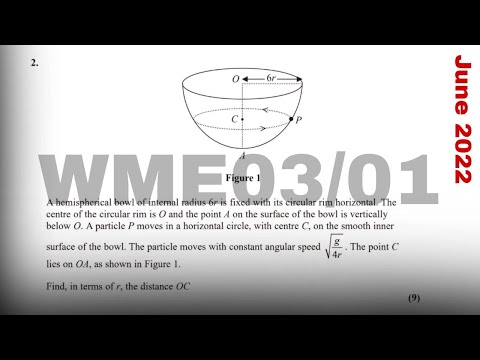 0:09:13
0:09:13
 0:20:00
0:20:00
 0:06:02
0:06:02
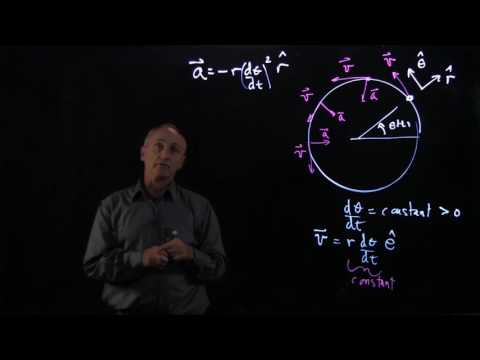 0:03:36
0:03:36
 0:08:44
0:08:44
 0:09:48
0:09:48
 0:07:13
0:07:13
 0:15:05
0:15:05
 0:04:54
0:04:54
 0:12:20
0:12:20
 0:09:57
0:09:57
 0:12:06
0:12:06
 0:04:26
0:04:26
 0:03:21
0:03:21
 0:18:38
0:18:38
 0:12:05
0:12:05