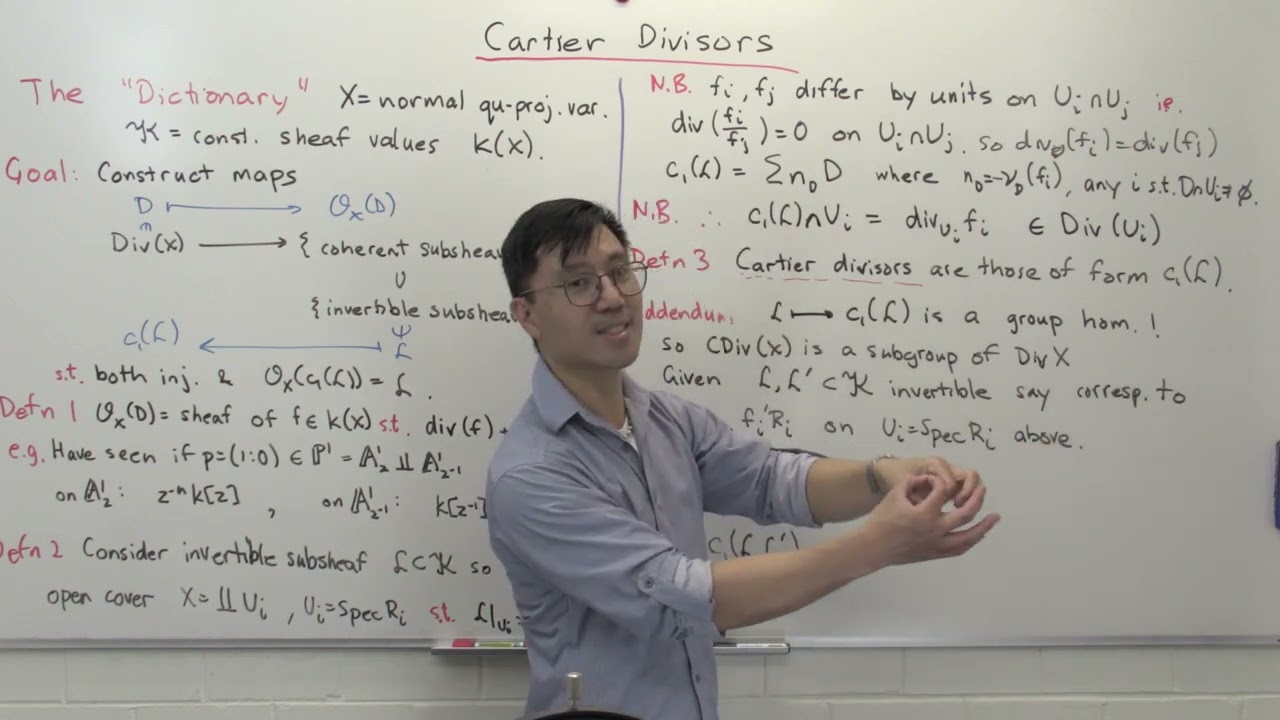
Cartier divisors

Показать описание

The most useful divisors in algebraic geometry are the Cartier divisors, because they are intimately related to invertible sheaves and their sections. In this video, we define the first Chern class of an invertible sheaf of rational functions, which is by definition a Cartier divisor. Conversely, given any divisor on a normal variety, we associate a sheaf of rational functions in an essentially inverse construction. Finally, we bring in sections of invertible sheaves and show how, modulo well-identified group, they correspond to certain effective linearly equivalent Cartier divisors. This means that to a large extent, we can view global sections of line bundles geometrically via their zeros, and there is very little loss of information in so doing.