filmov
tv
The Picard group of an algebraic variety

Показать описание
Invertible sheaves are important objects in algebraic geometry because their sections tell us how to map varieties into projective space. It thus becomes a natural question to ask what are all the invertible sheaves up to isomorphism. It turns out that the set of such isomorphism classes is a group called the Picard group. We introduce this group in this video. We show how to study it by relating it to the theory of Cartier divisors using the first Chern class map. We then compute the Picard group of projective space which also allows us to enunciate all the line bundles on it. Finally, we introduce the degree of a divisor or invertible sheaf on a smooth projective curve. This is a very important invariant which helps us to study both divisors, invertible sheaves and the Picard group.
The Picard group of an algebraic variety
Jason Parker – Polymorphic automorphisms and the Picard group
Line Bundles to Picard Group on Projective Space
Picard Group of Dedekind Domain
Polymorphic Automorphisms and the Picard Group
The Picard group of the stable module category of a finite group - Jesper Grodal
[8. Introduction to Fibrations] Picard group and tautological bundle
Schemes 38: Comparison of Cartier divisors and Pic
Irina Bobkova - Picard group of the K(2)-local category at the prime 2
Meet Nic Walts of The Picard Group
A guided tour to the Picard group of the stable module category - Jesper Grodal (Copenhagen)
Local Picard groups and descent of line bundles -- János Kollár (Princeton University)
Elliptic Curves - Lecture 9b - The (Picard) group law
Vesna Stojanoska : Representation spheres and chromatic Picard groups
Serre Twisting Sheaf and Picard Group
Tensor product of Ox modules, locally free sheaves and Picard group
Isomorphism of Picard Groups and Ideal Class Groups
The group law on the cubic curve and the Jacobian variety
Schemes 40: Examples of PicX
Agnes Beaudry: K(n)-local Picard Groups and Gross-Hopkins Duality
Richard Wong Research Summary 2020
Meet Nic Walts of The Picard Group
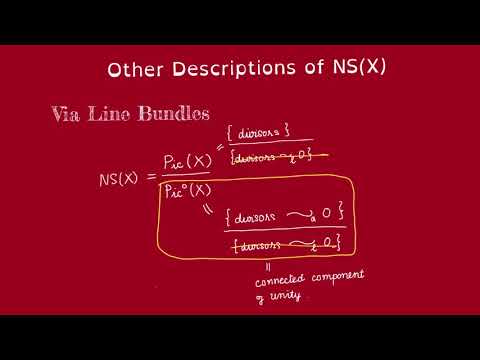
Neron Severi Group vs. Picard Group
Anya Nordskova: Derived Picard groups of representation-finite symmetric algebras (17/11/2022)
Комментарии
 0:22:29
0:22:29
 0:39:35
0:39:35
 0:09:17
0:09:17
 0:11:19
0:11:19
 0:27:18
0:27:18
 1:17:27
1:17:27
 1:14:17
1:14:17
 0:25:11
0:25:11
 0:56:46
0:56:46
 0:02:44
0:02:44
 1:10:23
1:10:23
 1:01:06
1:01:06
 0:34:53
0:34:53
 0:59:05
0:59:05
 0:12:48
0:12:48
 0:12:30
0:12:30
 0:11:11
0:11:11
 0:17:51
0:17:51
 0:24:25
0:24:25
 0:49:59
0:49:59
 0:07:59
0:07:59
 0:02:44
0:02:44
 0:02:29
0:02:29
 1:00:58
1:00:58