Direct Comparison Test - Calculus 2

Показать описание

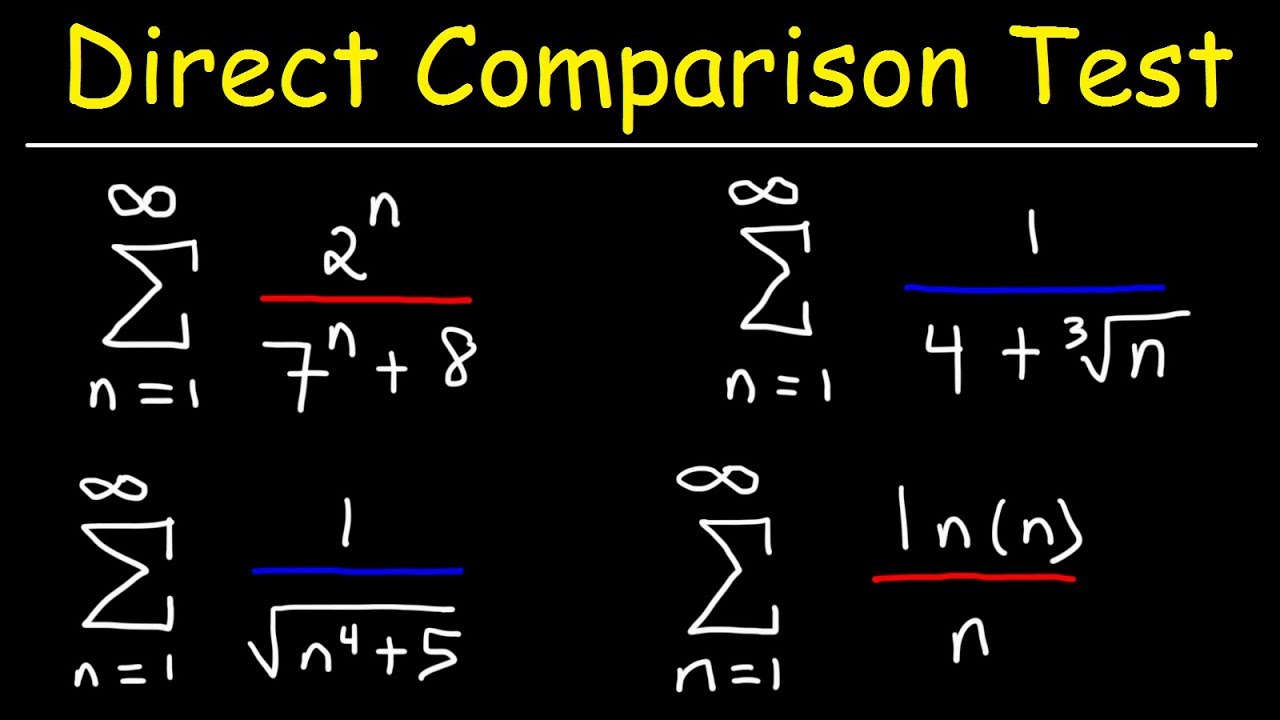
This calculus 2 video tutorial provides a basic introduction into the direct comparison test. If the big series converges, then the smaller series must also converge. Likewise, if the small series diverges, the big series must diverge as well. That's the basic idea of the direct comparison test. Typically, you need to compare the series you're analyzing with a simpler p-series or geometric series to tell if the original series will converge or diverge.

Integral Test For Divergence:

Remainder Estimate - Integral Test:

P-Series:

Direct Comparison Test:

Limit Comparison Test:

_________________________________
Alternating Series Test:

Alternate Series Estimation Theorem:

Absolute & Conditional Convergence:

The Ratio Test:

The Root Test:

__________________________________
Series Tests - Practice Problems:

Taylor & Maclaurin Polynomials:

Taylor's Remainder Theorem:

Power Series - Interval Convergence:

Power Series - Derivatives & Integrals:

___________________________________
Calculus Final Exam and Video Playlists:

Full-Length Videos and Worksheets:

Рекомендации по теме

Комментарии

My man is carrying the entirety of the next generation's higher education on his shoulders

joshuaway

I cannot thank you enough! I'm a full time college student living out on his own in an apartment so I work nights from 10PM-6AM five times a week and sometimes miss out on info during lectures. This section has been brutal to me.

aunpaidintern

An analogy.

You are driving home in your car with a friend. The car is low on gas, the low gas light comes on.

Your friend lives a few miles before your house, on the way, less distance.

If you make it home (converge) of course so did your friend.

But if your friend makes it home, that doesn't mean you won't run out of gas on the way to your house (diverge).

Same situation here.

rmdir

you and patrickjmt are the people i pray to thank you everynight

juanls

Do you realize what a huge help you are to so many people!? You deserve more attention! Thanks for all the videos you make you are my favorite youtube instructor of all time hands down!

Oscar-gxyf

Hi! Just wanted to let you know that your channel has been extremely helpful recently!! My calculus teacher passed away in January, and she was one of the best teachers I’ve ever had. I know she would have wanted me to continue working hard in her class, so I’m doing the best I can with your videos and a new teacher. Thanks for all you do!!!

claireeckhardt

that graph visual really helped me understand things so much clearer! thank you!!!

terrancepage

You are just awesome. Consistently through the past year, whenever I've struggled to understand a concept or technique, your videos make it click.

darwinorsler

I can't believe I only now found your videos. They are SO helpful, and make these problems so much easier to understand. Thank you!!!!

RamytRamyt

you're a life saver, thank you so much 😭❤

michellemathew_ximb

I don't even have to go to class, I just watch your videos before my quizzes. Good stuff man

slowpr

I can always count on this channel for the 2am next day exam cram

elguis

The problem at 11:50ish is true guys because remember we have been given a restriction if n is greater than or equal to 3. So if you were to plug in 3 you can actually see that 1/n is smaller.

Also keep in mind that you need to simplify for the second to last problem n/n^2 becomes 1/n

pfeliciano

Thank you for doing these!
If you can, please do one on Taylor and Maclaurin series & Power series.
Exam Monday and you’ve helped me ace everything

helpontheway