filmov
tv
JEE Delight | Geometry of Complex numbers | Lecture 7 | Triangle in a Circle | Selected #7 SE
Показать описание
JEE Delight | Geometry of Complex numbers | Lecture 7 | Triangle in a Circle | Selected #7 SE
00:00 SE#1: A, B and C are the points representing the complex numbers z1, z2 and z3 respectively on the complex plane and the circumcenter of the ABC lies at the origin. If the altitude AD meets the circumcircle again at P, then P represents the complex number
02:39 SE#2: P is a point on the Argand diagram. On the circle OP as diameter two points Q and R are taken such that POQ=QOR. If O is the origin and P, Q, R are represented by z1, z2 and z3 respectively then z2^2cos2q=z1z3cos^2q
05:45 SE#3: where p, q and r are the moduli of non-zero complex numbers u, v and w respectively, prove that arg(w/v)=arg(w-u/v-u)^2
08:28 SE#4: If z lies on the circle centered at origin. If area of the triangle whose vertices are z, wz and z+wz, where w is w is cube root of unity, is 4√3. Then radius of the circle is
10:54 SE#5: Let z1, z2 and z3 be three distinct complex numbers lying on a circle with center at origin such that z1+z2z3, z2+z3z1 and z3+z1z2 are purely real, then z1z2z3 is
13:19 SE#6: Let ABC be a triangle whose circumcenter coincides with the unit circle. Then the circumcenter, centroid and orthocenter are 0, a+b+c/3 and a+b+c respectively
14:34 SE#7: Let Ak (k=1,2,3...n) be the vertices of a regular n-gon inscribed in a unit circle then prove that product |A1Ak|=n Support the channel:
00:00 SE#1: A, B and C are the points representing the complex numbers z1, z2 and z3 respectively on the complex plane and the circumcenter of the ABC lies at the origin. If the altitude AD meets the circumcircle again at P, then P represents the complex number
02:39 SE#2: P is a point on the Argand diagram. On the circle OP as diameter two points Q and R are taken such that POQ=QOR. If O is the origin and P, Q, R are represented by z1, z2 and z3 respectively then z2^2cos2q=z1z3cos^2q
05:45 SE#3: where p, q and r are the moduli of non-zero complex numbers u, v and w respectively, prove that arg(w/v)=arg(w-u/v-u)^2
08:28 SE#4: If z lies on the circle centered at origin. If area of the triangle whose vertices are z, wz and z+wz, where w is w is cube root of unity, is 4√3. Then radius of the circle is
10:54 SE#5: Let z1, z2 and z3 be three distinct complex numbers lying on a circle with center at origin such that z1+z2z3, z2+z3z1 and z3+z1z2 are purely real, then z1z2z3 is
13:19 SE#6: Let ABC be a triangle whose circumcenter coincides with the unit circle. Then the circumcenter, centroid and orthocenter are 0, a+b+c/3 and a+b+c respectively
14:34 SE#7: Let Ak (k=1,2,3...n) be the vertices of a regular n-gon inscribed in a unit circle then prove that product |A1Ak|=n Support the channel:
Комментарии
 0:04:42
0:04:42
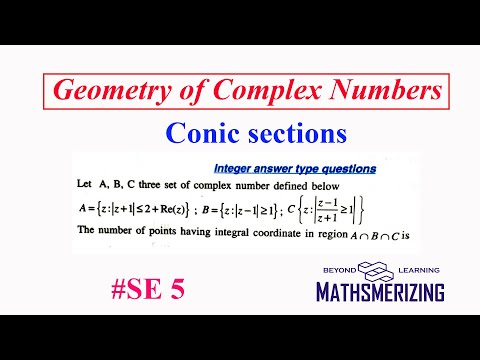 0:03:45
0:03:45
 0:04:08
0:04:08
 0:06:53
0:06:53
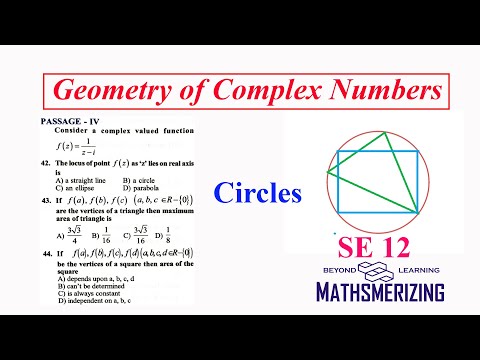 0:03:41
0:03:41
 0:12:07
0:12:07
 0:07:09
0:07:09
 0:09:30
0:09:30
 0:12:03
0:12:03
 0:05:37
0:05:37
 0:10:15
0:10:15
 0:05:18
0:05:18
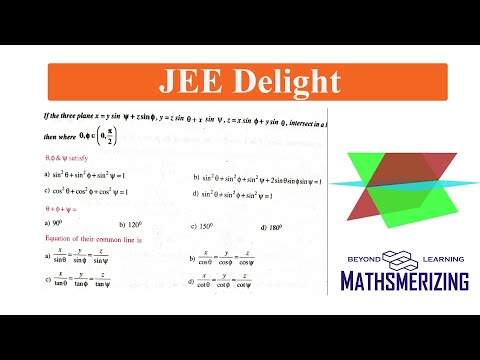 0:08:28
0:08:28
 0:11:40
0:11:40
 0:08:11
0:08:11
 0:04:46
0:04:46
 0:07:59
0:07:59
 0:07:39
0:07:39
 0:15:12
0:15:12
 0:17:36
0:17:36
 0:02:25
0:02:25
 0:05:49
0:05:49
 0:04:55
0:04:55
 0:08:46
0:08:46