JEE Delight | Geometry of complex number | Circles | #5 Selected solved examples | Timestamp details

JEE Delight | Geometry of complex number | Circles | #5 Selected solved examples | Timestamp details in description

00:00 #SE1: Find the equation of circle in complex form which touches the line iz+z+1+i=0 and the lines (2-i)z=(2+i)zbar and (2+i)z+(i-2)zbar-4i=0 are normals to the circle

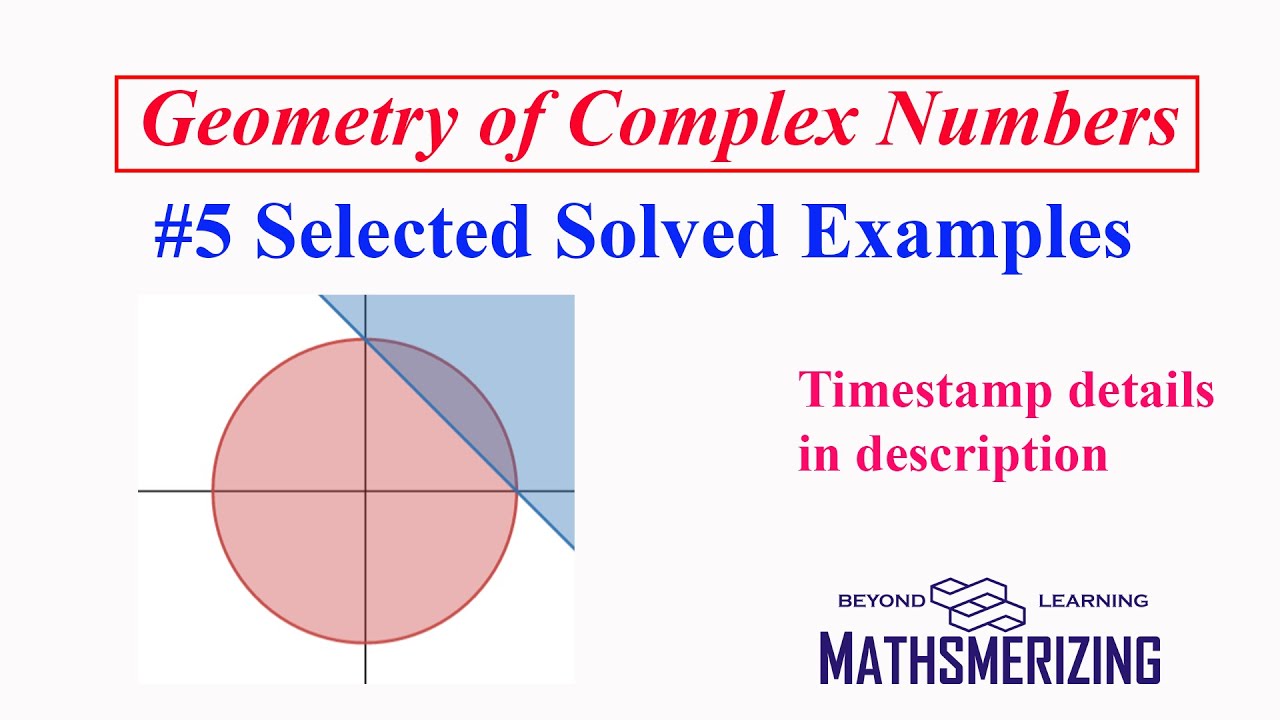
03:43 #SE2: Let A and B be sets defined by |z| less than or equal to 2 and (1-i)z+(1+i)zbar greater than equal to 4 find the area bounded by the region

05:56 #SE3: Let z be a complex number satisfying the equality |z-3i|=3, then cotq-6/z equals?

07:54 #SE4 : If one of the vertices of a square circumscribing the circle |z-1|=√2 is 2+3i, find the other vertices

10:05 #SE5 : If the complex number P(w) lies on a standard unit circle and z=(aw+b)(w-c)^-1 then find the locus of z and interpret it.

Support the channel: