filmov
tv
Solve & Check this Exponential Equation | 4^(x+2) + 4^(x+5) = 65

Показать описание
Solve this Exponential Question and Check Your Answer | Step-by-Step Tutorial
Solve and Check | Learn how to solve exponential equation quickly | Math Olympiad Training
Exponential Equation | Try solve this and check the solution | Good thinking 🤔
Olympiad Mathematics | Solve and Check Exponential Equation (x/8)^x=(8^8)^8 | Math Olympiad Training
Solve & Check this Exponential Equation | 4^(x+2) + 4^(x+5) = 65
Olympiad Mathematics | Solve and Check Exponential Equation | Math Olympiad Training
Tips To Solve Exponential equation | Find k | Solve And Check
Solving Exponential and Logarithmic Equations
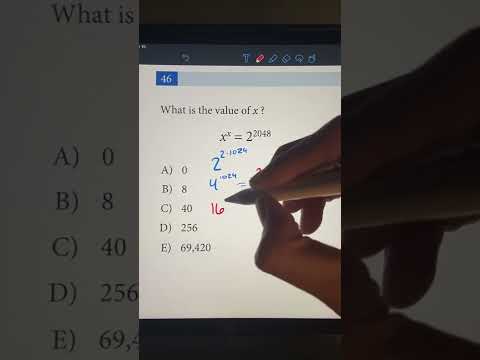
J.E.D.I. Training MATH for DIGITAL SAT - Day 6 of 7 (37 Digital SAT Math Problems EXPLAINED)
Solving Exponential Equations
Solving an exponential equation
Hard Math Problem with Exponents | Trick Question | JusticeTheTutor #shorts #mathematics #maths
A Nice Olympiad Exponential Multiplication Problem #short #olympiad #mathematics #maths #exponents
Finding a formula for an exponential function from a table
Exponential Equations - Algebra and Precalculus
Check this nice solution for Exponential Equation.
USA Olympiad Exponential Equation: Solve for X | Check out the TRICK!!
Can You Solve this Exponential Equation? | Easy Step-by-Step Tutorial
Exponential Search - better than Binary search? (Explained)
A Nice USA Olympiad Exponential Equation: Check the Method!!!
A Nice Exponential Equation | Check the two Methods !!
A Nice Exponential Equation| Check the TRICK!! |X=?
How to Compare Two Very Large Exponents?
How to calculate exponential power ( like e^x ) in scientific calculator. #scientific #calculator
Комментарии
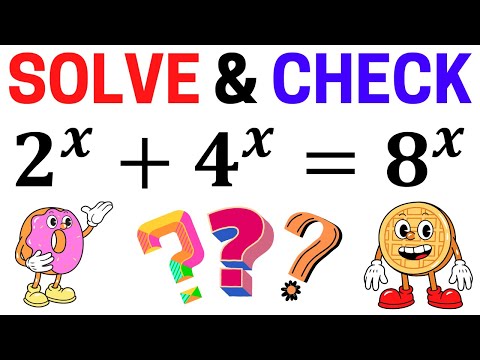 0:09:42
0:09:42
 0:08:08
0:08:08
 0:05:01
0:05:01
 0:08:09
0:08:09
 0:05:19
0:05:19
 0:04:56
0:04:56
 0:03:35
0:03:35
 0:07:08
0:07:08
 1:04:45
1:04:45
 0:16:36
0:16:36
 0:03:14
0:03:14
 0:00:57
0:00:57
 0:00:52
0:00:52
 0:04:13
0:04:13
 0:05:58
0:05:58
 0:03:06
0:03:06
 0:08:18
0:08:18
 0:09:29
0:09:29
 0:11:23
0:11:23
 0:04:03
0:04:03
 0:04:03
0:04:03
 0:05:33
0:05:33
 0:05:38
0:05:38
 0:01:01
0:01:01