filmov
tv
Sketching a Function Based on a Derivative Graph, Example 2
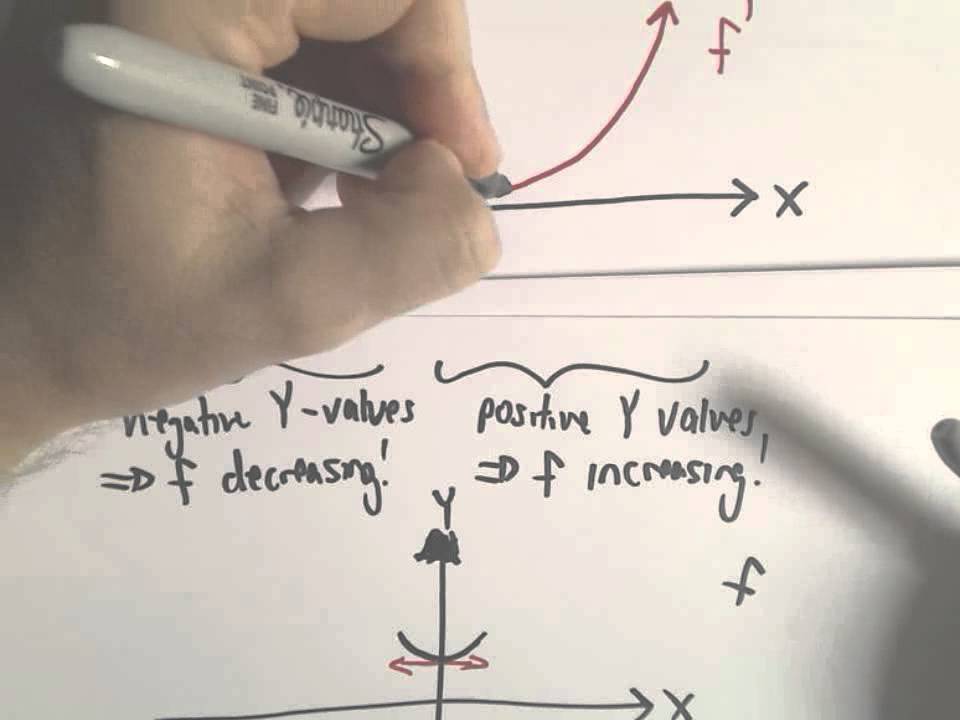
Показать описание
Sketching a Function Based On Its Properties
Sketching a Function Based on a Derivative Graph, Example 1
Sketching a Derivative from the Graph of a Function
Given fx sketch the first and second derivative graph
Sketching a Function Based on a Derivative Graph, Example 2
Calculus AB/BC – 5.8 Sketching Graphs of Functions and Their Derivatives
Calc I: Sketching a graph of a function given some properties (medium)
Draw the Function given Graph of Derivative
Solving a #System of #Equations: Linear and Quadratic #Equation Using Vieta’s #Theorem | #PSAT #Math...
Graphing Functions and Their Derivatives
Sketching a graph of a function
Sketching the Derivative of a Function
❖ Sketching the Derivative of a Function ❖
Domain and Range of a Function From a Graph
Sketching the Graph of a Function with Infinite Limits
Graphing Higher-Degree Polynomials: The Leading Coefficient Test and Finding Zeros
Sketching a Function from its derivative
Sketching a function based on the derivative information given.
Calculus--Sketching a Function from its Derivative Part 1
Sketching the derivative function from a picture
How to sketch level curves
Graphing Rational Functions and Their Asymptotes
Visualizing Multi-variable Functions with Contour Plots
Sketching Anti-Derivative Function
Комментарии
 0:05:13
0:05:13
 0:07:24
0:07:24
 0:08:44
0:08:44
 0:04:09
0:04:09
 0:06:06
0:06:06
 0:11:42
0:11:42
 0:05:32
0:05:32
 0:07:39
0:07:39
 0:00:37
0:00:37
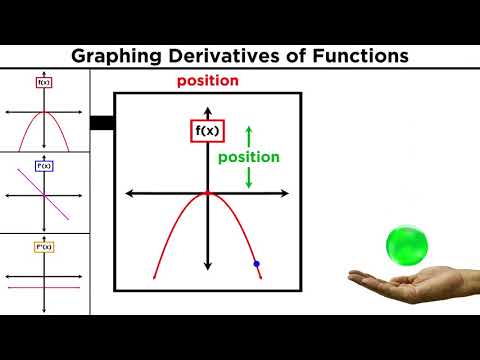 0:13:06
0:13:06
 0:05:05
0:05:05
 0:11:31
0:11:31
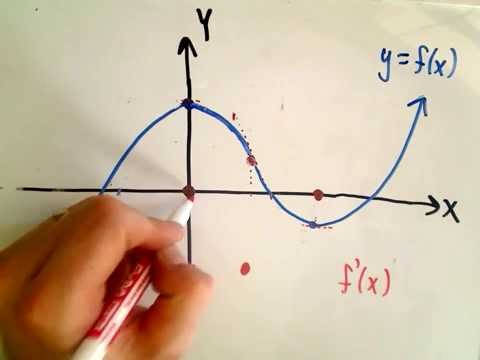 0:14:49
0:14:49
 0:13:24
0:13:24
 0:06:37
0:06:37
 0:09:15
0:09:15
 0:14:42
0:14:42
 0:04:03
0:04:03
 0:14:56
0:14:56
 0:17:40
0:17:40
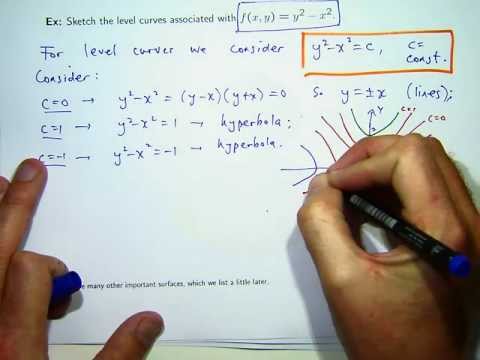 0:09:22
0:09:22
 0:07:24
0:07:24
 0:07:54
0:07:54
 0:16:17
0:16:17