Graphing Functions and Their Derivatives

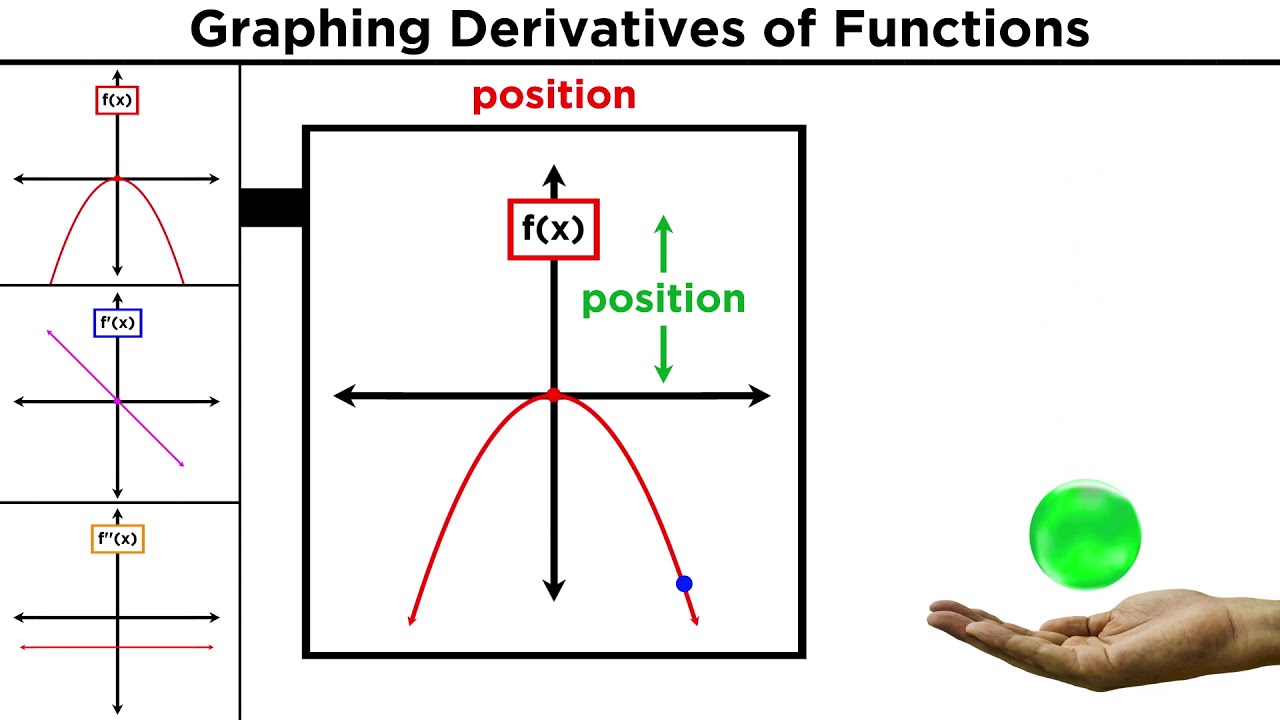
We know how to graph functions, and we know how to take derivatives, so let's graph some derivatives! Many students find that this hurts their brain, but it's just about practice! Remember that the value of a function and the rate of change of that function at a particular point are completely unrelated! A function may be positive but have a negative rate of change, or vice versa. Let's learn how to graph derivatives intuitively, and then some applications of this in physics!

Check out "Is This Wi-Fi Organic?", my book on disarming pseudoscience!

[Thaumius]

I learned concavity by thinking of faces. If the second derivative is +, happy face (concave upwards) If the second derivative is - sad face or frown (concave downwards)

[rafurafu]

i love the pop quiz after the video. makes me feel 20 IQ points smarter! thanks

[wanjirurandolph]

i love the way this was explained having real world examples like the ball makes this easier to remember

[critz]

Learned more here, than school. Thanks.

[NilEoe]

It's impressive how a few youtubers are doing so much good in helping thousand (if not more) students around the world thanks to how much better they are at explaining these concepts (plus, the video format is in my opinion better than a traditionnal lecture for this, in fairness to uni lecturers). You have a great outcome on the world :)

[DarinBrownSJDCMath]

7:52 Second derivative = 0 does not imply inflection point. But if the graph of the second derivative crosses the x-axis, then you will have an inflection point. You did mention that later, but the change of sign in f'' is necessary.

[ArfatXeon]

"Painful mental gymnastics..." lol

[carloselfrancos]

Thanks for your videos ^^
You've done great work :D
And I EVENTUALLY understood derivation xD (yay)

[juggrnauthd]

on the first example how is the derivative positive at the top left of the Cartesian plane if it descends and its in the negative coordinates...

[DarinBrownSJDCMath]

10:45 Whoa there, not every cubic function is odd!! In fact, this function f(x) = x^3 - 12x + 1 is not an odd function. I think you mean to say it has odd degree which does determine its end behavior.

[josephshaff]

Thanks Dave. . lol Book says if f crosses x-axis it makes an I.P. on f". I see they labeled the T.P.s of f as I.P.s on f'. Then they said where f crosses x axis those are I.P.s on f" lol I'll be ok. Did the section 2x going for 3rd but now I have to make my case. It's only this one problem it always looked weird. Thanks for the vid cleared things up.

[atp]

Thank you so much for explaining this step by step; I can never keep up with my teacher because he’s always referencing things rather than explaining them

[MiltosPol-qnzh]

Professor, whats the difference between f(x)=x^2 and y=x^2???

[ericfricke]

Thank you for your CONCISE explanations!

[annyan]

i must say hats offf am starting to love calculus

[NoActuallyGo-KCUF-Yourself]

Shouldn't there be a second-derivative test or other to confirm change in concavity before assuming an inflection point?
For example, d²/dx² of x⁴ is 0 at x=0, but there is no inflection there.

[giuseppecalvi]

Hi Dave, very nice video!! Many thanks for posting it! However, please note that (x^3 - 12* x +1) is NOT an ODD function.

[ejsafara]

hi dave ^^ i mainly know you from debates, but holly hell ur good at explaining math too! :D

[KaranGupta-pp]

Prof. Dave I would suggest making a position graph when we consider the x-axis as time totally in positive x-axis as it seems really hard to correlate time in a negative direction even if it's a position graph, I would suggest shifting this graph totally into a positive x direction.

[ArnavGarg-rlqh]

i was struggling with physics graph and now i finally understand it 🤯🤯🤯 thank you so much