filmov
tv
Math Olympiad Question | Compare 100^99 and 99^100 which is larger?
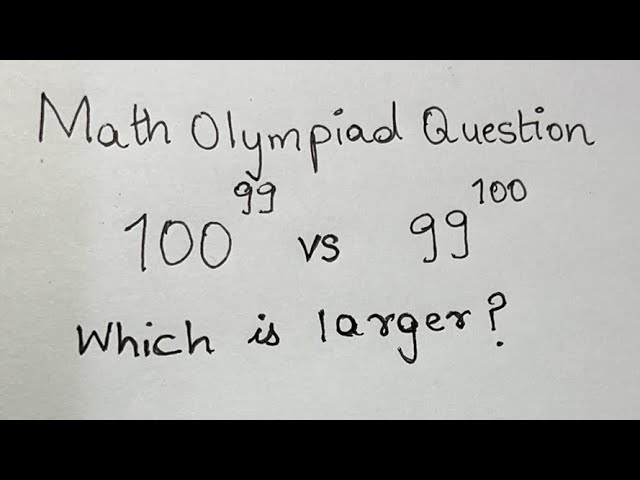
Показать описание
Math Olympiad Question | You should know this trick!!
A Nice Olympiad Exponential Multiplication Problem #short #olympiad #mathematics #maths #exponents
A Nice Math Olympiad Exponential Equation 3^x = X^9
Norway Math Olympiad Question | You should be able to solve this!
Solve this difficult math Olympiad problem
The unexpectedly hard windmill question (2011 IMO, Q2)
Japanese Math Olympiad Problem | A Nice Math Problem : Comparison
Math Olympiad Question | Equation solving | You should learn this trick to pass the exam
Answer Key Set C IMO 2024-25/Class 9 Maths Olympiad Exam/12th Dec 2024
Math Problem Test your IQ 🧠 #olympiad #maths #jee #iitjee
Math Olympiad question | Trending shorts || #shorts #youtubeshorts #short
France - Math Olympiad Question | An Algebraic Expression | You should be able to solve this!
New Zealand - Math olympiad Question
60 years ago this question was on the International Mathematical Olympiad
Trending maths olympiad questions #mathspuzzles #olympiad #algebra #viral #shorts
International Olympiad Exam (IMO) Enters the Chat ☠️ #PW #Shorts #Exams
A Nice Math Olympiad Algebraic Expansions Problem. #math #olympiad #mathematics #matholympiad #viral
A beautiful international math olympiad problem
Luxembourg - Math Olympiad Question | You should know this trick
Solving the hardest question of a British Mathematical Olympiad
Germany - Math Olympiad Question
Math Olympiad Practice
Germany - Math Olympiad Question | The BEST Trick
Math Olympiad 3^m–2^m=65 | Math Olympiad Problems | Algebra
Комментарии
 0:00:33
0:00:33
 0:00:52
0:00:52
 0:02:34
0:02:34
 0:03:21
0:03:21
 0:00:10
0:00:10
 0:16:03
0:16:03
 0:04:15
0:04:15
 0:01:54
0:01:54
 0:05:21
0:05:21
 0:00:32
0:00:32
 0:00:15
0:00:15
 0:08:40
0:08:40
 0:01:12
0:01:12
 0:09:10
0:09:10
 0:00:05
0:00:05
 0:00:16
0:00:16
 0:00:51
0:00:51
 0:01:00
0:01:00
 0:02:51
0:02:51
 0:11:26
0:11:26
 0:11:00
0:11:00
 0:01:00
0:01:00
 0:10:37
0:10:37
 0:10:49
0:10:49