Trigonometric Substitution for Algebra Proof | Trigonometry | Trigonometric Functions

Trigonometric Substitution used for algebra proof is shown in this video.

Trigonometric Substitution will make it possible for Algebra Proof cause
we can use the Properties of Trigonometric Functions to solve the proof.

Sin squared plus cos squared equals 1 is an important property of Trigonometric Functions.We will use this property to simplify the proof.

---------------------------------------------------------------------------------------------------------------------------

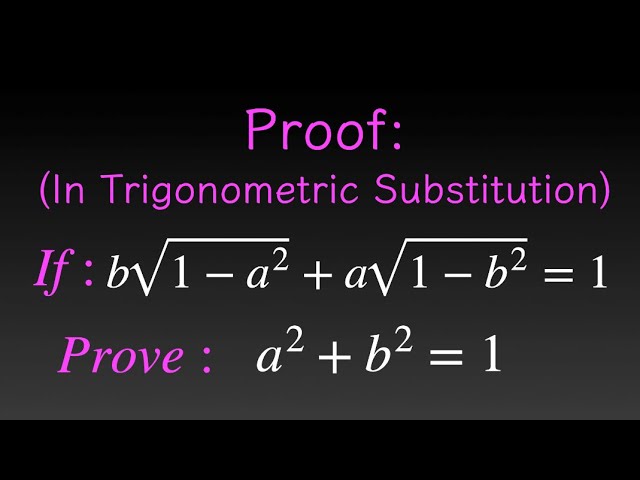
The algebra proof is : a^2 + b^2 =1 if we know : b sqrt(1-a^2) + a sqrt(1-b^2)=1.

There are sqrt(1-a^2) and sqrt(1-b^2) in the expression, so there must be 1-a^2 ≥0 and1-b^2 ≥0. So there must be |a|≤1 and |b|≤1.
Recall sin squared plus cos squared equals 1, and |sin|≤1, |cos|≤1, so it’s possible to use Trigonometric Substitution .

---------------------------------------------------------------------------------------------------------------------------

The algebra proof is solved by using Trigonometric Substitution in this video. It will be possible for us to solve some difficlute algebra problems if we can use Trigonometry Knowledge with flexibility.

#Trigonometry #Trigonometric Substitution #Trigonometric functions

[schematism]

Very wonderful and linear demonstration, thank you.
I only ask you if you put the condition of angle between zero and pi, in order to have only positive values for the absolute value of sin function. Immediately, I would have put the entire circonference as dominion, but this would have implied some negative values for sin, which would have been superfluous, maybe. Thank you very much!

[ramvaidy]

Nice solution, but I dont understand one thing; why can't you just sub in a = sin theta and b = cos theta, that would make the answer much simpler, no?

[ZOCAandK]

Use Cauchy's inequality can prove it as well

[barryseok]

a=square root of (1/2), b= square root of (1/2) so a**2 + b**2 = 1, therer is no need to use sine & cosine to prove ...

[beautifulmindinpuzzles]

Alternately by algebra :
1) Square both sides
2) Isolate the radical
3) Let
1 - a^2 - b^2 + a^2 b^2 = u^2
Sub in: 2abu = u^2 + a^2 b^2
4) Solve for u: u = ab
5) Sub in (3) to get
a^2 + b^2 =1
Nice problem. Thanks!