filmov
tv
Measure Theory 15 | Image measure and substitution rule

Показать описание
Please consider to support me if this video was helpful such that I can continue to produce them :)
🙏 Thanks to all supporters! They are mentioned in the credits of the video :)
This is part 15 of 22 videos.
#MeasureTheory
#Analysis
#Integral
#Calculus
#Measures
#Mathematics
#Probability
I hope that this helps students, pupils and others. Have fun!
(This explanation fits to lectures for students in their first and second year of study: Mathematics for physicists, Mathematics for the natural science, Mathematics for engineers and so on)
Measure Theory 15 | Image measure and substitution rule
Measure Theory 15 | Image measure and substitution rule [dark version]
Measure Theory: Part 15 Image Measure and Substitution Rule
IQ TEST
Notion of lebesgue measure and its basic properties (MAT)
It's a baby girl: Nub Theory: Ultrasound case!!
Maths Ki Problem 😜 PIHOOZZ
How REAL Men Integrate Functions
Proof of Area Formula (geometric measure theory)
Measure Theory for Applied Research (Class.3: Measures & Measure Spaces)
measure theory 2: functions
Lebesgue measurable functions and basic properties (MAT)
Pakistan education system what a beautiful environment WOW🤣🤣
Measure Theory, Functional Analysis, and The Lebesgue Integral for Undergraduates - Johnston
Measure Theoretic Probability, Lesson 1
4 hour Sleep 16 hour study 🤯 by AIR-4, IIT Bombay, ISRO, BARC #iitjee #neet #gate #isro
F-3 Batch Farewell Party with Neetu Mam 🤩 | @NeetuSinghEnglish #farewell #kdcampus #shorts
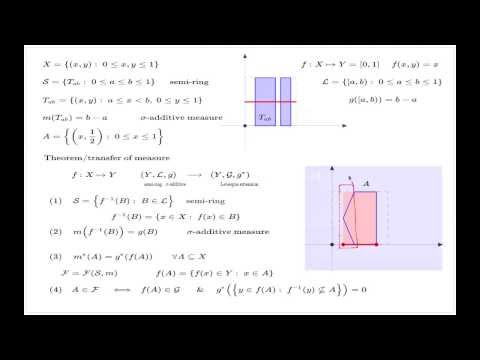
Transfer of Measure
This chapter closes now, for the next one to begin. 🥂✨.#iitbombay #convocation
Lebesgue measurable functions: almost everywhere concept and its implications (MAT)
cardboard art #Shorts
What does measure theory mean?
Measure Theory 17 | Product measure and Cavalieri's principle
Measure Theory 13 | Lebesgue-Stieltjes Measures
Комментарии
 0:11:47
0:11:47
 0:12:02
0:12:02
 0:11:47
0:11:47
 0:00:29
0:00:29
 0:19:10
0:19:10
 0:00:10
0:00:10
 0:00:23
0:00:23
 0:00:35
0:00:35
 0:50:28
0:50:28
 0:12:30
0:12:30
 0:00:31
0:00:31
 0:30:46
0:30:46
 0:00:16
0:00:16
 0:15:46
0:15:46
 0:22:29
0:22:29
 0:00:59
0:00:59
 0:00:14
0:00:14
 0:09:24
0:09:24
 0:00:16
0:00:16
 0:25:10
0:25:10
 0:00:03
0:00:03
 0:00:41
0:00:41
 0:10:28
0:10:28
 0:13:55
0:13:55