Applied topology 2: Topology and homotopy equivalences

Applied topology 2: Topology and homotopy equivalences

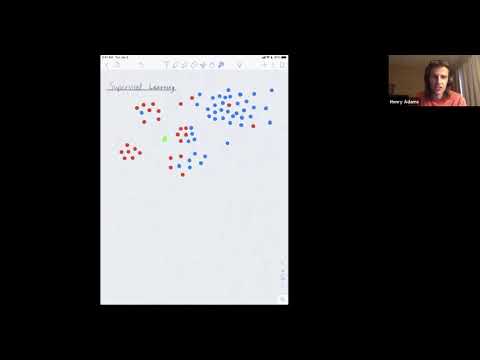
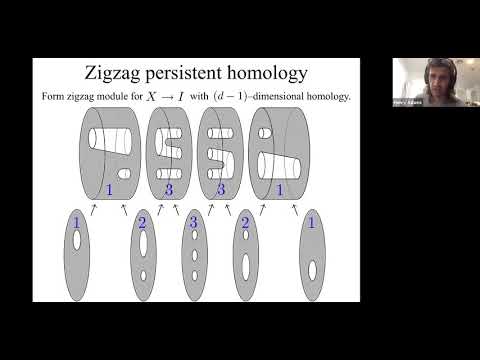
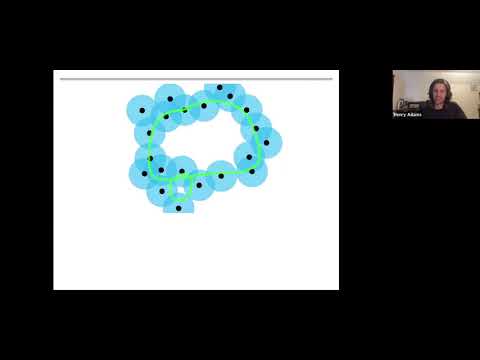
Abstract: From a high-level perspective, we try to explain what topology measures and ignores about shapes. We explain how a coffee cup is in some sense the same shape as a donut. Topologists often deem two shapes to be the same if they are "homotopy equivalent" to each other. We give several examples of shapes that are homotopy equivalent to each other, and several examples of shapes that are not homotopy equivalent to each other.

This video accompanies the class "Topological Data Analysis" at Colorado State University:

[HelloWorlds__JTS]

Thanks for making these simplified tutorial videos, Henry! I do have one issue that keeps popping up no matter how many of these sorts of videos, or short descriptions in print, I have found. And it is directly related to the last question - which is why I'm asking this here rather than on one of your videos on homology:

Why is it that a path that starts at a point on, e.g. a torus, which goes around the hole and returns to the same point - so that it's fair to say this path has been "glued" closed, resulting in a nonconstrictable loop - can then be unglued by continuing the path in the opposite direction around the hole along any other path that returns it to the point? I think the latter describes the fundamental group. In this case the supposed gluing did nothing to cause the nonconstrictable loop around the hole to remain intact, whereas the answer you gave in this video seems to imply that this ungluing is not allowed.

It seems to have something to do with the various separation axioms and Hausdorffness of the topological space, such that T2 is used in one case but not the other. In other words, it seems to have something to do with what it means to be the same point, or at least points that aren't separated, differing by the choice of separation Ti.

At what point in the progression between the two genuses in your answer to the question in the video, or by what rule explaining gluing, does the gluing become irreversible, or at least cause the change in classification?

Can you correct, clarify, and close this for me, once and for all?

[blurp]

How come you're not allowed to glue or break the shapes but you're allowed to puncture them?

[mohamedradwan]

How about the difference between homotopy and homeomorphism?

[vijaybikramsubedi]

How is the 6th shape in lower row (hollow torus with puncture which is just above the coffee mug) homotopy equivalent to its immediate left neighbour?
I'm just not being able to picture it mentally as others.