filmov
tv
Applied topology 7: How do you recover the shape of a dataset?
Показать описание
Applied topology 7: How do you recover the shape of a dataset?
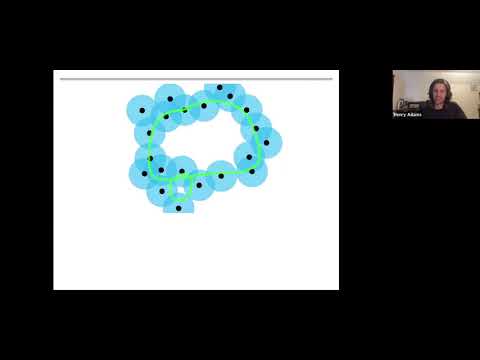
Abstract: Given a point cloud dataset, we explain how you can "blur your vision" in order to try to recover the underlying shape from which that dataset might have been sampled. We blur our vision by growing balls around each data point, and then considering the union of the balls. It is possible to also represent these thickenings via simplicial complexes (vertices, edges, triangles, tetrahedra, etc), including for example the Cech or Vietoris-Rips simplicial complex. This video is a preview to persistent homology, which we will discuss next!
This video accompanies the class "Topological Data Analysis" at Colorado State University:
Abstract: Given a point cloud dataset, we explain how you can "blur your vision" in order to try to recover the underlying shape from which that dataset might have been sampled. We blur our vision by growing balls around each data point, and then considering the union of the balls. It is possible to also represent these thickenings via simplicial complexes (vertices, edges, triangles, tetrahedra, etc), including for example the Cech or Vietoris-Rips simplicial complex. This video is a preview to persistent homology, which we will discuss next!
This video accompanies the class "Topological Data Analysis" at Colorado State University:
0:07:10
Applied topology 7: How do you recover the shape of a dataset?
0:03:54
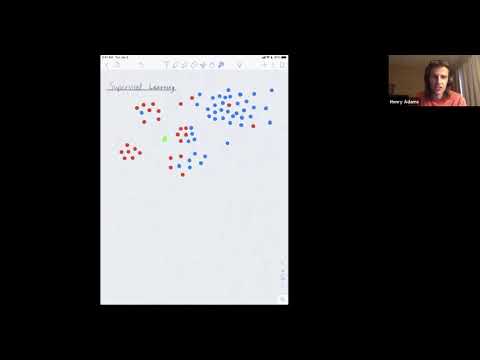
Applied topology 10: Unsupervised vs supervised learning
0:10:35
Applied topology 23: Paper Introduction: Coordinate-free coverage in sensor networks
0:08:47
Applied topology 19: Linear dimensionality reduction - Principal Component Analysis (PCA), Part I
0:22:07
Henry Adams - Bridging applied and geometric topology
0:13:43
An Introduction to Directed Topology [Robin Belton]
0:14:47
Applied topology 1: Datasets have shape
0:09:40
Applied topology 6: Homology
0:13:19
Topology is Impossible Without These 7 Things
0:07:32
Applied topology 17: Persistence and local geometry, Part A
0:11:11
You Could Have Invented Homology, Part 1: Topology | Boarbarktree
0:09:23
Applied topology 4: An introduction to the torus and Klein bottle
0:09:26
Applied topology 12: Hierarchical clustering and single-linkage clustering
1:28:53
Algebraic Topology - Lecture 7 - The Universal Covering
0:39:34
Fixed points in digital topology
0:07:04
Applied topology 18: Persistence and local geometry, Part B
0:14:36
Topology For Beginners | Topology for Msc Maths | Topology | What is topology | What is manifold
1:17:30
Persistence and Triangulation in Lagrangian Topology - Paul Biran
0:10:55
Applied topology 11: Clustering and K-means clustering
0:07:07
Applied topology 3: A punctured torus is homotopy equivalent to a figure eight
0:57:48
Topological and combinatorial methods in Theoretical Distributed Computing - Feichtner Kozlov
0:23:32
Topological Data Analysis of Plant-Pollinator Resource Complexes [Melinda Kleczynski]
0:45:19
Chad Giusti: Topology convexity and neural networks
0:59:34
Mason Porter (7/21/2021): Topological Data Analysis of Spatial Complex Systems
Комментарии