filmov
tv
Solving 5(1-5x^2)^2=1-x

Показать описание
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #PolynomialEquations
via @YouTube @Apple @Desmos @NotabilityApp
@googledocs @canva
PLAYLISTS 🎵 :
#ChallengingMathProblems #PolynomialEquations
via @YouTube @Apple @Desmos @NotabilityApp
@googledocs @canva
PLAYLISTS 🎵 :
Solving 5(1-5x^2)^2=1-x
How To Find x To Form Consecutive Arithmetic Sequence x +3, 2x +1, 5x +2
Solve quadratic equation by factorisation
Solve for x: 5/2 x^2+2/5=1-2x (CBSE 2022)
Learn how to add two mixed numbers with unlike denominators
Solving the Exponential Equation 5^(2x + 1) = 25
Expanding Brackets
Simplify
Calculus 2 Exam Review Part6
Strategies to Solve Multi Step Linear Equations with Fractions - EDEXCEL - GCSE - SAT
Algebra: FOIL Method #Shorts #algebra #math #maths #mathematics #education #learn
🤔How to simplify algebraic expressions??? Algebraic Expressions/Short Tricks #shorts #shortsfeed
5^x+1+5^2-x=5^3+1 How to solve for x??
Solving an equation with variables on both side and one solution
Solve 2^(x+1)=5^(1-2x)
how to setup partial fractions (all cases)
When mathematicians get bored (ep1)
Partial Fractions - Denominator with quadratic factors | SHS 1 ELECTIVE MATH
Learn how to evaluate for a function
A.I. Solve 100 x 100 Rubik’s Cube
How REAL Men Integrate Functions
This trick can make your rubik's cube 2x faster😱🔥#ytshorts#shorts#drcuber
solve 2(x+1)+3(x-1)=5, 20 seconds
Solve the equation
Комментарии
 0:08:58
0:08:58
 0:02:44
0:02:44
 0:09:05
0:09:05
 0:05:11
0:05:11
 0:04:18
0:04:18
 0:01:02
0:01:02
 0:00:51
0:00:51
 0:05:21
0:05:21
 5:47:36
5:47:36
 0:15:41
0:15:41
 0:00:18
0:00:18
 0:00:16
0:00:16
 0:03:29
0:03:29
 0:02:36
0:02:36
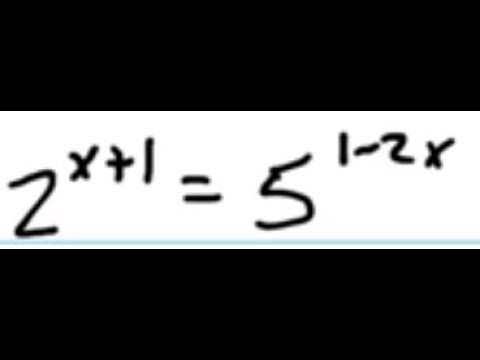 0:04:49
0:04:49
 0:09:08
0:09:08
 0:00:37
0:00:37
 0:08:20
0:08:20
 0:02:15
0:02:15
 0:00:16
0:00:16
 0:00:35
0:00:35
 0:00:16
0:00:16
 0:00:24
0:00:24
 0:01:53
0:01:53