Finding Equivalent Fractions and Simplifying Fractions

Fractions: This video will explain finding equivalent fractions and simplifying fractions. Practice questions are provided at the end of the video.

Skip to:

00:00 Introduction
00:23 What is a fraction?
00:43 What is a denominator?
00:55 What is a numerator?
01:11 Equivalent fractions explained
03:24 How to simplify fractions
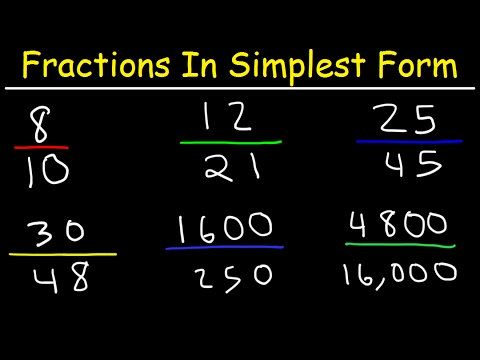
04:25 Practice questions: write the fractions in the simplest form

Below are some links to products we recommend:

An excellent book if you're teaching mathematics or helping your children:

Educational maths help videos to learn mathematics for school, exams or just general life. New videos added weekly.

LIKE, SUBSCRIBE & COMMENT

Follow us:

#fractions #equivalentfractions #simplifyingfractions #maths #learnmath #mathhelp #mathsteachingresources #GCSE

[MinityMaths]

Thank you for watching! Subscribe to our channel for more videos like this!

[iqfncmq]

HOW TO SIMPLIFY FRACTIONS THE EASIEST WAY:
If the difference between the numerator and denominator is 1, the fraction cannot be simplified.
A. Ask: does the numerator go into the denominator evenly (i.e. without any remainder)?
If yes, proceed to B.
If not, proceed to C.
B. Great! The simplest fraction is 1 over the number of times the numerator fits in the denominator. (e.g. 20/60, 20 fits evenly 3 times, hence 1/3.)
C. Write the remainder, (e.g. 20/46, (20 fits 2 times in 46, with a remainder of) 6.) to the right of the fraction, and ask: does the remainder go evenly into the numerator?
If yes, proceed to D.
If not, proceed to E.
D. Great! The "remainder" is the Greatest Common Factor. Divide both the numerator and denominator by the GCF/remainder to have the simplest fraction.
E. Write the "new remainder" (using the last example, 20/46, 6 fits into 20 with a "new remainder" of 2) to the right of the previous "remainder", and ask: does the "new remainder" fit evenly into the previous "remainder" (2 into 6)?
If yes, proceed to D.
If not, proceed to E.
Please, (with your amazing talent, ) produce a video demonstrating it.

//Euclidean Algorithm.