filmov
tv
Find the product of (5x+7)(3x+4) | algebra | Basic Mathematics

Показать описание
#algebra #basicmathematics #sammaiah #produtofbinomial #freeclasses #freeclass #s
Find the product of (5x+7)(3x+4)
(5x+7)(3x+4)
=5x(3x+4)+7(3x+4)
=15x²+20x+21x+28
=15x²+41x+28
Find the product of (5x-7)(3x+4)
(5x-7)(3x+4)
=5x(3x+4)-7(3x+4)
=15x²+20x-21x-28
=15x²-x-28
Find the product of (5x+7)(3x-4)
(5x+7)(3x-4)
=5x(3x-4)+7(3x-4)
=15x²-20x+21x-28
=15x²+x-28
Find the product of (5x-7)(3x-4)
(5x-7)(3x-4)
=5x(3x-4)-7(3x-4)
=15x²-20x-21x+28
=15x²-41x+28
THANK YOU
Find the product of (5x+7)(3x+4)
(5x+7)(3x+4)
=5x(3x+4)+7(3x+4)
=15x²+20x+21x+28
=15x²+41x+28
Find the product of (5x-7)(3x+4)
(5x-7)(3x+4)
=5x(3x+4)-7(3x+4)
=15x²+20x-21x-28
=15x²-x-28
Find the product of (5x+7)(3x-4)
(5x+7)(3x-4)
=5x(3x-4)+7(3x-4)
=15x²-20x+21x-28
=15x²+x-28
Find the product of (5x-7)(3x-4)
(5x-7)(3x-4)
=5x(3x-4)-7(3x-4)
=15x²-20x-21x+28
=15x²-41x+28
THANK YOU
Find the product of (5x+7)(3x+4) | algebra | Basic Mathematics
Find each the following products:(5x + 7) × (3x + 4)
Find the product of (5x + 7) × (3x + 4 ) ?
(x+3)(x+5) Expand and Simplify
5 simple unsolvable equations
Solving an equation with variables on both side and one solution
Multiply (5x+2)(3x-4)
Expand & Simplify: (2x - 3)(3x - 4)
Find the product of (5x+2)(3x+4) | Basic Mathematics | competitive exams special
Multiply (2x^2+3x-7) and (3x^2-5x+4) | Product of two algebraic expressions | Class 8th Maths
Find the Product (3x+5)(3x-6)
Solving an equation for y and x
Find the product of (-2x^5)(-3x^4)(5x^3)
Taking the derivative of two binomials using product and chain rule
How to expand a binomial raised to the 3 power
(3x+5) / (4x+2) = (3x+4) / (4x+7) find the value of x ?
Composite Functions
Solving Rational Equations
Resolver ecuaciones de primer grado: a) 5x + 6 = 10x + 5
Find the derivative of y = 3x^2 - 5x - 7
Find the Product 3x-4
Solving congruences, 3 introductory examples
Find the Product (3x+3)(x+4)
Solve by completing the square | Step by Step Technique
Комментарии
 0:02:11
0:02:11
 0:02:09
0:02:09
 0:00:53
0:00:53
 0:00:44
0:00:44
 0:00:50
0:00:50
 0:02:36
0:02:36
 0:01:42
0:01:42
 0:01:15
0:01:15
 0:02:11
0:02:11
 0:03:13
0:03:13
 0:02:03
0:02:03
 0:03:47
0:03:47
 0:00:40
0:00:40
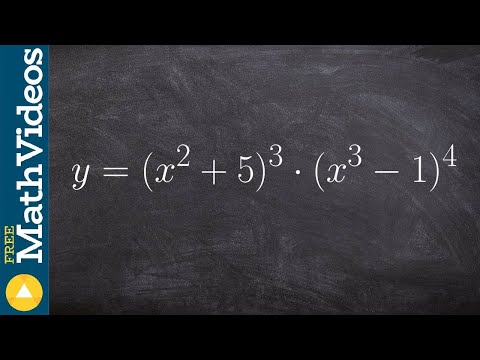 0:04:44
0:04:44
 0:02:53
0:02:53
 0:03:25
0:03:25
 0:05:23
0:05:23
 0:11:03
0:11:03
 0:05:51
0:05:51
 0:00:43
0:00:43
 0:01:28
0:01:28
 0:03:51
0:03:51
 0:01:31
0:01:31
 0:05:20
0:05:20