filmov
tv
JEE Delight | Calculus | JEE Advanced 2023 | Paper1 | Q4 | Limits | Sandwich theorem
Показать описание
JEE Delight | Calculus | JEE Advanced 2023 | P1 Q4 | Limits | Sandwich theorem
Let f : (0, 1) −→ IR be the function defined as f(x) = √n
where n ∈ N. Let g : (0, 1) → R be a function such that
x for all x ∈ (0, 1). Then limx→0
f(x)g(x)
(A) does NOT exist
(B) is equal to 1
(C) is equal to 2
(D) is equal to 3
Support the channel:
Let f : (0, 1) −→ IR be the function defined as f(x) = √n
where n ∈ N. Let g : (0, 1) → R be a function such that
x for all x ∈ (0, 1). Then limx→0
f(x)g(x)
(A) does NOT exist
(B) is equal to 1
(C) is equal to 2
(D) is equal to 3
Support the channel:
0:04:41
0:06:56
0:05:52
0:12:03
0:04:55
0:04:46
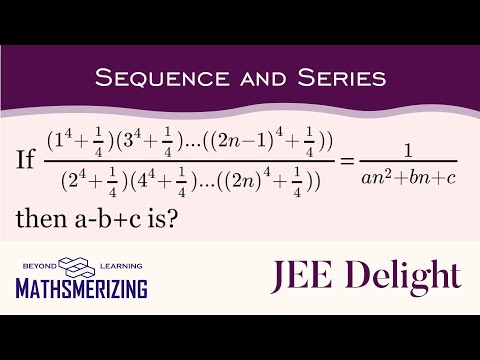0:06:55
0:03:09
0:04:36
0:06:48
0:16:08
0:11:16
0:05:18
0:14:46
0:05:09
0:10:05
0:08:46
0:07:39
0:10:58
0:02:25
1:07:03
0:05:14
0:03:57
0:12:48