filmov
tv
JEE Delight | Sequence & Series | Sophie Germain Identity | A rare telescoping product problem
Показать описание
JEE Delight | Sequence & Series | Sophie Germain Identity | An elegant solution to a rare telescoping product problem.
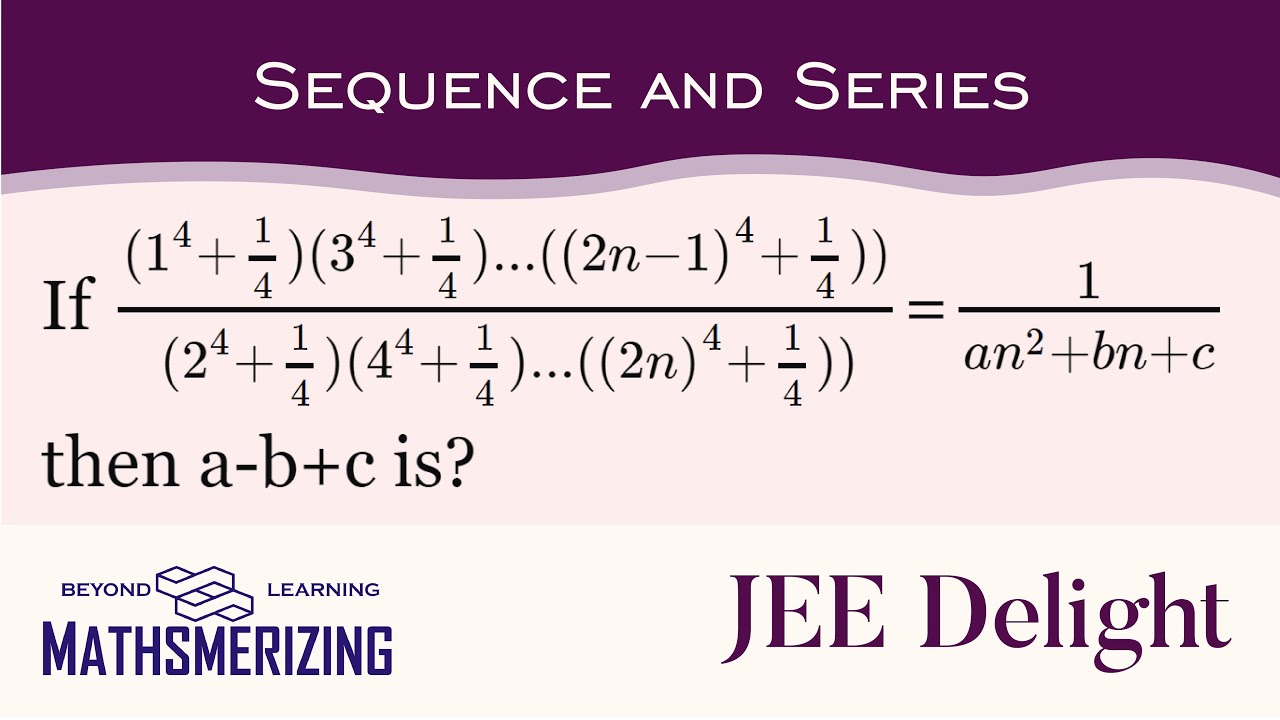
Solve 1^4+1/4)(3^4+1/4)...((2n-1)^4+1/4))/(2^4+1/4)(4^4+1/4)...((2n)^4+1/4))
Unravel the secrets of a challenging JEE Advanced math problem with our succinct and insightful solution. If you thought higher algebra was daunting, think again! Our video breaks down a complex sequence problem, showing you a step-by-step approach to finding the elusive values of a, b, and c. Perfect for JEE aspirants looking to master their problem-solving skills and gain that competitive edge. Watch, learn, and conquer your math fears as you prepare for one of the most pivotal exams of your life. Join us on this mathematical adventure and stay ahead of the curve with more tips, tricks, and solutions!
Support the channel:
Solve 1^4+1/4)(3^4+1/4)...((2n-1)^4+1/4))/(2^4+1/4)(4^4+1/4)...((2n)^4+1/4))
Unravel the secrets of a challenging JEE Advanced math problem with our succinct and insightful solution. If you thought higher algebra was daunting, think again! Our video breaks down a complex sequence problem, showing you a step-by-step approach to finding the elusive values of a, b, and c. Perfect for JEE aspirants looking to master their problem-solving skills and gain that competitive edge. Watch, learn, and conquer your math fears as you prepare for one of the most pivotal exams of your life. Join us on this mathematical adventure and stay ahead of the curve with more tips, tricks, and solutions!
Support the channel:
JEE Delight | Sequence & Series | Sophie Germain Identity | A rare telescoping product problem
JEE Delight | Sequences (Real Analysis) | Squeeze play theorem | lim x^100ln(x)/e^xarctan(pi/3+sinx)
JEE Delight | 17 must know models of Telescopic summation | Method of difference
JEE Delight | Sequences (Real Analysis) | Lecture 12 | Cauchy's second theorem on limits | #2 S...
JEE Delight | JEE Advanced 2023 | Paper1 | Q10 | Sequence and series
JEE Delight | Sequences (Real Analysis) | ASE #3 | an=n(an-1+1) and Pn=(1+1/a1)(1+1/a2)...(1+1/an)
JEE Delight | Simple yet elegant | max [r1+r2] for two touching circles inscribed in a semi-circle
JEE Delight | B Stat/ B Math | ISI | UGB 2023 | Q6 | Series | Telescopic summation
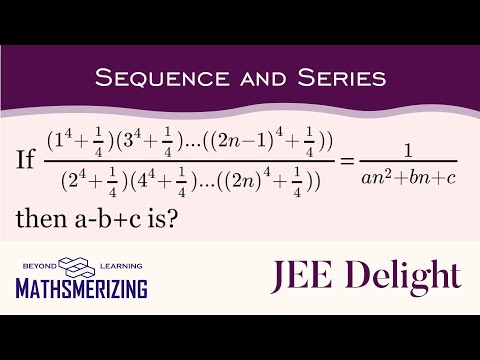
JEE Delight | 3 D geometry & Determinants | Passage | Three planes intersect in a line
JEE Delight: IPMAT Q18: In the sequence 12345678910111213.. find 6389th digit?
JEE Delight | Differentiability of parametric functions | Solved example with two methods
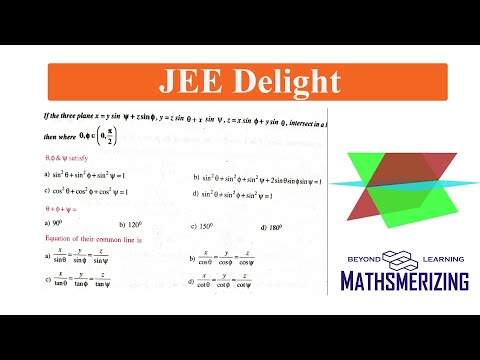
JEE Delight | Sequences (Real Analysis) | Lecture 11 | Cauchy's first theorem on limits | #3 SE
JEE Delight | Circles | A circle trapped in a trapezoid | Matrix matching
JEE Delight: Binomial series reversing technique: find summation nCksinkxcos(n-k)x
Amazing JEE Delight | P&C | CMI 2022 | A8 | JEE Delight | Chennai mathematical institute
JEE Delight: Sum of all coefficients in the expansion of (a+b+c+d)^8 in terms containing b but not c
JEE delight: If (1+2^1/2)=xn+yn, find xn+1 and yn+1
JEE delight: Number of functions such that for every x there is exactly one y such that f(x)=f(y)
JEE Delight | Leibniz Integral Rule | Max/Min of an integral function
JEE Advanced 2022 | Paper 1 | Q6 | Mathematics | Sequence & series
JEE Delight | 3 amazing applications of simple Triangle inequality | Algebra | Probability | P&C
Limits | An amazing JEE Delight PYQ | Telescopic summation of trigonometric series and limits
JEE Delight | ISI UGA 2024 | Q14 | Limit of probability of atmost one handshake in a group of n
JEE Delight | Geometry of complex number | Circles | SE#12 | Triangle & square | Complex functio...
Комментарии
 0:06:55
0:06:55
 0:03:15
0:03:15
 1:07:03
1:07:03
 0:04:03
0:04:03
 0:05:14
0:05:14
 0:03:29
0:03:29
 0:12:03
0:12:03
 0:04:39
0:04:39
 0:08:28
0:08:28
 0:03:20
0:03:20
 0:11:16
0:11:16
 0:04:56
0:04:56
 0:07:39
0:07:39
 0:02:47
0:02:47
 0:04:36
0:04:36
 0:02:53
0:02:53
 0:04:12
0:04:12
 0:02:12
0:02:12
 0:05:09
0:05:09
 0:05:02
0:05:02
 0:16:41
0:16:41
 0:09:17
0:09:17
 0:04:36
0:04:36
 0:03:41
0:03:41