filmov
tv
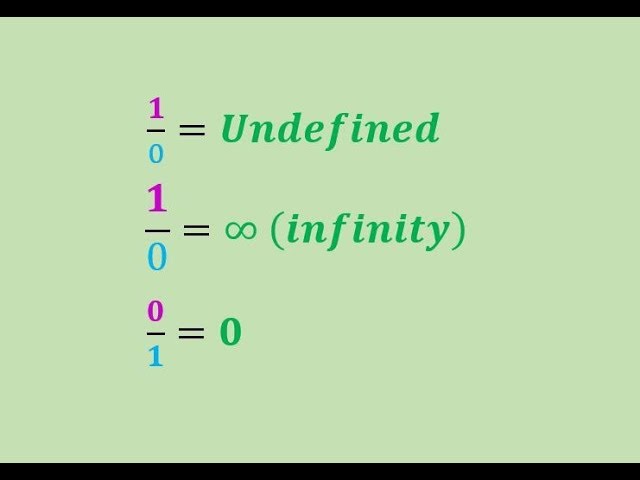
1/0 = Undefined or Infinity: Easy proof to understand with a real world example.
Показать описание
1/0 = Undefined or Infinity: Easy proof to understand with a real world example.
1/0 = undefined or infinity
Why can't you divide by zero? - TED-Ed
1/0 = Undefined or Infinity: Easy proof to understand with a real world example
Does 1/0 = Infinity? #shorts
1/0 = ? . Don’t get confused.
What is Zero Times Infinity?
1 divided by 0 is equal to || One by zero | Division by Zero
1 ^ ∞, It's Not What You Think
Dividing by zero?
Problems with Zero - Numberphile
Approaching but not Reaching Infinity for 1/0: Dividing by Zero
Any number divided by 0 is undefined or infinity. That's why!!!
I Finally Found Out What 0/0 Should Be
Any Number Divided by Zero is UNDEFINED or INFINITY (in Hindi)
Why zero divided by zero is undefined/indeterminate | Algebra II | Khan Academy
0 x ♾️ , It's Not What You Think
Difference Between Infinity and Undefined | Maths Class 11
The Most Controversial Number in Math
Why Can't We DIVIDE by ZERO? (1÷0) | Infinity OR Undefined?
The Mind-Blowing Truth About 1/0: Undefined vs Infinity!🤔😮
Simple proof why 1/0 is infinity?
How To Count Past Infinity
Absolute infinity Minus One?!?
Комментарии
 0:03:41
0:03:41
 0:00:31
0:00:31
 0:04:51
0:04:51
 0:04:14
0:04:14
 0:00:57
0:00:57
 0:06:16
0:06:16
 0:00:32
0:00:32
 0:00:49
0:00:49
 0:04:28
0:04:28
 0:09:09
0:09:09
 0:13:00
0:13:00
 0:01:00
0:01:00
 0:02:51
0:02:51
 0:04:40
0:04:40
 0:18:48
0:18:48
 0:02:26
0:02:26
 0:05:07
0:05:07
 0:22:58
0:22:58
 0:06:46
0:06:46
 0:04:50
0:04:50
 0:04:31
0:04:31
 0:01:30
0:01:30
 0:23:46
0:23:46
 0:00:21
0:00:21