filmov
tv
A visual alternating sum!
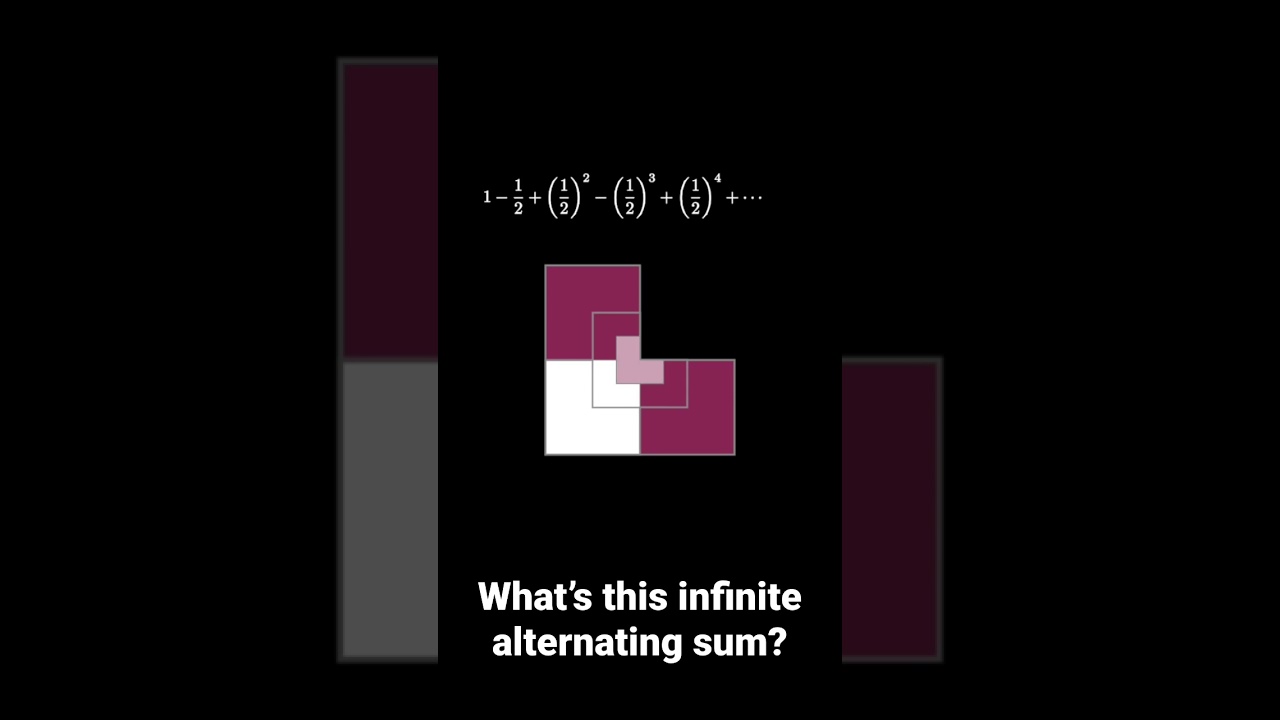
Показать описание
This is a short, animated visual proof demonstrating the sum of the infinite geometric series with ratio -1/2.
#mathshorts #mathvideo #math #calculus #mtbos #manim #animation #theorem #pww #proofwithoutwords #visualproof #proof #iteachmath #geometricseries #infiniteseries #fractals #sum #induction
To learn more about animating with manim, check out:
#mathshorts #mathvideo #math #calculus #mtbos #manim #animation #theorem #pww #proofwithoutwords #visualproof #proof #iteachmath #geometricseries #infiniteseries #fractals #sum #induction
To learn more about animating with manim, check out:
A visual alternating sum!
Alternating Sum of Triangular Numbers (visual proof without words)
Halloween Visual Proof: Alternating Sum of Odds #math
Geometric Series: Alternating Sum of Powers of 1/4 (visual proof)
Alternating Sum of Squares II (visual proof without words)
Geometry of the Alternating Harmonic Series (visual proof)
Alternating infinite sum!
Alternating Series (-1/2)^n #1 || Visual Proof
Alternating Geometric Series: sum of Powers of 1/2 (visual proof)
Alternating Sum Of Squares (visual proof)
Alternating 1/4 Sum
Alternating Geometric Series Sum
Alternating Geometric Series: Powers of -1/3 (visual proof)
Alternating sum of squares is triangular!
Alternating series #2 | Visual solution |
Visual Proof Short: Alternating Sum of Squares II
Alternating Geometric Series: sum of powers of 1/2 II (visual proof)
Visualizing the Alternating Series Estimation Theorem
Squares from alternating triangles (visual proof without words)
How to find the sum of almost any rearrangement of the alternating harmonic series.
Easy Proof Of Summation of Alternating Harmonic Series
Alternating Geometric Series (visual proof)
Alternating series #1 | Visual solution |
2023 Number Challenge: Estimate the Alternating Harmonic Series
Комментарии
 0:00:51
0:00:51
 0:01:17
0:01:17
 0:02:01
0:02:01
 0:01:26
0:01:26
 0:01:05
0:01:05
 0:04:20
0:04:20
 0:00:52
0:00:52
 0:01:02
0:01:02
 0:01:28
0:01:28
 0:02:02
0:02:02
 0:00:57
0:00:57
 0:01:00
0:01:00
 0:01:43
0:01:43
 0:00:44
0:00:44
 0:03:08
0:03:08
 0:00:52
0:00:52
 0:01:58
0:01:58
 0:06:41
0:06:41
 0:01:39
0:01:39
 0:00:50
0:00:50
 0:19:28
0:19:28
 0:02:06
0:02:06
 0:01:37
0:01:37
 0:04:45
0:04:45